 |
PBT501S - PROBABILITY THEORY 1 - 1ST OPP - JUNE 2022 |
 |
1 Page 1 |
▲back to top |

NAMIBIA UNIVERSITY
OF SCIENCE AND TECHNOLOGY
FACULTY OF HEALTH, APPLIED SCIENCES, AND NATURAL
RESOURCES
DEPARTMENT OF MATHEMATICS AND STATISTICS
QUALIFICATION : BACHELOR OF SCIENCE APPLIED MATHEMATICS AND STATISTICS
QUALIFICATION CODE: 07BAMS
LEVEL: 5
COURSE: PROBABILITY THEORY 1
COURSE CODE: PBT501S
DATE: JUNE 2022
SESSION: JUNE
DURATION: 3 HOURS
MARKS: 100
EXAMINER(S)
FIRST OPPORTUNITY EXAMINATION QUESTION PAPER
| Dr. D. Ntirampeba
Mr. E. Mwahi
moperator:; | Mr. A. Roux
THIS QUESTION PAPER CONSISTS OF 3 PAGES
(Excluding this front page and statistical tables)
INSTRUCTIONS
Answer ALL the questions.
Write clearly and neatly.
Number the answers clearly.
PERMISSIBLE MATERIALS
1. Non-programable calculator
ATTACHMENTS
1. Statistical tables (Z-Tables)
 |
2 Page 2 |
▲back to top |
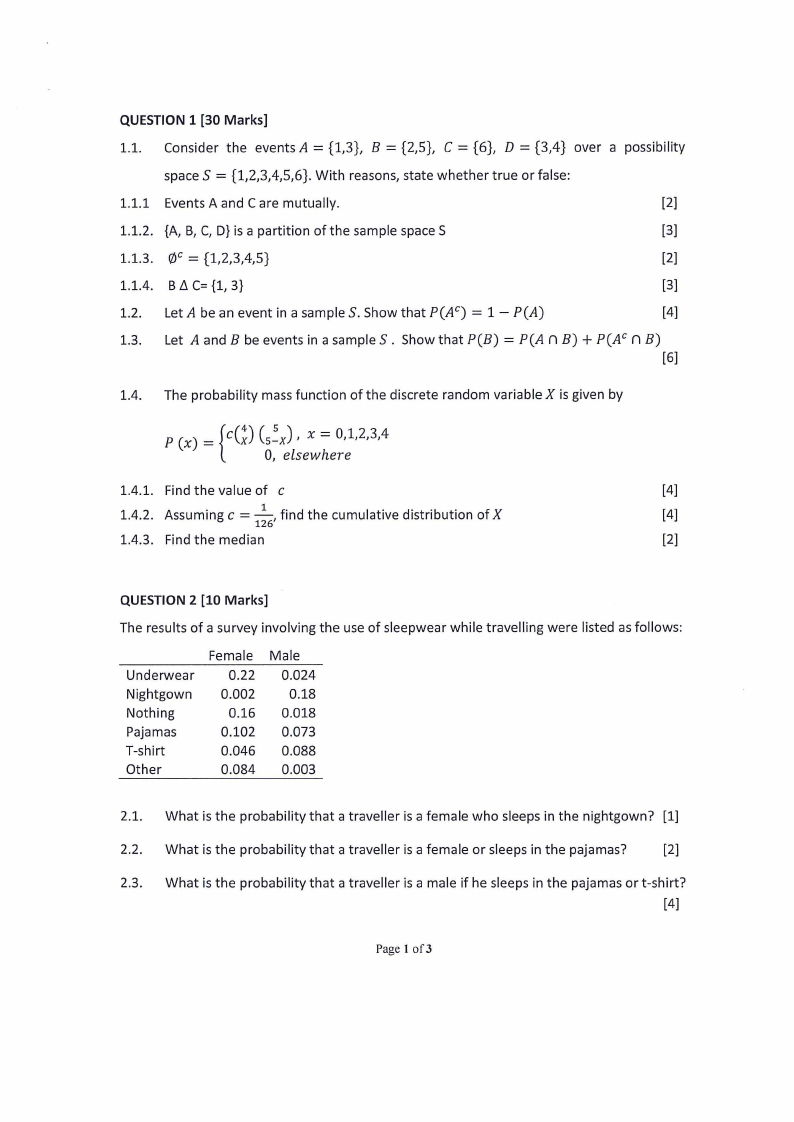
QUESTION 1 [30 Marks]
1.1. Consider the eventsA = {1,3}, B = {2,5}, C = {6}, D = {3,4} over a possibility
space S = {1,2,3,4,5,6}. With reasons, state whether true or false:
1.1.1 Events A and C are mutually.
[2]
1.1.2. {A, B, C, D}is a partition of the sample space S
[3]
1.1.3. O° = {1,2,3,4,5}
[2]
1.1.4. BAC={1, 3}
[3]
1.2. Let A be an event in a sample S. Show that P(A°) = 1 — P(A)
[4]
1.3. Let AandB be events in asample S. Show that P(B) = P(AN B) + P(A NB)
[6]
1.4. The probability mass function of the discrete random variable X is given by
P(x) = {e 4\\ (.°(5y)» x _= 0,1,2,3,4
0, elsewhere
1.4.1. Find the value of c
[4]
1.4.2. Assuming c = or find the cumulative distribution of X
[4]
1.4.3. Find the median
[2]
QUESTION 2 [10 Marks]
The results of a survey involving the use of sleepwear while travelling were listed as follows:
Underwear
Nightgown
Nothing
Pajamas
T-shirt
Other
Female
0.22
0.002
0.16
0.102
0.046
0.084
Male
0.024
0.18
0.018
0.073
0.088
0.003
2.1. What is the probability that a traveller is a female who sleeps in the nightgown? [1]
2.2... What is the probability that a traveller is a female or sleeps in the pajamas?
[2]
2.3. What is the probability that a traveller is a male if he sleeps in the pajamas or t-shirt?
[4]
Page 1 of3
 |
3 Page 3 |
▲back to top |

2.4. Assuming a traveller is male, what is the probability that he sleeps pajamas?
[3]
QUESTION 3 [15 Marks]
3.1. Police plan to reinforce speed limits by using a radar traps at 4 different locations
with the city of Windhoek. The radar traps at each of the locations L1, L2, L3, and L4
are operated 40%, 30%, 20%, and 30% of the time, and if a person who is speeding
on his way to has a probabilities of 0.2, 0.1, 0.5, and 0.2, respectively, of passing
through these locations, what is the probability that he will receive a speeding
ticket?
[5]
Further, if the person received a speeding ticket on his way to work, what is the
probability that he passed through the radar trap at L2?
[5]
3.2 A diagnostic test for cancer is said to be 98% accurate if a person has the disease.
Also, if a person does not have cancer, the test will report that he or she does not
have it with probability 0.1. Only 0.1% has the disease in question. If a person is
chosen at random from the population and diagnostic test indicates that he or she
has cancer, what is the probability that he or she does, in fact, have cancer.
[5]
QUESTION 4[20 Marks]
4.1. A large industrial firm purchase several new word-processors at the end of each
year, the exact number depending on the frequency of repairs in the previous year.
Suppose that the number of wordprocessors, X, that are purchased each year has
the following probability distribution:
x|
0
1
2
3
p(x) | O12 0.3
0.4
0.2
If the cost on new wordprocessors at the end of this year is given by 12000 — 50X?, in
Namibia Dollars,
4.1.1. how much can this firm expect to spend on new wordprocessors at the end of this
year?
[6]
4.1.2. find the variance of the number of wordprocessors that are purchased for this firm
at the end of this year.
[7]
Page 2 of3
 |
4 Page 4 |
▲back to top |

4.1.3. find the coefficient of variation for the number of wordprocessors that are
purchased for this firm at the end of this year.
[4]
4.2. A random variable X has a mean = 10 and a variance o? = 4. Use Chebyshev’s
theorem find
P(S<X <15)
[3]
QUESTION 5[25 Marks]
5.1. LetX be a binomial random with a probability mass function given by
fF) =
n
() p*q"™, for x = 0,1,..,n
0,
elsewhere
Show that "9 f(x) =1.
[5]
5.2. A multiple choice test consists of five questions with each question having four
alternative answers, only one of which is correct. To pass you need at least four
right answers. What is the probability that you pass?
[4]
5.3. | Accrotime is a manufacturer of quartz crystal watches. Accrotime researchers have
shown that the watches have an average life of 28 months before certain electronic
5.3.1.
components deteriorate, causing the watch to become unreliable. The standard
deviation of watch lifetimes is 5 months, and the distribution of lifetimes is normal.
If Accrotime guarantees a full refund on any defective watch for 2 years after
purchase, what percentage of total production should the company expect to
replace?
[4]
5.3.2. If Accrotime does not want to make refunds on more than 12% of the watches it
makes, how long should the guarantee period be (to the nearest month)?
[5]
5.4. Asecretary makes 2 errors per page, on average.
5.4.1. What is the probability that on the page he or she will make 4 or more errors? [5]
5.4.2. What is the expected number of errors in the next three pages?
[2]
END OF QUESTION PAPER
Page 3 of3
 |
5 Page 5 |
▲back to top |
Standard Normal Probabilities
Table entry
:
Zz
:00
-3.4 .0003
3.3. .0005
-3.2
.0007
=3.1 - 0010
-3.0 .0013
=2-9 = 0019
-2.8
.0026
e207 0035
-2.6 .0047
2.5 .0062
2.4 .0082
2.3, 0107
-2.2 .0139
=2,15 2 0179
-2.0 .0228
=L.9% 0287
—1.8 .0359
=l.7 ..0446
—1.6 .0548
-1.5 .0668
-1.4 .0808
-1.3 .0968
—1.2
.1151
sla 2 1357
-1.0 .1587
=0:9
.1841
-0.8
.2119
—0-7 © .2420
0.6 .2743
-0.5
.3085
-0.4 .3446
=0.3° 2.3821
-0.2
.4207
=0.1. ..4602
-0.0 .5000
-O1
:02
.0003 = .0003
0005 =.0005
0007 + .0006
.0009 = .0009
.0013 =.0013
.0018 .0018
0025 .0024
0034 ~=.0033
0045 .0044
0060 § .0059
.0080 .0078
0104 =.0102
.0136 = =.0132
0174 ~=—-.0170
0222 .0217
0281 .0274
0351 .0344
.0436 =.0427
0537 = .0526
.0655 ~=.0643
.0793 = .0778
0951 =.0934
1131 = 1112
1335... 1314
1562 = =.1539
1814 .1788
.2090 =.2061
2389... .2358
.2/09 ~=.2676
3050 =.3015
3409 = .3372
3783 3745
.4168
.4129
4562 = 4522
.4960 4920
Table entry for z is the area under the standard normal curve
to the left of z.
.03
.0003
.0004
.0006
.0009
.0012
.0017
.0023
.0032
.0043
.0057
.0075
.0099
.0129
.0166
.0212
.0268
.0336
.0418
.0516
.0630
.0764
.0918
.1093
1292
1515
1762
.2033
.2327
.2643
.2981
3336
.3707
.4090
.4483
.4880
.04
.0003
.0004
.0006
.0008
.0012
0016
.0023
.0031
.0041
.0055
.0073
.0096
.0125
.0162
.0207
.0262
.0329
.0409
.0505
.0618
.0749
.0901
.1075
.1271
1492
.1736
.2005
.2296
.2611
2946
3300
.3669
.4052
4443
.4840
:05
.0003
.0004
.0006
.0008
0011
0016
0022
.0030
.0040
0054
.0071
0094
0122)
0158
0202
0256.
0322.
0401)
0495
0606
0735
.0885
1056
A251.
.1469
1711
1977,
2266
2578
.2912.
3264
3032).
4013)
4404.
4801
-06
:07
.08
= .0003
.0003
.0003
.0004 .0004 .0004
=6©.0006 §8.0005 # .0005
.0008 .0008 .0007
.0011
.0011
.0010
.0015
0015
.0014
=.0021
.0021
.0020
0029
.0028 ~ .0027
.0039 .0038 .0037
= .0052 = .0051
.0049
.0069 .0068 .0066
=©.0091 ~=.0089 = .0087
=©.0119 .0116 .0113
.0154 .0150 8.0146
.0197 .0192 .0188
.0250. 0244. = .0239
= .0314 .0307 #.0301
.0392. =—.0384... :0575
.0485
.0475
.0465
.0594 .0582 .0571
.0721
.0708
.0694
.0869
.0853
.0838
=.1038 = .1020 .1003
.1230:. 1210... 1190
.1446 = .1423
1401
1685-1660.
«.1635
.1949 = .1922——.1894
2236; 2206. 2177.
=.2546 =.2514 ~=—.2483
2877. - 2843. .2810
=.3228)=— 3192) 3156
23594) 23557, 3520
3974 = 3936 ~—— 3897
4364 = 4325. 4286
4761
3.4721
.4681
-09
.0002
.0003
.0005
.0007
.0010
.0014
.0019
.0026
.0036
.0048
.0064
.0084
.0110
.0143
.0183
.0233
.0294
.0367
.0455
.0559
.0681
.0823
.0985
.1170
.1379
.1611
.1867
.2148
.2451
.2776
3121
3483
3859
4247
.4641
 |
6 Page 6 |
▲back to top |
Table entry
Standard Normal Probabilities
Table entry for z is the area under the standard normal curve
to the left of z.
Zz
:00
-O1
.02
-03
.04
:05
-06
:07
.08
.09
0.0
.5000
.5040
.5080
.5120
.5160
5199
5239
3.5279 .5319
5359
0.1
9398
5438
.5478
9917
2007
-9996..= 5636.
5675
.5/714
D/03
0.2
.5793
.5832
5871
.5910
.5948
5987
.6026
.6064
.6103
.6141
0.3
.6179
.6217
.6255
.6293
.6331
6368
.6406 .6443
.6480
.6517
0.4
.6554
.6591
.6628
.6664
-6700
6736
.6772 .6808
.6844
.6879
0.5
.6915
.6950
.6985
7019
.7054
7088: 7123
7157,
.7190
7224
0.6
7257
7291
.7324
.7357
.7389
7422 ~~ -.7454
.7486
7517
7549
0.7
.7580
7611
.7642
./673
.7704
1734
.7164.. 7794
7823
7852
0.8
7881
.7910
.7939
.7967
7995
8023
~=.8051
.8078
.8106
.8133
0.9
.8159
.8186
8212
8238
.8264
8289: .8315
.8340 .8365
.8389
1.0
.8413
8438
.8461
.8485
.8508
8531
=©.8554 ~—s 8577
.8599
.8621
1
.8643
.8665
.8686
.8708
.8729
8749
.8770 .8790
.8810
.8830
1.2
.8849
.8869
.8888
.8907
8925
8944
.8962
.8980
.8997
9015
13
.9032
9049
.9066
.9082
.9099
9115229131
.9147
.9162
9177
1.4
.9192
.9207
9222
.9236
9251
9265
9279
.9292
.9306
.9319
LS
9332
-9345
.9357
.9370
.9382
9394.
9406
.9418 -9429
9441
1.6
9452
.9463
.9474
.9484
9495
9505
.9515
.9525
9535
.9545
7,
99954. 2.9564
.9573
9582
9591
9599
(9608
.9616
9625
.9633
1.8
9641
.9649
.9656
.9664
9671
.9678 .9686 .9693
.9699
.9706
1.9
.9713
.9719
.9726
9732
.9738
9744
.9750
.9756
9761
9767
2.0
9772
.9778
.9783
.9788
.9793
9798
.9803 .9808
.9812
-9817
2.1
9821
.9826
.9830
.9834
.9838
9842
.9846
.9850
-9854
9857
2.2
.9861
.9864
-9868
.9871
.9875
9878
.9881
.9884
-9887
.9890
23
.9893
.9896
-9898
.9901
.9904
9906
.9909 = 9911
9913
.9916
2.4
.9918
.9920
.9922
9925
.9927
9929 = 9931
9932
-9934
.9936
25
.9938
.9940
9941
9943
9945
9946
.9948
.9949
9951
9952
2.6
.9953
9955
.9956
.9957
9959
9960
=.9961 .9962
.9963
.9964
27,
.9965
.9966
-9967
.9968
.9969
9970.-3,9971
.9972
.9973
.9974
2.8
.9974
9975
.9976
.9977
.9977
9978
.9979 .9979
.9980
.9981
2.9
9981
9982
9982
.9983
9984
9984
.9985
.9985
.9986
.9986
3.0
.9987
.9987
.9987
.9988
.9988
9989
.9989
9989
.9990
9990
Sal
.9990
29991
9991
9991
.9992
+9992 (9992 - 9992
9993
.9993
3.2
9993
.9993
.9994
.9994
.9994
9994
.9994 9995
.9995
9995
3:3
.9995
.9995
-9995
.9996
.9996
9996
.9996
.9996
.9996
.9997
3.4
.9997
.9997
.9997
.9997
.9997
9997
.9997
.9997
.9997
.9998





