 |
FIM601S - FINANCIAL MATHEMATICS 2 -1ST OPP - JUNE 2023 |
 |
1 Page 1 |
▲back to top |

n Am I B I A u n IVER s I TY
OF SCI En CE
TECH n OLOGY
FACULTYOF HEALTH,NATURALRESOURCESAND APPLIEDSCIENCES
SCHOOLOF NATURALAND APPLIEDSCIENCES
DEPARTMENTOF MATHEMATICS, STATISTICSAND ACTUARIALSCIENCE
QUALIFICATION:Bachelor of science; Bachelor of sciencein Applied Mathematics and Statistics
QUALIFICATIONCODE: 07BSAMS
LEVEL: 6
COURSECODE: FIM601S
COURSENAME: FINANCIAL MATHEMATICS 2
SESSION:JUNE 2023
PAPER:THEORY
DURATION: 3 HOURS
MARKS: 100
FIRSTOPPORTUNITY EXAMINATION QUESTION PAPER
EXAMINER
DrV. Katoma
Mrs. H.YNkalle
MODERATOR:
Prof. A.S. Eegunjobi
INSTRUCTIONS
• Answer ALL the questions in the booklet provided.
• Show clearly all the steps used in the calculations.
• All written work must be done in blue or black ink and sketches
must be done in pencil.
PERMISSIBLEMATERIALS
• Non-programmable calculator without a cover.
THIS QUESTION PAPERCONSISTSOF 3 PAGES(Including this front page)
1
 |
2 Page 2 |
▲back to top |

Question 1[25]
1.1 Define the following terms
1.1.1 Net Present Value
[2]
1.1.2 Internal Rate of Return
[2]
1.1.3 Discounted Cash flow
[2]
1.1.4 Zero-coupon bond
[2]
1.2 Name four (4) Instruments in the money markets.
[4]
1.3 Explain three (3) ways in which Derivatives can be used.
[6]
1.4 VK Investment cc has an existing debt of N$ 2,000000 on which it makes annual payments
at an annual effective rate of LIBORplus 0.5%. VK Investment cc decides to enter a swap with
a notional amount of N$ 2000000 on which it makes annual payments at a fixed annual
effective rate of 3% in exchange for receiving annual payments at the annual effective LIBOR
rate. The annual effective LIBOR rates over the first and second years of the swap contract
are 2.5% and 4% respectively. VK Investment cc does not make or receive any other payments.
Calculate the net interest payment that VK Investment cc makes in the second year.
[7]
Question 2 [25]
2.1 Explain the difference between a Forward and Future contract
[5]
2.2 An investment of N$ 200.00 returns N$ 120.00 at the end of 1st year and N$ 100.00 at
the end of 2nd year. What is the internal rate of return (/ RR)?
[5]
2.3 Consider a 3 x 9 FRAfor £1,000,000 with an FRArate of 3.4%. Suppose the reference rate
is LIBORand the 6-month LIBORon the effective date is 3.7%. Assume ACT/360 and the loan
is for a period of 180 days. Find how much the borrower receives from the lender on the
effective date.
[5]
2.4 Yvonne is considering a project which requires an amount of N$3000.00 and another
amount of N$1000.00 after one year. In two years', time, when the project ends, she
expects an inflow of N$4500.00. Assume that Yvonne can lend and borrow at the same fixed
rate of 7.13% per annum.
2.4.1 what is the internal rate of return (/ RR) of this project?
[7]
2
 |
3 Page 3 |
▲back to top |
2.4.2 ls the above Investment profitable? Explain
[3]
Question 3 [25]
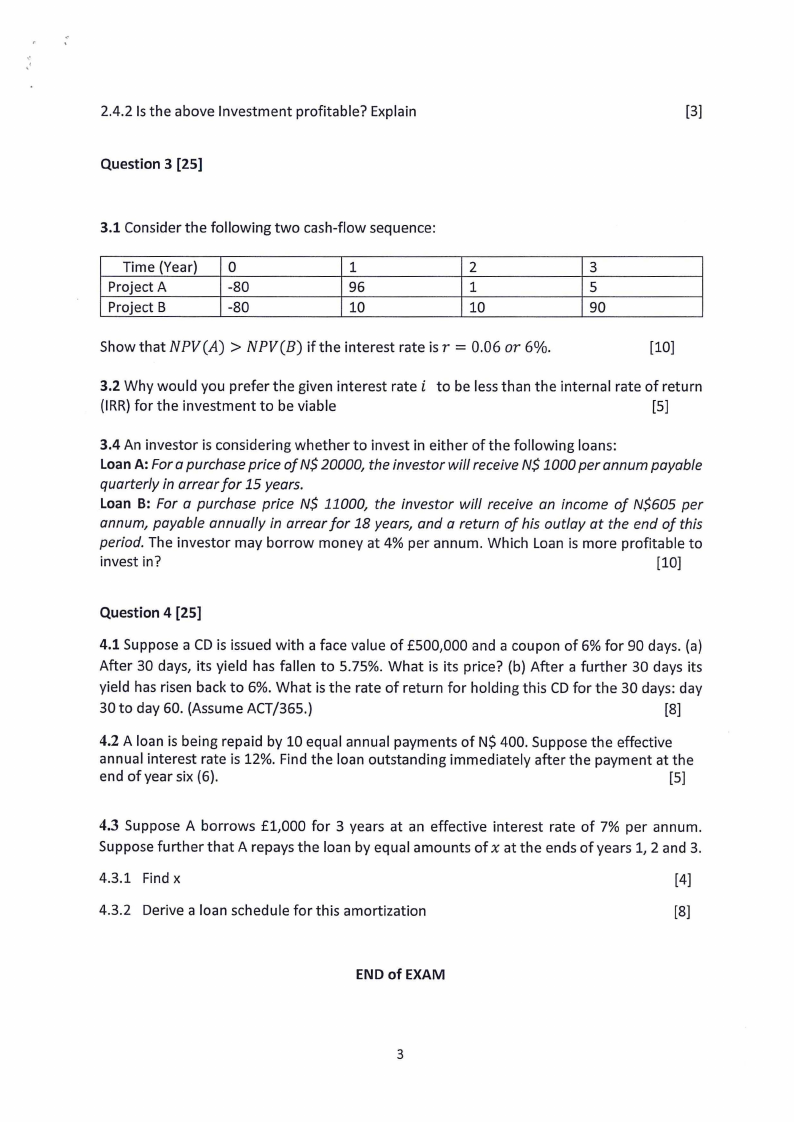
3.1 Consider the following two cash-flow sequence:
Time (Year) 0
1
2
3
Project A
-80
96
1
5
Project B
-80
10
10
90
= Show that NPV(A) > NPV(B) if the interest rate is r 0.06 or 6%.
[10]
3.2 Why would you prefer the given interest rate i to be less than the internal rate of return
{IRR)for the investment to be viable
[5]
3.4 An investor is considering whether to invest in either of the following loans:
Loan A: Fora purchase price of N$ 20000, the investor will receive N$ 1000 per annum payable
quarterly in arrear for 15 years.
Loan B: For a purchase price N$ 11000, the investor will receive an income of N$605 per
annum, payable annually in arrear for 18 years, and a return of his outlay at the end of this
period. The investor may borrow money at 4% per annum. Which Loan is more profitable to
invest in?
[10)
Question 4 [25]
4.1 Suppose a CD is issued with a face value of £500,000 and a coupon of 6% for 90 days. (a)
After 30 days, its yield has fallen to 5.75%. What is its price? (b) After a further 30 days its
yield has risen back to 6%. What is the rate of return for holding this CD for the 30 days: day
30 to day 60. (Assume ACT/365.)
[8]
4.2 A loan is being repaid by 10 equal annual payments of N$ 400. Suppose the effective
annual interest rate is 12%. Find the loan outstanding immediately after the payment at the
end of year six (6).
[5]
4.3 Suppose A borrows £1,000 for 3 years at an effective interest rate of 7% per annum.
Suppose further that A repays the loan by equal amounts of x at the ends of years 1, 2 and 3.
4.3.1 Find x
[4]
4.3.2 Derive a loan schedule for this amortization
[8)
ENDof EXAM
3





