 |
MMP701S - MATHEMATICAL METHODS IN PHYSICS - 1st Opp - JUNE 2022 |
 |
1 Page 1 |
▲back to top |

NAMIBIA UNIVERSITY
OF SCIENCE AND TECHNOLOGY
FACULTY OF HEALTH, APPLIED SCIENCES AND NATURAL RESOURCES
DEPARTMENT OF NATURAL AND APPLIED SCIENCES
QUALIFICATION : BACHELOR OF SCIENCE
QUALIFICATION CODE: 07BOSC
COURSE CODE: MMP701S
SESSION: JUNE 2022
DURATION: 3 HOURS
LEVEL: 7
COURSE NAME:
IN PHYSICS
PAPER: THEORY
MATHEMATICAL METHODS
MARKS: 100
EXAMINER(S)
FIRST OPPORTUNITY EXAMINATION QUESTION PAPER
Prof Dipti R Sahu
MODERATOR: _ | Prof S.C. Ray
INSTRUCTIONS
1. Answer ALL the questions.
2. Write clearly and neatly.
3. Number the answers clearly.
PERMISSIBLE MATERIALS
Non-programmable Calculators
THIS QUESTION PAPER CONSISTS OF 3 PAGES (Including this front page)
 |
2 Page 2 |
▲back to top |
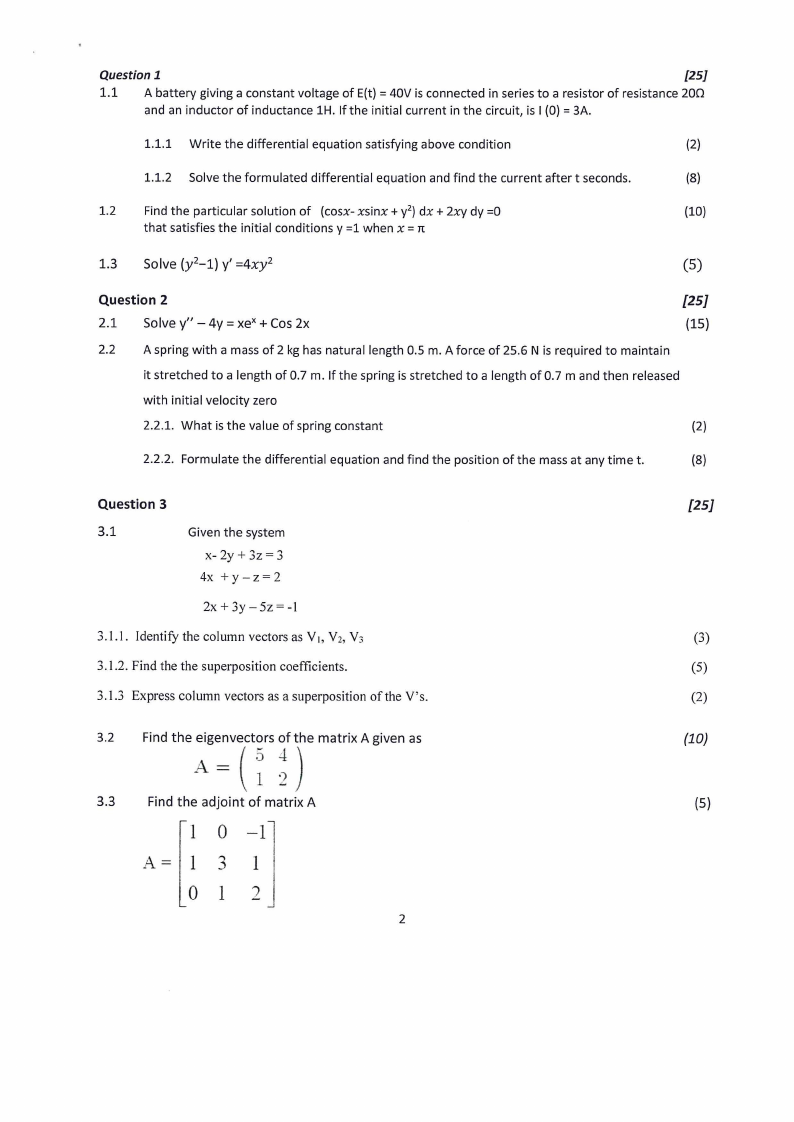
Question 1
[25]
1.1
A battery giving a constant voltage of E(t) = 40V is connected in series to a resistor of resistance 200
and an inductor of inductance 1H. If the initial current in the circuit, is | (0) = 3A.
1.1.1 Write the differential equation satisfying above condition
(2)
1.1.2 Solve the formulated differential equation and find the current after t seconds.
(8)
1.2
Find the particular solution of (cosx- xsinx + y’) dx + 2xy dy =0
(10)
that satisfies the initial conditions y =1 when x =n
1.3. Solve (y2-1) y’ =4xy?
(5)
Question 2
[25]
2.1 Solve y” — 4y = xe* + Cos 2x
(15)
2.2
A spring with a mass of 2 kg has natural length 0.5 m. A force of 25.6 N is required to maintain
it stretched to a length of 0.7 m. If the spring is stretched to a length of 0.7 m and then released
with initial velocity zero
2.2.1. What is the value of spring constant
(2)
2.2.2. Formulate the differential equation and find the position of the mass at any time t.
(8)
Question 3
[25]
3.1
Given the system
x- 2y +3z=3
4x ty-z=2
2x + 3y —5z=-l
3.1.1. Identify the column vectors as V), V2, V3
(3)
3.1.2. Find the the superposition coefficients.
(5)
3.1.3 Express column vectors as a superposition of the V’s.
(2)
3.2
=U) Find the eigenvectors of the matrix A given as
:
12
(10)
3.3
Find the adjoint of matrix A
(5)
1 0 -1]
A={1l 3 1 |
01 2 |
 |
3 Page 3 |
▲back to top |

Question 4
-
4.1
Verify that the functions f(x) = 1, f2(x) = sin x, and f3(x) = cos x are orthogonal
in [—2, 2], and use them to construct an orthonormal set of functions in [—z, 7]
4.2
Determine the first three Hermite polynomials from the generating formula
H,, (y) = (-1)"e*” oi er
[25]
(10)
(5)
What is Gram-Schmidt Orthogonalization Process, explain it mathematically
(10)
WSSNORONR onnmnvonnaess ces eisGettiWirseenssnnevssiievsiaiiny EN D....n0cesssosneesenenssenesvsicesSbsinTsWistensnnseevnnaepqasnpitsdsb FIzVeEeETiesonseceevesnesgaa





