 |
TPH601S - THERMAL PHYSICS - 2ND OPP -JULY 2023 |
 |
1 Page 1 |
▲back to top |

n Am I BI A u n IVER s ITY
OF SCIEnCE Ano TECHn0L0GY
FACULTY OF HEALTH, NATURAL RESOURCES AND APPLIED SCIENCES
SCHOOL OF HEALTH AND APPLIED SCIENCES
DEPARTMENT OF BIOLOGY, CHEMISTRY AND PHYSICS
QUALIFICATION: BACHELOROF SCIENCE(MAJOR AND MINOR}
QUALIFICATION CODE: 07BOSC
LEVEL: 6
COURSE CODE: TPH601S
SESSION: JULY 2023
COURSE NAME: THERMAL PHYSICS
PAPER: THEORY
DURATION: 3 HOURS
MARKS: 100
SECOND OPPORTUNITY/SUPPLEMENTARY EXAMINATION PAPER
EXAMINER(S) DR VAINO INDONGO
MODERATOR: PROFSYLVANUSONJEFU
INSTRUCTIONS
1.
Write all your answers in the answer booklet provided.
2.
Read the whole question before answering.
3.
Begin each question on a new page.
4.
A list of constants and useful formulae are shown on that las page of this paper.
PERMISSIBLE MATERIALS
1. Non-Programmable Scientific Calculator
THIS PAPER CONSISTS OF 4 PAGES
(INCLUDING THIS FRONT PAGE)
llPage
 |
2 Page 2 |
▲back to top |
QUESTION 1
(20)
1.1 Briefly, explain the following thermodynamic terms:
(i)
Internal energy
(2)
(ii) Boundary wall
(2)
(iii) Open system
(2)
(iv) lsochoric process
(2)
(v) The triple point of water
(2)
1.2 Use the following information in the table below to calculate the unknown temperature
T (in Kelvin).
(4)
Temperature (0 C)
100
T
0
Height of mercury (Hg)
8.1 cm
6.5 cm
2.1 cm
1.3 Suppose that the gas tank in your car is completely filled when the temperature is 7°C.
How many gallons will spill out of the 150-gallon steel tank when the temperature rises
to 35°C?
(6)
QUESTION 2
(25)
2.1 Draw and label correctly a P-V diagram of three isotherms of temperatures T1 = 200 K,
T2 = 260 Kand T3 = 230 K. Illustrate an adiabatic curve on the same diagram, cutting
through all isotherms.
(5)
2.2 Convert the following temperatures into Fahrenheit readings:
2.2.1 263.15 K
(3)
2.2.2 101°c
(2)
21Page
 |
3 Page 3 |
▲back to top |
2.4 The compression ratio of a petrol engine is 20.0 to 1; that is, air in a cylinder is
compressed adiabatically to - 1- of its initial volume.
20.0
(a) If the initial pressure is 1.01 x 10 5Pa and the initial temperature is 20°C, find
the final pressure and the temperature after adiabatic compression.
(6)
(b) How much work does the gas do during the compression if the initial volume
of the cylinder is 1.00 L = 1.00 x 10- 3m3 . Use the values Cv = 20.8 J/mol. K
and y = 1.400 for air.
(5)
(c) Hence, find the change in internal energy of the air.
(4)
QUESTION 3
(30)
3.1 Calculate the entropy change of 150 moles of an ideal gas which undergoes a free
expansion from V1 to 8V1 under a constant temperature. R = 8.314 J/mol.K
(6)
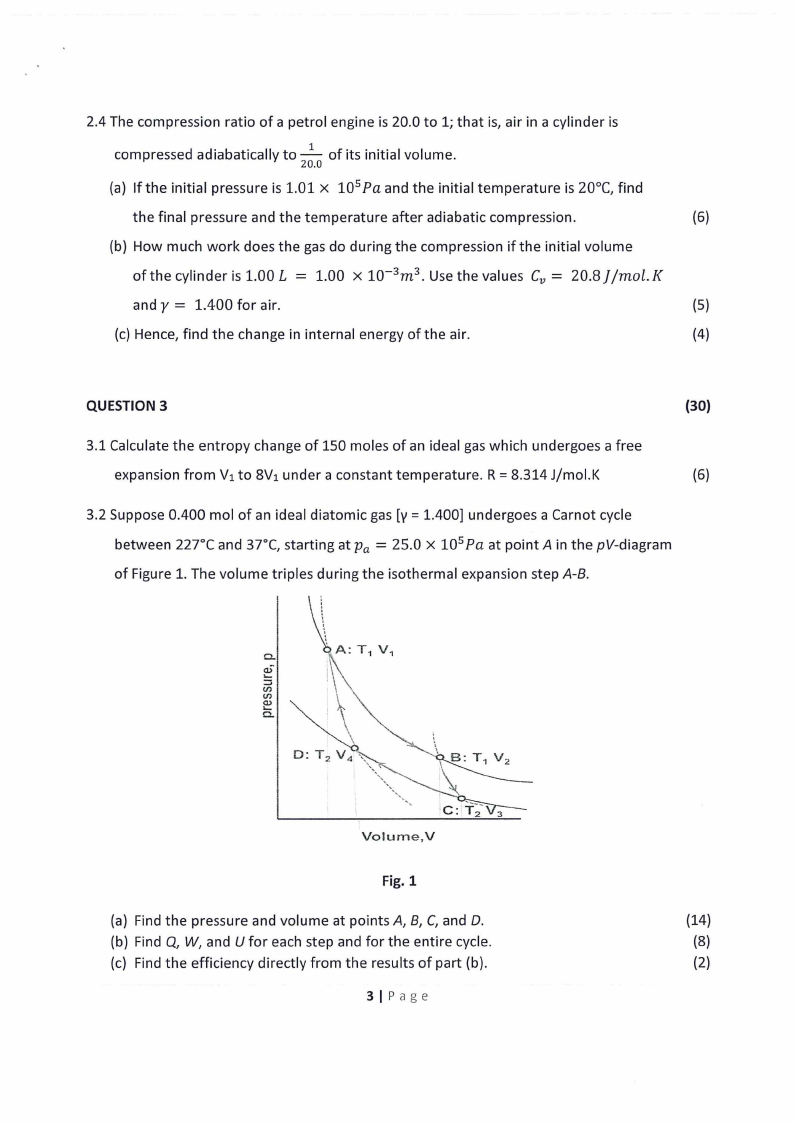
3.2 Suppose 0.400 mol of an ideal diatomic gas [y = 1.400] undergoes a Carnot cycle
= between 227°C and 37°C, starting at Pa 25.0 x 10 5 Pa at point A in the pV-diagram
of Figure 1. The volume triples during the isothermal expansion step A-8.
0.
(.I..)..
::l
VJ
VJ
0.
D: T 2 V 4 ',,,',,,
',,,, _____
Volume,V
Fig.1
(a) Find the pressure and volume at points A, 8, C, and D.
(14)
(b) Find Q, W, and U for each step and for the entire cycle.
(8)
(c) Find the efficiency directly from the results of part (b).
(2)
3IPage
 |
4 Page 4 |
▲back to top |

QUESTION 4
(25)
4.1 A gasoline engine takes in 1.42 x 104 J of heat and delivers 3300 J of work in every cycle.
The heat was obtained by burning gasoline with heat combustion of 4.60 x 104 J/g.
(i) What is the thermal efficiency of the gasoline engine?
(3)
(ii) How much heat is discarded to the environment in each cycle?
(3)
(iii) What mass of fuel is burned per cycle?
(3)
(iv) If the engine goes through 70.0 cycles per second, what is the power output of
the engine in kW?
(3)
4.2 Derive the Maxwell Relation from Helmholtz Free energy, F = U - TS.
(7)
4.3 The speeds of 9 molecules of a gas are 24n, 20n, 25n, 21n, 23n, 30n, 29n, 19n and 27n
all in ms-1, such that n is equal to the number of molecules. Evaluate the rms speed.
(6)
END
Useful equations and constants:
= = < K. E.>
_
-
1
-2
mVrm2 s
1 3k 8 T
-2m-- m
3
-2k 8 T
v = /3P=VJ3Nk=BJT3kBT
rms
Nm
m
The ideal gas law PV = NkBT
Boltzman's constant: kB= 1.38x10- 23 JK-1,
Avogadro's number: NA= 6.022 x 1023 mo1-1
= kBT
Mean free path: 11. .Jzctzp
1 atm = 1.01 x 105 Pa
= mv 2
Maxwell-Boltzmann Distribution: f(V) 4TI [~k ] 2 v 2 e- /zkBT
2TT BT
41Page





