 |
AOR802S - APPLIED OPERATIONS RESEARCHS - 1ST OPP - NOVEMBER 2023 |
 |
1 Page 1 |
▲back to top |

nAm I BIA UnlVERS ITY
OF SCIEnCEAno TECHnOLOGY
FacultyofHealth,Natural
ResourceasndApplied
Sciences
Schoolof Natural and Applied
Sciences
Departmentof Mathematics,
StatisticsandActuarialScience
13JacksonKaujeuaStreet
Private Ba5 13388
Windhoe,
NAMIBIA
T: •26<:612072913
E: msas@nust.na
W: www.nust.nD
QUALIFICATION : BACHELOR of SCIENCE HONOURS IN APPLIED MATHEMATICS
BACHELOR of SCIENCE HONOURS IN APPLIED STATISTICS
QUALIFICATION CODE: 08BSHM
08BSHS
LEVEL:8
COURSE: APPLIED OPERATIONS RESEARCH
COURSECODE: AOR802S
DATE: NOVEMBER 2023
SESSION: 1
DURATION: 3 HOURS
MARKS: 200 (To be converted to 100%)
EXAMINER:
MODERATOR:
FIRST OPPORTUNITY: EXAMINATION QUESTION PAPER
Prof Sunday A. Reju
Prof O/uwole D. Makinde
INSTRUCTIONS
1. Answer all questions on the separate answer sheet.
2. Please write neatly and legibly.
3. Do not use the left side margin of the exam paper. This must be allowed for the
examiner.
4. No books, notes and other additional aids are allowed.
5. Mark all answers clearly with their respective question numbers.
6. Use of COMMA is NOT ALLOWED for a DECIMAL POINT.
PERMISSIBLE MATERIALS
1. Non-Programmable Calculator
ATTACHMENTS
NONE
This paper consists of 4 pages including this front page.
 |
2 Page 2 |
▲back to top |
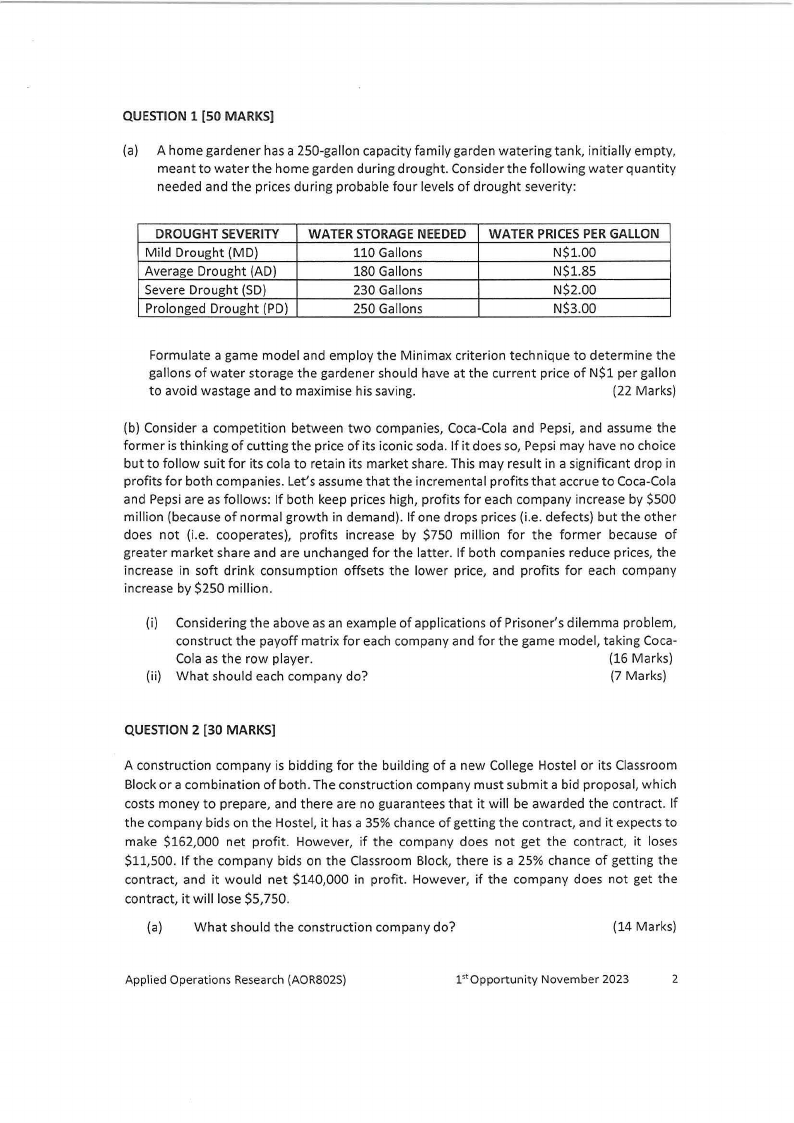
QUESTION 1 [SOMARKS)
(a) A home gardener has a 250-gallon capacity family garden watering tank, initially empty,
meant to water the home garden during drought. Consider the following water quantity
needed and the prices during probable four levels of drought severity:
DROUGHT SEVERITY
Mild Drought (MD)
Average Drought (AD)
Severe Drought (SD)
Prolonged Drought (PD)
WATER STORAGE NEEDED
110 Gallons
180 Gallons
230 Gallons
250 Gallons
WATER PRICES PER GALLON
N$1.00
N$1.85
N$2.00
N$3.00
Formulate a game model and employ the Minimax criterion technique to determine the
gallons of water storage the gardener should have at the current price of N$1 per gallon
to avoid wastage and to maximise his saving.
(22 Marks)
(b) Consider a competition between two companies, Coca-Cola and Pepsi, and assume the
former is thinking of cutting the price of its iconic soda. If it does so, Pepsi may have no choice
but to follow suit for its cola to retain its market share. This may result in a significant drop in
profits for both companies. Let's assume that the incremental profits that accrue to Coca-Cola
and Pepsi are as follows: If both keep prices high, profits for each company increase by $500
million (because of normal growth in demand). If one drops prices (i.e. defects) but the other
does not (i.e. cooperates), profits increase by $750 million for the former because of
greater market share and are unchanged for the latter. If both companies reduce prices, the
increase in soft drink consumption offsets the lower price, and profits for each company
increase by $250 million.
(i) Considering the above as an example of applications of Prisoner's dilemma problem,
construct the payoff matrix for each company and for the game model, taking Coca-
Cola as the row player.
(16 Marks)
(ii) What should each company do?
(7 Marks)
QUESTION 2 [30 MARKS)
A construction company is bidding for the building of a new College Hostel or its Classroom
Block or a combination of both. The construction company must submit a bid proposal, which
costs money to prepare, and there are no guarantees that it will be awarded the contract. If
the company bids on the Hostel, it has a 35% chance of getting the contract, and it expects to
make $162,000 net profit. However, if the company does not get the contract, it loses
$11,500. If the company bids on the Classroom Block, there is a 25% chance of getting the
contract, and it would net $140,000 in profit. However, if the company does not get the
contract, it will lose $5,750.
(a) What should the construction company do?
(14 Marks)
Applied Operations Research (AOR802S)
l'tOpportunity November 2023
2
 |
3 Page 3 |
▲back to top |

(b) How sensitive to the estimate of the probability of the award of a contract is the
decision (i):
• in either to build the Hostel or the Classroom Block?
(6.5 Marks)
• to the net profit for each case, if awarded the contract?
(9.5 Marks)
QUESTION 3 (54 MARKS]
Consider a winning bid of $5.4 million to construct a new plant for a major manufacturer and
the manufacturer needs the plant to go into operation within 40 weeks. Below is the list of
the various project activities. The third column provides important additional information for
coordinating the scheduling of the project crews.
Activity
A
B
C
D
E
F
G
H
I
I
K
L
M
N
Activity Description
Excavate
lay the foundation
Put up the rough wall
Put up the roof
Install the exterior plumbing
Install the interior plumbing
Put up the exterior siding
Do the exterior painting
Do the electrical work
Put up the wcJllboard
Install the flooring
Do the inte,ior painting
tnstal I the exterior fixtures
Install the interior fixtures
Immediate
Predecessors
-
A
B
C
C
E
D
f, G
C
F, I
I
I
H
K, L
Estimated
Duration
2 weeks
4 weeks
10 weeks
6 weeks
4 weeks
5 weeks
7 weeks
9 weeks
7 weeks
8 weeks
4 weeks
5 weeks
2 weeks
6 weeks
(a) Define Critical Path Method (CPM) and Project Evaluation and Review Technique
(PERT).
(3 Marks)
(b) Sketch the project network diagram for the above project.
(16 Marks)
(c) Distinguish between crashing a project and a project activity. Hence obtain the crash
costs per week saved for each activity from the following investigative time-cost trade-off
data.
(37 Marks)
Activity
A
B
C
D
f
F
G
Time
Normal
Crash
2weeks
4 weeks
10 weeks
6 weeks
4 weeks
Sweeks
7weeks
1 week
2 weeks
7weeks
4 weeks
3weeks
3weeks
4weeks
Cost
Normal
5180,000
$320,000
$620,000
5260,000
$410,000
$180,000
$900,000
Crash
s 280,000
5 420,000
5 860,000
5 340,000
s 570,000
s 260,000
s1,020,000
Activity
H
I
1
K
L
M
N
Time
Normal
9 Weeks
7weeks
Bweeks
4weeks
Sweeks
2weeks
6weeks
Crash
6 weeks
5weeks
6weeks
3weeks
3weeks
1 week
3 weeks
Cost
Normal
$200,000
5210,000
$430,000
$160,000
$250,000
5100,000
$330,000
Crash
s 380,000
s 270,000
s 490,000
s 200,000
s 350,000
s 200,000
s 510,000
(d) Discuss your observations.
(4 Marks)
Applied Operations Research (AOR802S)
l"Opportunity November 2023
3
 |
4 Page 4 |
▲back to top |
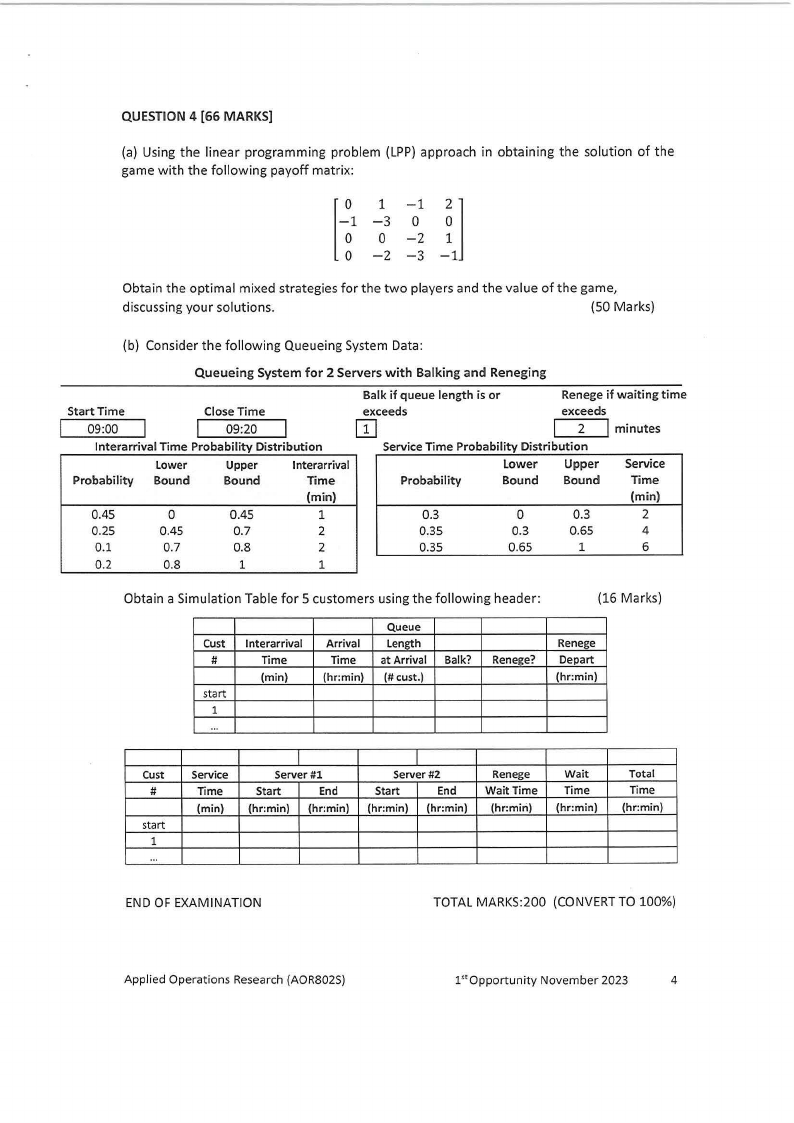
QUESTION 4 [66 MARKS)
(a) Using the linear programming problem (LPP) approach in obtaining the solution of the
game with the following payoff matrix:
1 -1
-3 0
0 -2
-2 -3
Obtain the optimal mixed strategies for the two players and the value of the game,
discussing your solutions.
(SO Marks)
(b) Consider the following Queueing System Data:
Queueing System for 2 Servers with Balking and Reneging
Balk if queue length is or
Renege if waiting time
Start Time
09:00
Close Time
09:20
exceeds
IT]
I exceeds
2
minutes
lnterarrival Time Probability Distribution
Service Time Probability Distribution
Lower
Upper
lnterarrival
Lower
Upper
Service
Probability Bound
Bound
Time
Probability
Bound Bound
Time
(min)
(min)
0.45
0
0.45
1
0.3
0
0.3
2
0.25
0.45
0.7
2
0.35
0.3
0.65
4
0.1
0.7
0.8
2
0.35
0.65
1
6
0.2
0.8
1
1
Obtain a Simulation Table for 5 customers using the following header:
(16 Marks)
Cust
#
start
1
...
lnterarrival
Time
(min)
Arrival
Time
(hr:min)
Queue
Lenirth
at Arrival
(# cust.)
Balk?
Renege?
Renege
Depart
(hr:min)
Cust
#
start
1
...
Service
Time
(min)
Server#l
Start
End
(hr:min) (hr:min)
Server #2
Start
End
(hr:min) (hr:min)
Renege
Wait Time
(hr:miri)
Wait
Time
(hr:min)
Total
Time
(hr:min)
END OF EXAMINATION
TOTAL MARKS:200 (CONVERTTO 100%)
Applied Operations Research (AOR802S)
l"Opportunity November 2023
4





