 |
CAN702S - COMPLEX ANALYSIS - 2ND OPP - JAN 2023 |
 |
1 Page 1 |
▲back to top |

nAm I BI A UnlVE RS ITY
OF SCIEnCE Ano TECHnOLOGY
FACULTY OF HEALTH, APPLIED SCIENCESAND NATURAL RESOURCES
DEPARTMENT OF MATHEMATICS AND STATISTICS
QUALIFICATION: Bachelor of Science in Applied Mathematics and Statistics
QUALIFICATION CODE: 07BSAM
LEVEL: 7
COURSE CODE: CAN702S
COURSE NAME: COMPLEX ANALYSIS
SESSION: JANUARY 2023
DURATION: 3 HOURS
PAPER: THEORY
MARKS: 100
SUPPLEMENTARY /SECOND OPPORTUNITY EXAMINATION QUESTION PAPER
EXAMINER
DR. NEGA CHERE
MODERATOR:
PROF. FORTUNE' MASSAMBA
INSTRUCTIONS
1. Answer ALL the questions in the booklet provided.
2. Show clearly all the steps used in the calculations.
3. All written work must be done in blue or black ink and sketches must
be done in pencil.
PERMISSIBLE MATERIALS
1. Non-programmable calculator without a cover.
THIS QUESTION PAPER CONSISTS OF 3 PAGES (Including this front page}
 |
2 Page 2 |
▲back to top |
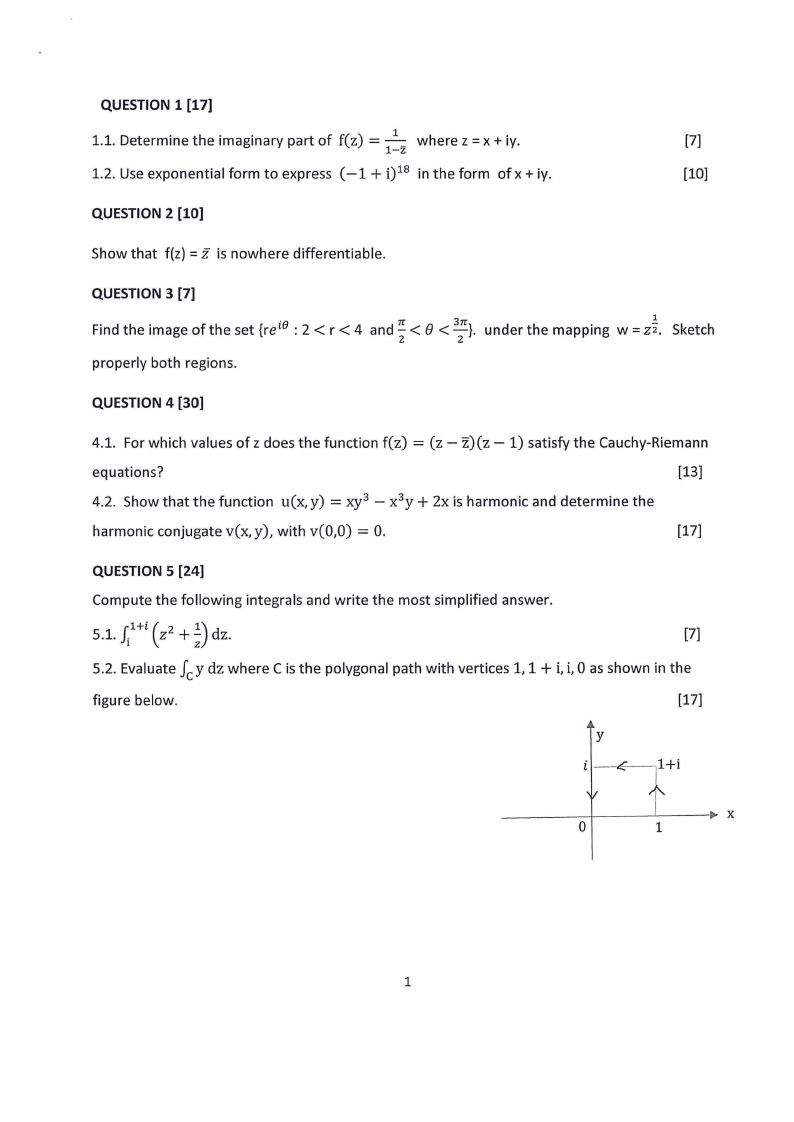
QUESTION 1 [17]
= 1.1. Determine the imaginary part of f(z)
where z = x + iy.
[7]
1-z
1.2. Use exponential form to express ( -1 + i) 18 in the form of x + iy.
[10]
QUESTION 2 [10]
Show that f(z) = z is nowhere differentiable.
QUESTION 3 [7]
zz. Find the image of the set {rei0 : 2 < r < 4 and'!!.< 0 < 3rr}. under the mapping w = 1 Sketch
2
2
properly both regions.
QUESTION 4 [30]
= 4.1. For which values of z does the function f(z) (z - z)(z - 1) satisfy the Cauchy-Riemann
equations?
[13]
= + 4.2. Show that the function u(x, y) xy 3 - x3 y 2x is harmonic and determine the
harmonic conjugate v(x, y), with v(O,O) = 0.
[17]
QUESTION 5 [24]
Compute the following integrals and write the most simplified answer.
5.1. ~1+i ( z 2 +;)dz.
[7]
5.2. Evaluate fc y dz where C is the polygonal path with vertices 1, 1 + i, i, 0 as shown in the
figure below.
[17]
y
i-.:f---,l+i
t
X
0
1
1
 |
3 Page 3 |
▲back to top |

QUESTION 6 [12)
Jrc = 6.1.
23
2
dz where C is the circle lzl 2 traversed once counterclockwise direction. [7]
z +2z-3
f -%--dz z2
6.2.
C z +9
where C is the circle lzl = 2 oriented counterclockwise.
[5]
END OF SUPPLEMENTARY/SECOND OPPORTUNITY EXAMINATION PAPER
2





