 |
MMO702S - MATHEMATICAL MODELLING 2 - 2ND OPP - JAN 2023 |
 |
1 Page 1 |
▲back to top |
n Am I BIA u n IVE Rs ITY
OF SCIEnCE Ano TECHnOLOGY
FACULTY OF HEALTH AND APPLIED SCIENCES
DEPARTMENT OF MATHEMATICS AND STATISTICS
QUALIFICATION: Bachelor of Science in Applied Mathematics and Statistics
QUALIFICATION CODE: 07BSAM
LEVEL: 7
COURSE CODE: MMO702S
COURSE NAME: MATHEMATICAL MODELLING 2
SESSION: JANUARY 2023
DURATION: 3 HOURS
PAPER: THEORY
MARKS: 288 (To be Converted to 100%)
SUPPLEMENTARY/2ND OPPORTUNITY EXAMINATION QUESTION PAPER
EXAMINER
PROF.S. A. REJU
MODERATOR:
PROF.0. D. MAKINDE
INSTRUCTIONS
1. Attempt ALL the questions.
2. All written work must be done in blue or black ink and sketches must be
done in pencil.
3. Use of COMMA is not allowed as a DECIMAL POINT.
PERMISSIBLEMATERIALS
1. Non-programmable calculator without a cover.
THIS QUESTION PAPER CONSISTS OF 3 PAGES (including this front page)
Page 1 of 3
 |
2 Page 2 |
▲back to top |
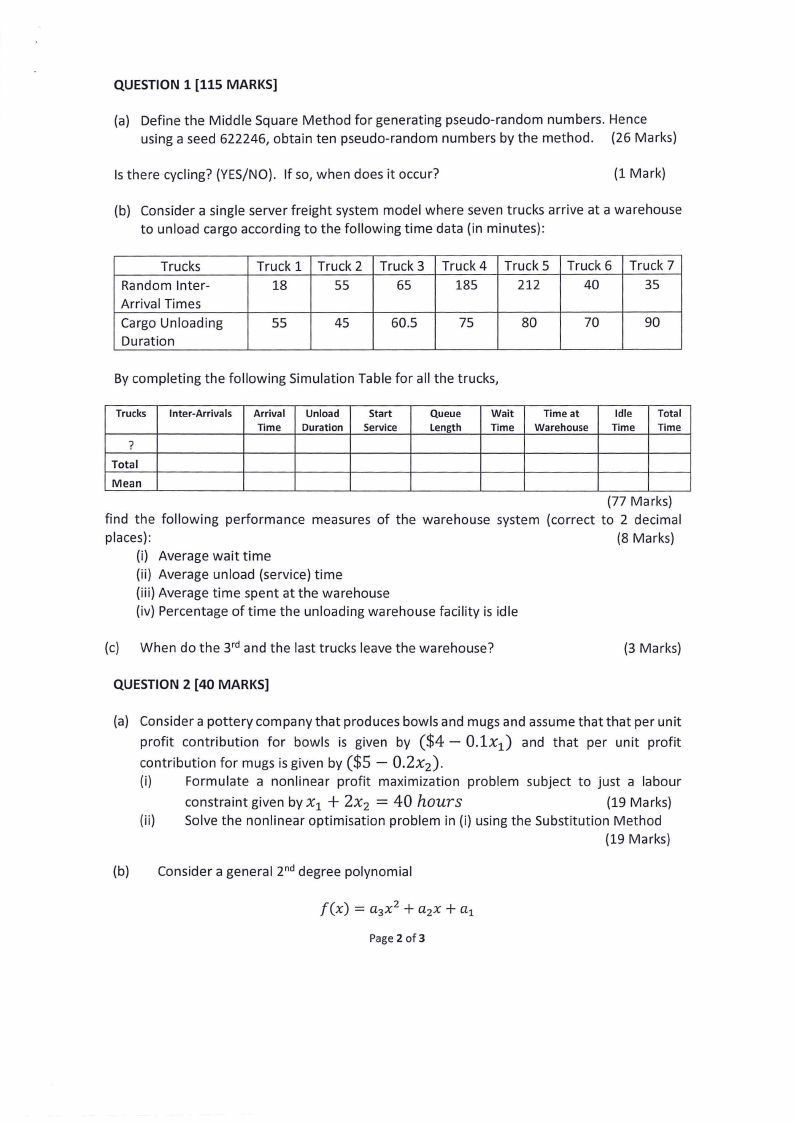
QUESTION 1 [115 MARKS]
(a) Define the Middle Square Method for generating pseudo-random numbers. Hence
using a seed 622246, obtain ten pseudo-random numbers by the method. (26 Marks)
Is there cycling? (YES/NO). If so, when does it occur?
(1 Mark)
(b) Consider a single server freight system model where seven trucks arrive at a warehouse
to unload cargo according to the following time data (in minutes):
Trucks
Random Inter-
Arrival Times
Cargo Unloading
Duration
Truck 1 Truck 2 Truck 3 Truck 4 Truck 5 Truck 6 Truck 7
18
55
65
185
212
40
35
55
45
60.5
75
80
70
90
By completing the following Simulation Table for all the trucks,
Trucks
Inter-Arrivals
Arrival
Time
Unload
Duration
Start
Service
Queue
Length
Wait
Time
Time at
Warehouse
Idle
Time
Total
Time
?
Total
Mean
(77 Marks)
find the following performance measures of the warehouse system (correct to 2 decimal
places):
(8 Marks)
(i) Average wait time
(ii) Average unload (service) time
(iii) Average time spent at the warehouse
(iv) Percentage of time the unloading warehouse facility is idle
(c) When do the 3rd and the last trucks leave the warehouse?
(3 Marks)
QUESTION 2 [40 MARKS]
(a) Consider a pottery company that produces bowls and mugs and assume that that per unit
profit contribution for bowls is given by ($4 - 0.1x1 ) and that per unit profit
contribution for mugs is given by ($5 - 0.2x 2).
(i)
Formulate a nonlinear profit maximization problem subject to just a labour
= + constraint given by x1 2x2 40 hours
(19 Marks)
(ii) Solve the nonlinear optimisation problem in (i) using the Substitution Method
(19 Marks)
(b) Consider a general 2nd degree polynomial
Page 2 of 3
 |
3 Page 3 |
▲back to top |

(i}
State the normal equations for determining the regression coefficients a11 a2
and a3 of the polynomial f (x) for fitting a set of data.
(6 Marks}
(ii) Consider the following data
X
1.2
1.5 2.0 2.6 3.2 4.5 5.2 5.7 6.0 6.8
y
1.1
1.3
1.6
2.0
3.4
4.1
3.2
4.5
2.5
5.2
•
Obtain the normal equations for f(x) defined by (a) above using the above data.
(43 Marks}
•
State the 3-line MATLAB commands for solving the system of three equations
(without determining the values of the regression coefficients}.
(6 Marks)
QUESTION 3 [40 MARKS]
(a) A small-scale vocational business firm produces two farming implements: hoes and
shovels and realises a net unit profit of N$125 per hoe and N$140 per shovel. Assume
that the firm has up to 250 square metres of iron sheet and 200 metres of wood length
to devote to a small farming project plus a signed contract of supplying 10 hoes and 15
shovels to a family farm during the period of the project. Moreover, assume that it
requires 2 square metres of iron and 0.65 metre of wood to fabricate a hoe and 3 square
metres of iron and 0.85 metre of wood to produce a shovel. Formulate and solve the
model for maximising the firm's profits for hoes and shovels.
(20 Marks}
(b} (i} Define post-optimality analysis for linear optimisation problems
(5 Marks}
(ii} Discuss the analysis for change in the firm's profits on hoes, showing all
expressions to support your conclusion.
(15 Marks}
QUESTION 4 [40 MARKS]
(a} A spring with a mass of 2kg has natural length 0.5m and a force of 25.6N is required to
maintain it stretched to a length of 0.7m and then released with initial velocity 0.
Formulate an appropriate model equation and solve to obtain the expression for the
position of the mass at any time t, stating all physical laws to support the fundamental
equations and associated concepts of your model and its solution before using the given
data.
(25 Marks)
(b) Then suppose that the mass-spring system in (a) is immersed in a fluid with damping
= constant c 40. Stating the general model differential equations for the damped
system, find the position of the mass at any time t if it starts from the equilibrium
position and is given a push to start it with an initial velocity of 0.6m/s.
(15 Marks)
END OF EXAMINATION
TOTAL MARKS:288 CONVERTTO 100%
Page 3 of 3





