 |
AMI810S-ADVANCED MICROECONOMICS-2ND OPP-JULY 2022 |
 |
1 Page 1 |
▲back to top |

nAmlBIA UnlVERSITY
OF SCIEnCE Ano TECHnOLOGY
FACULTY OF MANAGEMENT SCIENCES
DEPARTMENT OF ACCOUNTING, ECONOMICS AND FINANCE
QUALIFICATION: BACHELOR OF ECONOMICS
QUALIFICATION CODE: 07BECO
LEVEL: 8
COURSE CODE: AMl810S
COURSE NAME: ADVANCED MICROECONOMICS
SESSION: JUNE 2022
DURATION: 3 HOURS
PAPER: THEORY
MARKS: 100
EXAMINER(S)
SECOND OPPORTUNITY EXAMINATION QUESTION PAPER
MR. PINEHAS NANGULA
MODERATOR: Dr Ernest Ngeh Tingum
INSTRUCTIONS
1. Answer ALL the questions.
2. Write clearly and neatly.
3. Number the answers clearly.
PERMISSIBLE MATERIALS
1. Scientific calculator
2. Pen and Pencil
3. Ruler
THIS QUESTION PAPER CONSISTS OF_ 4_ PAGES {Including this front page)
 |
2 Page 2 |
▲back to top |

QUESTION ONE
[25MARKS]
John has the utility function U(Z,B)= 1oz0AB0·6, where Z denotes the amount of food consumed
and B the amount of clothing. Now suppose that he has an income of N$100 per week and that
the price of clothing is Pb= N$10 per unit. Suppose that the price of food is initially Pz1 = N$20
per unit and that the price subsequently falls to P12 =N$10 per unit.
Use income, substitution, and total effect to determine if pizzais a normal good or inferior good
or giffen good.
QUESTION TWO
[30MARKS]
a) What is a basket (or a bundle) of goods?
[2 marks]
b) What does the assumption that preferences are complete mean about the consumer's
ability to rank any two baskets?
[4 marks]
c) Give an example of preferences that do not satisfy the assumption that preferences are
transitive.
[4 marks]
d) Ali is a university student who receives a monthly stipend from his parents of N$5,000.
He uses this stipend to pay rent for housing and to go to the movies. In the town where Ali
goes to university, each square foot of rental housing costs N$2.50 per month. Eachmovie
he attends costs N$12. Let x denote the square feet of housing, and let y denote the
number of movies he attends per month.
i) What is the expression for Ali's budget constraint?
[2 marks]
ii) Draw a graph of Ali's budget line.
[2 marks]
iii) What is the maximum number of square feet of housing he can purchase given his
monthly stipend?
[2 marks]
iv) What is the maximum number of movies he could attend given his monthly stipend
[2 marks]
v) Suppose Ali's parents increase his stipend by 10 percent. At the same time, suppose
that in the university town where he lives, all prices, including housing rental rates and
movie ticket prices, increase by 10 percent. What happens to the graph of Ali's budget
line?
2
[2 marks]
 |
3 Page 3 |
▲back to top |
e) Esther consumes two goods: housing and food.
i) Suppose we are given Esther's marginal utility of housing and his marginal utility of food
at the basket he currently consumes. Can we determine his marginal rate of
substitution of housing for food at that basket? If yes, work it out?
[4marks]
ii) Suppose we are given Esther's marginal rate of substitution of housing for food at the
basket he currently consumes. Can we determine his marginal utility of housing and his
marginal utility of food at that basket? If yes, work it out?
[6 marks]
QUESTION THREE
[25MARKS]
= = Suppose Cola and Pepsi's demand curves are given by Ql {64 + 2P2) -4Pl and Q2 {50 + Pl)-
5P2, respectively. Coca-Cola's marginal cost is $5 per unit, and Pepsi's marginal cost is $4 per
unit
a) What is Coca-Cola's profit-maximizing price when Pepsi's price is $8?
[7 marks]
b) What is the equation of Coca-Cola's price reaction function (i.e., Coca-Cola's profit-
maximizing price when Pepsi sets an arbitrary price P2)?
[8 marks]
c)
What are Coca-Cola'sand Pepsi'sprofit-maximizing prices and quantities at the Bertrand
equilibrium?
[10 marks]
QUESTION FOUR
[20marks]
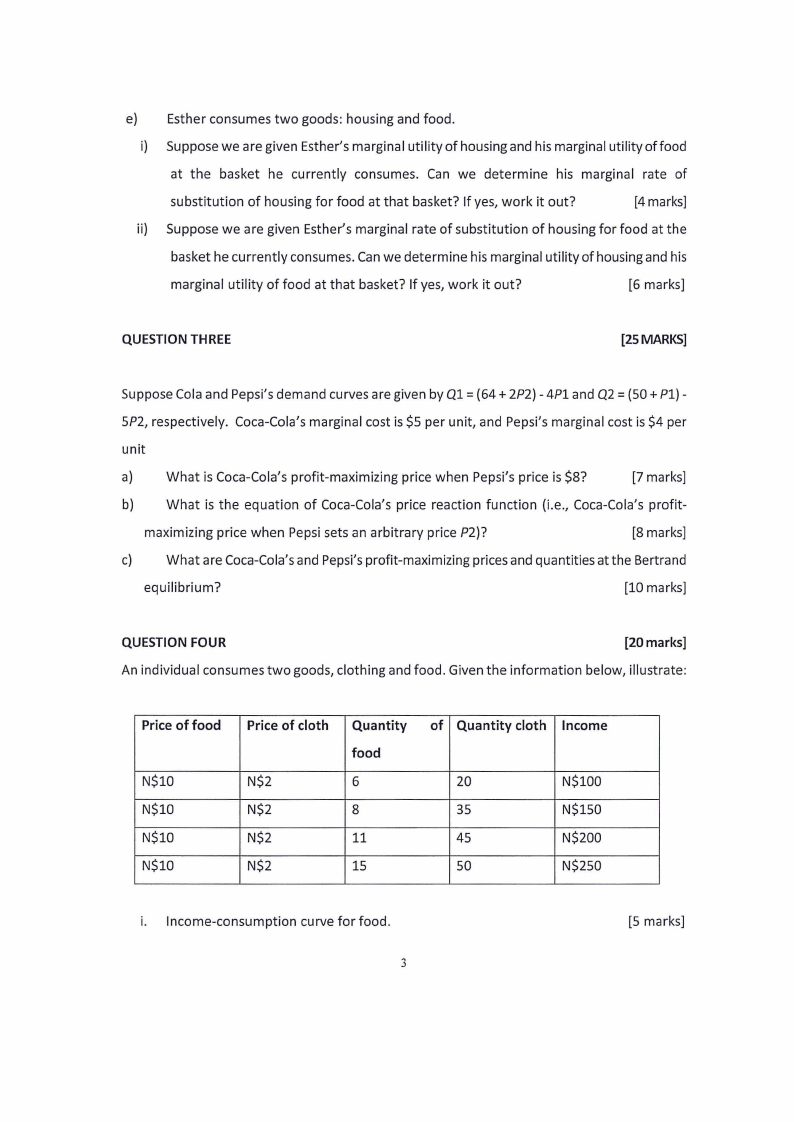
An individual consumes two goods, clothing and food. Given the information below, illustrate:
Price of food
N$10
N$10
N$10
N$10
Price of cloth
N$2
N$2
N$2
N$2
Quantity
food
6
8
11
15
of Quantity cloth Income
20
N$100
35
N$150
45
N$200
50
N$250
i. Income-consumption curve for food.
3
[5 marks]
 |
4 Page 4 |
▲back to top |

ii. Income-consumption curve for clothing.
iii. Engel curve for clothing.
iv. Engel curve for food.
All the best
[5 marks]
[5 marks]
[5 marks]
4





