 |
ITM111S - INTRODUCTION TO MATHEMATICS - 1ST OPP - NOV 2022 |
 |
1 Page 1 |
▲back to top |
nAmlBIA UnlVERSITY
OF SCIEnCE Ano TECHnOLOGY
FACULTYOF HEALTH,APPLIEDSCIENCESAND NATURAL RESOURCES
DEPARTMENTOF MATHEMATICSAND STATISTICS
QUALIFICATION:Bachelor ofTechnology: Geo-Information Technology, Bachelor of Human Resources
Management, Bachelor of Marketing, Bachelor of Transport Management, Bachelor of Business
Administration, Bachelor of Agricultural Management, Bachelor of Horticulture
QUALIFICATION CODE:
07BGIT,07BHRM,07BMAR,07BBAD,27BAGR,07BTRM,07BHOR
NQF LEVEL:5
COURSENAME: INTRODUCTION TO MATHEMATICS
(BUSINESS AND MANAGEMENT)
COURSECODE: ITM111S
DATE: NOVEMBER 2022
DURATION: 3 HOURS
PAPER:THEORY
MARKS: 100
EXAMINER
FIRSTOPPORTUNITY EXAMINATION QUESTION PAPER
Ms A. SAKARIA,Ms Y. NKALLE, Ms P. NGHISHIDIVALI, Mr B. OBABUEKI, Mr F.
NDINODIVA
MODERATOR:
Mr I. NDADI
INSTRUCTIONS
1. Answer ALL the questions in the answer sheet.
2. QUESTION 1 of this question paper entail multiple choice questions
with options A to D. Write down the letter corresponding to the best
option for each question.
3. For QUESTION 2 indicate whether the given mathematical statements
are true (T) or false (F).
4. QUESTION 3 show clearly all the steps used in the calculations.
PERMISSIBLEMATERIALS
1. Non-programmable calculator without a cover.
THIS QUESTION PAPERCONSISTSOF 4 PAGES(Including this front page)
~1~
 |
2 Page 2 |
▲back to top |

QUESTION 1 (30 MARKS]
Write down the letter corresponding to the best option for each question in the answer
booklet/sheet provided.
1.1 The average of two numbers is 7, and three times the difference between them is 18.
What are the numbers?
[3]
A. 52 and 34
B. 7 and 7
C. 10 and 4
D. 8 and 6
1.2 Find the Highest Common Factor (HCF)of the numbers 255, I05 and 90.
A. 45
B. 3
C. 5
[3]
D. 15
1.3 The factors of the expression -ai+2x 2 -aix+2x are:
[3]
A. (x-l)(2x+ai)
B. (x-l)(2x-ai)
C. (2x-ai)(x+l) D. ai(2x 2 -2x-l)
6x-42x 3
sx- 1.4 The expression
simplifies to:
3
[3]
3
A.-4
2x
3
C.-2
2x
D. lx2
2
1.5 Given sets .0={1,2,3,5,7,ll,12}, A={l,2,3,7,11} and B={3,5,7,ll,12},
find (An BY.
[3]
A. (AnBY ={l,2,5,12}
B. (AnBr ={1,2,7,11}
C. (An BY={37,,11}
D. (An Br ={3,5,12}
1.6 Expand and simplify the expression (x-xy)2-x 1 -x(-2xy) .
[3]
B. x2y2
C. x-xy
1.7 The solutions of the quadratic equation 4x 2 -1 =Oare:
A. (2x+ 1)(2x- l) B. x=- 1 andx=-- 1
2
2
C. X = 0 andX =- l
[3]
D. X = 4 andX =l
 |
3 Page 3 |
▲back to top |
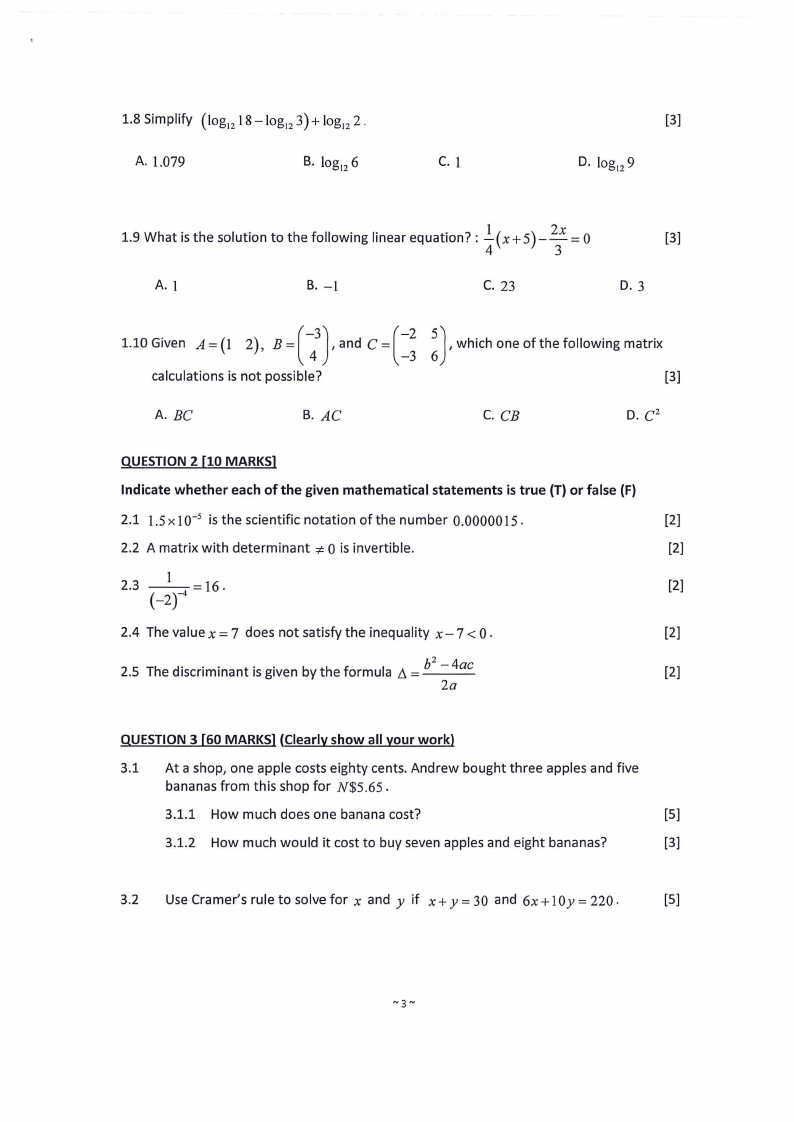
1.8 Simplify (log 12 18- log 12 3) + log 12 2.
A. 1.079
B. log 12 6
C. 1
[3]
D. log 12 9
1.9 What is the solution to the following linear equation?: _!_x(+ 5)- 2x = 0
4
3
A. 1
B. -1
C. 23
[3]
D. 3
1.10 Given A= (l 2), B =(~}and
calculations is not possible?
=!:} C =(
which one of the following matrix
[3]
A.BC
B. AC
C. CB
D. C 2
QUESTION 2 (10 MARKS)
Indicate whether each of the given mathematical statements is true (T) or false (F)
2.1 1.5 x 10-5 is the scientific notation of the number 0.0000015.
[2]
* 2.2 A matrix with determinant 0 is invertible.
[2]
2.3 --=116,
[2]
(-2t
2.4 The value x =7 does not satisfy the inequality x- 7 < 0.
[2]
2.5 The d.1scn. m.inant .1sgi.ven by t he f ormu Ia =-b-i--4ac
[2]
2a
QUESTION 3 (60 MARKS) (Clearly show all your work)
3.1 At a shop, one apple costs eighty cents. Andrew bought three apples and five
bananas from this shop for N$5.65.
3.1.1 How much does one banana cost?
[5]
3.1.2 How much would it cost to buy seven apples and eight bananas?
[3]
3.2 Use Cramer's rule to solve for x and y if x + y = 30 and 6x + l 0y = 220.
[5]
 |
4 Page 4 |
▲back to top |

3.3 Out of 180 students, 50 students play Piano {P), 68 play Guitar {G), and 59 play Flute
{F). Also 35 play Piano and Guitar, 40 play Guitar and Flute and 25 play Piano and
Flute. 15 play all, Piano, Guitar, and Flute.
3.3.1 Draw a Venn diagram to represent this information.
[4]
3.3.2 Find the number of students who:
3.3.2.1 Play piano or guitar
[2]
3.3.2.2 Do not play any of the instruments
[2]
3.3.2.3 Play flute and piano but not guitar
[2]
3.4 Find the values of the letters x, y and z given that:
[6]
3.5.1 The matrix 3A-_!_B
[4]
2
3.5.2 The product of CA
[2]
3.6 The inverse of matrix B
[5]
3.7 An investment amount is expected to grow from N$70000.00 to N$120000.00
in 4 years when the interest rate is compounded monthly. Calculate the annual
interest rate that will give the expected growth.
[6]
3.8 Find the 30th term of the progression 9; 13;17; 21;....
[4]
3.9 Findthesumofthefirst
110 terms of the series 5+12+19, ....
[5]
L ( i). 5
3.10 Determine the value of the series
12 -
[5]
i=2
END OF EXAMINATION QUESTION PAPER





