 |
MTA611S - MATHEMATICS FOR AGRIBUSINESS - 1ST OPP - JUNE 2024 |
 |
1 Page 1 |
▲back to top |

nAmlBIA unlVERSITY
OF SCIEn CE Ano TECHn OLOGY
FACULTYOF HEALTH,NATURALRESOURCESAND APPLIEDSCIENCES
SCHOOLOF AGRICULTUREAND NATURALRESOURCESSCIENCES
DEPARTMENT OF AGRICULTURALSCIENCESAND AGRIBUSINESS
QUALIFICATION: BACHELOROF SCIENCEIN AGRICULTURE
QUALIFICATION CODE:07BAGA
LEVEL: 7
COURSECODE: MTA611S
COURSENAME: MATHEMATICS FORAGRIBUSINESS
SESSION:JUNE 2024
PAPER: 1
DURATION: 3 HOURS
MARKS: 100
EXAMINER($)
FIRSTOPPORTUNITY EXAMINATION QUESTION PAPER
MR MWALA LUBINDA
MODERATOR: MR TEOFILUSSHIIMI
INSTRUCTIONS
1. Attempt all questions.
2. Write clearly and neatly.
3. Number the answers clearly & correctly.
PERMISSIBLE MATERIALS
1. All written work MUST be done in blue or black ink
2. Calculators allowed
3. The LASTPAGEhas FORMULA
4. No books, notes or other additional aids are allowed
THIS QUESTION PAPERCONSISTSOF 6 PAGE{Including this front page)
 |
2 Page 2 |
▲back to top |

Mathematics for Agribusiness
MTA611S
QUESTION ONE
[MARKS]
= a. Given a cost function c(q) (q - 3) 0·5, where c(q) is the cost of production in
thousands of dollars and q is the quantity of output produced in thousands of units. Use
this information to answer the questions below.
i. Find the c(12).
(3)
ii. Find the domain value that corresponds to a range value of 4.
(3)
iii. Use interval or set notation to express the appropriate domain of the function.
(6)
b. Consider an Agribusiness whose production process is represented by a univariate
quadratic function that exhibits a maximum output level and possesses roots at zero
and ten units of the input variable. Based on this information, answer the questions
below.
i. Derive the mathematical expression of the Agribusiness's production function.
(3)
ii. Find the range and domain values at the maximum point of the production
function.
(5)
iii. Draw and label a graph that illustrates the production function. The graph must
clearly show the roots, maxima, and y-intercept points of the production
(5)
function.
TOTAL MARKS
[25]
First Opportunity Question Paper
Page 2 of 6
June 2024
 |
3 Page 3 |
▲back to top |

Mathematics for Agribusiness
MTA6115
QUESTION TWO
= a. Given a multivariate production function q(l, k) 10l 0·25 k 0·75 , find the following
derivatives qk, qkk and qkl·
[MARKS]
(5)
b. Suppose an Agribusiness's total revenue function, r(q), and cost function, c(q), are
represented as:
r(q) = 4000q - 33q 2
c(q) = 2q 3 - 3q 2 + 400q + 5000
(8)
Assuming q is the quantity of output, compute the profit-maximizing output level and
maximum profit. Furthermore, proves that the profit-maximizing output level is the
relative maximum. Show all your calculations.
= c. Using the Difference Quotient, find the first derivation of the function g(q) 2q 2 .
Show all the steps.
(4)
d. Find the equation of a straight line that is tangent to the function:
f(q) = In (4q 2 + 4q - 3)
(8)
at X = 1.
TOTAL MARKS
[25)
First Opportunity Question Paper
Page 3 of 6
June 2024
 |
4 Page 4 |
▲back to top |

Mathematics for Agribusiness
MTA611S
QUESTION THREE
a. For each of the following cost functions, find c'(q):
= i. c(q)
ln(3q 4 )
ii. c(q)
lnq
qZ
iii. c(q) = q ln(q 2 + 4)
[MARKS]
(3)
(3)
(4)
b. Suppose a farmer faces the following cost function in his tomato production enterprise:
c(k, l) = 3k 3 + 1.Sl2 - 18kl + 17
Where c(k, l) is the multivariate production function with labour, l , and capital, k, as
(15)
the main inputs. Based on this information find: (i) the critical points of the cost function;
and (ii) for each critical point, determine if the function is at a relative maximum,
relative minimum, inflection point, or saddle point.
TOTAL MARKS
[25)
First Opportunity Question Paper
Page 4 of 6
June 2024
 |
5 Page 5 |
▲back to top |

Mathematics for Agribusiness
MTA611S
QUESTION FOUR
a. Solve the following indefinite integral:
[MARKS]
(5)
b. Solve the following definite integral:
f3 2q
i qz + 5 dq
(5)
c. Suppose you have observed that the rate at which production in a small stock enterprise
changes with respect to improvement in carry capacity is given by:
dy
dx = 100\\IX
Where dy is the rate of change with dy being the change in output (i.e., number of
dx
animals) and dx being the change in carrying capacity. Furthermore, y and x represent
(5)
the number of animals and carrying capacity (i.e., number of small stock units (SSU)per
hectare), respectively. Derive an equation that represents the output, y, as a function of
carrying capacity, x, given initial conditions of 500 animals and carrying capacity of 3
SSUper hectare.
d. Consider an agribusiness that faces a budget constraint of $108,000 and prices of labour
(l) and capital (k) of N$4 and N$3 per unit. Suppose the agribusiness's production
function is represented by:
q(k, l) = ko.4ko.s
(10)
Find the units of l and k that maximises the agribusiness's output. Furthermore,
compute and interpret the lambda (il) value.
TOTAL MARKS
[25)
THE END
First Opportunity Question Paper
Page 5 of 6
June 2024
 |
6 Page 6 |
▲back to top |
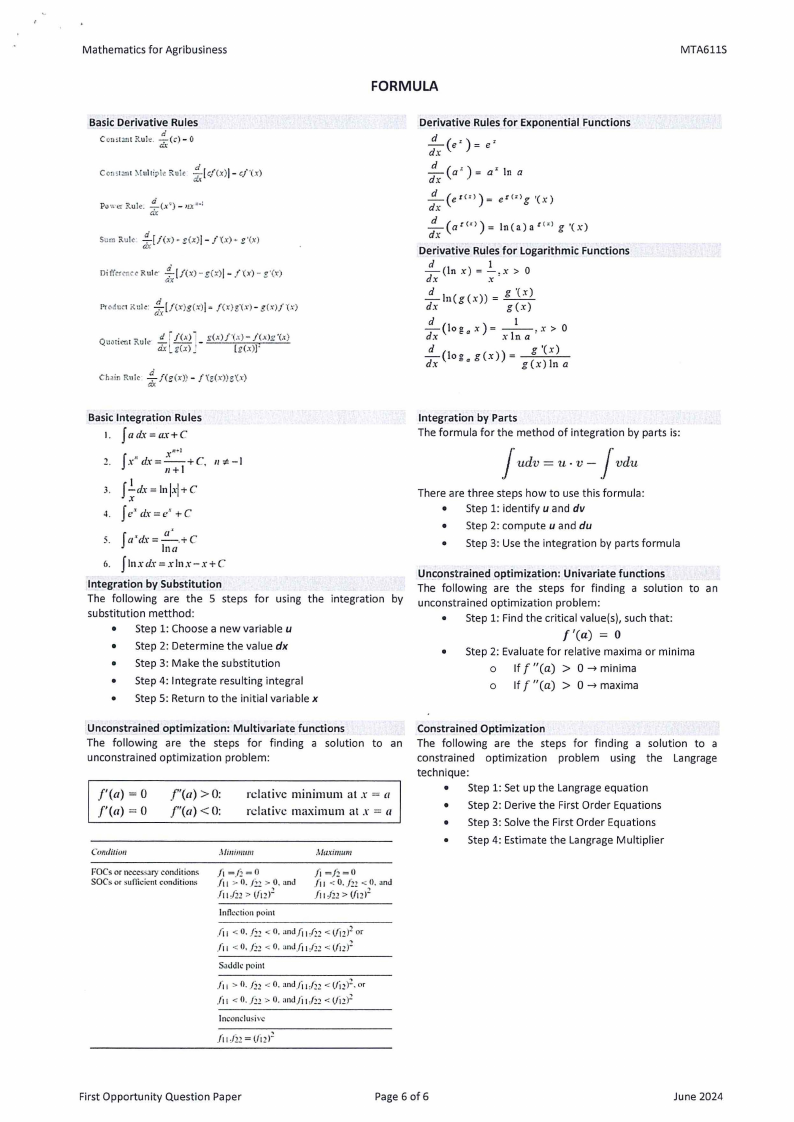
Mathematics for Agribusiness
MTA611S
FORMULA
Basic Derivative Rules
Coustaut R.uh=. ~(c)-0
c:x
ConSIJIII ~lulliple Rul, {[cf(x)[- cf'(x)
Sum Ruk ..:;..[/(x)-g(x)j-/Xx)-g'(x)
ax
l'ro,iuct Kuk ~a[xf(x)g(x)[
J(x) g'(x)- g(x)f'(x)
Ch,in Ruic .!;.-/(e(x))- /Xe(x))eX~)
"'
Derivative Rules for Exponential Functions
-d ( e·. ) = e·•
dx
-d(') a = a 'I n a
dx
!!._(er<•>)= er<rlg '(x)
dx
_::._(at<•>)= ln(a)a r<•>g '(x)
dx
Derivative Rules for Logarithmic Functions
-(dIn x) = -I, x > 0
dx
x
-ldn(g(x))
dx
= _uo_'·(_x-)
g (x)
-d(log
dx
-(dlog
dx
0 x)=
--,xl
x In a
>0
= 0 g(x))
0u
'(~)
•
g(x)lna
Basic Integration Rules
f I. adr=ax+C
;;+n•I
2. fxr.dx=
+c, 11;,,-l
1
3. J-!.d=rIn 1.,1C+
JX
4. e' dx = c•' + C
f 5. a'd,· = ~-+ C
Ina
6. Jlnx,fr=xlnx-x+C
Integration by Substitution
The following are the 5 steps for using the integration by
substitution metthod:
• Step 1: Choose a new variable u
• Step 2: Determine the value dx
• Step 3: Make the substitution
• Step 4: Integrate resulting integral
• Step 5: Return to the initial variable x
Integration by Parts
The formula for the method of integration by parts is:
.! ;·udv = u •v- vd11.
There are three steps how to use this formula:
• Step 1: identify u and dv
• Step 2: compute u and du
• Step 3: Use the integration by parts formula
Unconstrained optimization: Univariate functions
The following are the steps for finding a solution to an
unconstrained optimization problem:
• Step 1: Find the critical value(s), such that:
f '(a) = 0
• Step 2: Evaluate for relative maxima or minima
o If f "(a) > 0 minima
o If f "(a) > 0 maxima
Unconstrained optimization: Multivariate functions
The following are the steps for finding a solution to an
unconstrained optimization problem:
f'(a) = 0
f'(a) = 0
f'(a) > 0:
f'(a) < 0:
relative minimum at x = a
relative maximum at x = a
Comlilion
FOCs or ncccss.1ryconditions
SOCs or sufficient conditions
.\\Jinimum
/1 =h = 0
/11 > 0. hl 0, and
/11/12 > ((12>2
lnOcclion poinl
Maximum
Ji =h =0
/11 <0./!2 <0. and
/11.J}.2 > if1d
Constrained Optimization
The following are the steps for finding a solution to a
constrained optimization problem using the Langrage
technique:
• Step 1: Set up the Langrage equation
• Step 2: Derive the First Order Equations
• Step 3: Solve the First Order Equations
• Step 4: Estimate the Langrage Multiplier
/11 < 0. h.2 < 0.and/11 /i2 < \\/i2/ or
/11 < 0, h.2 < 0. anJ.fj 1,½2< Ui2) 2
Saddle poinl
/11 > 0. hi< 0, andjj 1,/~2 < Vi2) 2. or
/11 < 0. h! > 0. and Ji 1!12< 1Ji2)2
Inconclusive
First Opportunity Question Paper
Page 6 of 6
June 2024





