 |
MTA611S - MATHEMATICS FOR AGRIBUSINESS - 2ND OPP - JUNE 2024 |
 |
1 Page 1 |
▲back to top |

nAmlBIA UnlVERSITY
OF SCIEn CE Ano TECHn OLOGY
FACULTYOF HEALTH,NATURALRESOURCESAND APPLIEDSCIENCES
SCHOOLOF AGRICULTUREAND NATURALRESOURCESSCIENCES
DEPARTMENTOF AGRICULTURALSCIENCESAND AGRIBUSINESS
QUALIFICATION: BACHELOROF SCIENCEIN AGRICULTURE
QUALIFICATION CODE:07BAGA
LEVEL: 7
COURSECODE: MTA611S
COURSENAME: MATHEMATICS FORAGRIBUSINESS
SESSION:JULY2024
PAPER:2
DURATION: 3 HOURS
MARKS: 100
SECONDOPPORTUNITY/ SUPPLEMENTARYEXAMINATION QUESTION PAPER
EXAMINER(S} MR MWALA LUBINDA
MODERATOR: MR TEOFILUSSHIIMI
INSTRUCTIONS
1. Attempt all questions.
2. Write clearly and neatly.
3. Number the answers clearly & correctly.
PERMISSIBLEMATERIALS
1. All written work MUST be done in blue or black ink
2. Calculators allowed
3. The LASTPAGEhas FORMULA
4. No books, notes or other additional aids are allowed
THIS QUESTION PAPERCONSISTSOF 6 PAGE(Including this front page)
 |
2 Page 2 |
▲back to top |

Mathematics for Agribusiness Management
MTA 61 IS
QUESTION ONE
a. Consider a function f(a) = In (a 2 - 2a - 8), compute f(lO), and use interval or set
notation to express the domain of a function.
[MARKS]
(6)
= b. Using the limits concept, determine if the function below is continuous at k l.
2k - 2
(6)
g(k) k2-k
c. Suppose you know that the production function that expresses the relationship
between table grapes output (q) and fertilizer application rate (x) is a quadratic function
that has: (i) maxima point and (ii) roots at Oand 50. Based on the provided information,
answer the questions below
i. Derive the mathematical equation of the production function.
(3)
ii. Find the critical point of the production function you have derived in c(i).
(S)
iii. Draw and label a graph that illustrates the production function. The graph must
clearly show the roots, maxima, and y-intercept points of the production
(S)
function.
TOTAL MARKS
[25)
Second Opportunity Question Paper
Page 2 of 6
July 2024
 |
3 Page 3 |
▲back to top |

'Mathematics for Agribusiness Management
MTA61 IS
QUESTION TWO
[MARKS]
a. Suppose a small-scale vegetable vendor told you that her original capital value for a
vending business is N$25,000. Each week her business income and expenses are
(S)
N$12,000 and N$7,000, respectively. If all profits are retained in the business, express
the value of the business, V, at the end of time, t, weeks as a function oft.
= b. Given a function h(t) 4t 2 , use the Difference Quotient to h'(t). Show all the steps.
(4)
c. Find:
.I.
}I' m--(-2- + h) 2 - 4
h->O
h
(2)
ii. g"(x), given that g(x) = x 3 - x 2 + 10.
(3)
= iii. f'(x), given that f(x) In ( 3x(zx-l)).
Sx-2
(S)
d. Find the equation of a straight line that is tangent to the curve:
g(q) = q2 - 2q - 24
(6)
at q = 2.
TOTAL MARKS
[25)
Second Opportunity Question Paper
Page 3 of6
July 2024
 |
4 Page 4 |
▲back to top |

Mathematics for Agribusiness Management
MTA61 IS
QUESTION THREE
a. Consider the following exponential function:
z(x, y) = 37-2xy2
Find Zx, Zyy, and Zyx·
[MARKS]
(10)
b. Given the following function:
z(x,y) = 2y 3 - x 3 + 147x - 54y + 12
(15)
Optimize it to: (i) find its critical value(s) and (ii) test whether the function is at relative
maximum or minimum.
TOTAL MARKS
[25]
Second Opportunity Question Paper
Page 4 of6
July 2024
 |
5 Page 5 |
▲back to top |

Mathematics for Agribusiness Management
QUESTION FOUR
a. Solve:
MTA 611S
[MARKS]
(2)
b. Solve:
fo1
(3x 3 - x + 1) dx
(3)
c. Solve:
f 12y 2 (y 3 + 2)dy
(5)
d. Suppose a Small Diary Enterprise faces fixed costs of N$4000 and marginal costs
represented by the function:
de
dq
= 250
+ 30q + 9q 2
(5)
where c is the total cost (in dollars) of producing q kilograms of product. Find the cost
of producing 100 kilograms of the product.
e. To meet an order for weaners, a farmer wishes to distribute his production between
two farms, farm 1 and farm 2. The total-cost function, c(q 1, q2 ), for the weaner
production is:
c(q1, qz) = q"f+ 3q1 + 25q 2 + 1000
(10)
where q1 and q2 are the numbers of weaners produced at farm 1 and farm 2,
respectively. How should the farmer distribute his weaner production across the two
farms to minimize production costs? {Hint: use the Langrage approach with the
constraint being: q1 + q2 = 100).
TOTAL MARKS
[25)
THE END
Second Opportunity Question Paper
Page 5 of6
July 2024
 |
6 Page 6 |
▲back to top |
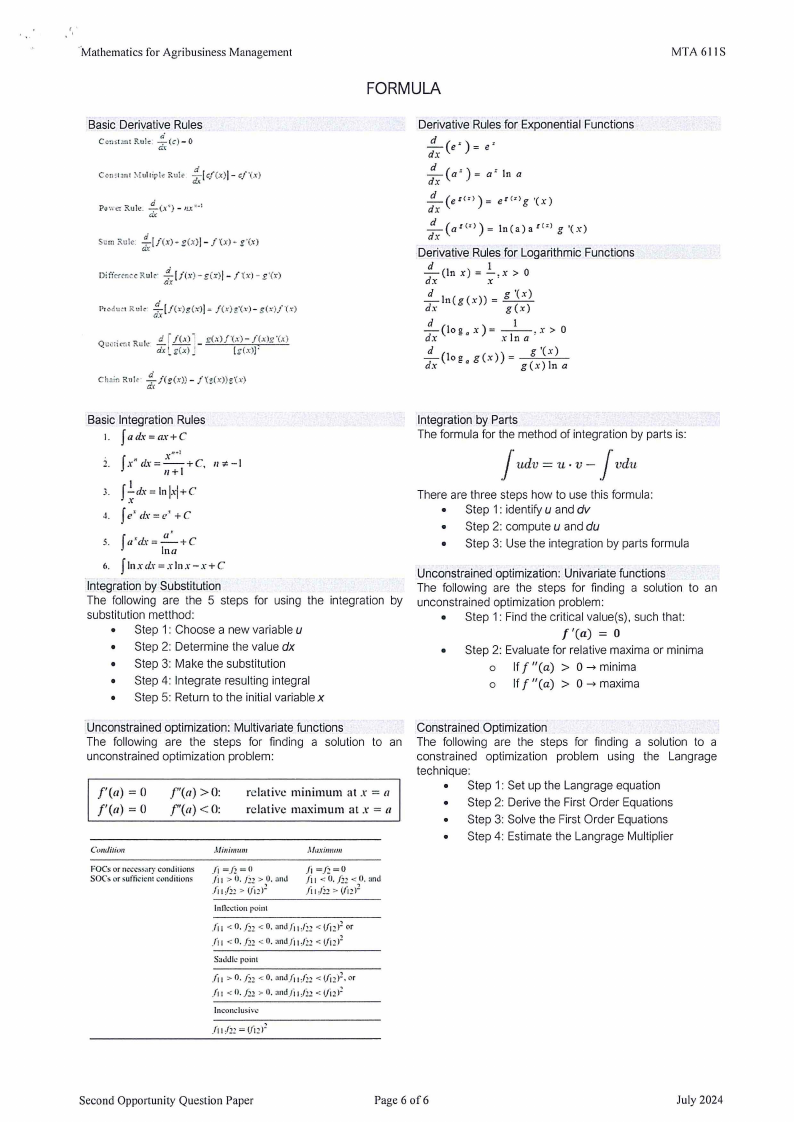
'Mathematics for Agribusiness Management
MTA 61 IS
FORMULA
Basic Derivative Rules
Constant Rule. _![_(c)- 0
~\\"
Con~IJnt ~iullipt~Rule f[cf(:c)J- cf'(x)
~I Sum Ruic. j(x)• z(x)J- f Xx)·· g ·(x)
ctx
Dificrtncc Ruic· ~Jj(x)-
ax
s(x)J - f Xx) - e'(x)
Produ-t Ruk 4a-x[J(:r)g(x)j
/(x) g'(x)- g(x)f'(x)
Quc:i,-n1 Rule .'!../.(..,)j_ g(x)f'(x)- j(x)g '(.<)
J dx _ 2(x)
[g(x)]·
Chain Ruic· f(g(x)) - f \\'.e(x))e\\'.x)
r.,:
Derivative Rules for Exponential Functions
:"(e=)=e=
!!_(a')= a= In a
dx
.!!._(e'',i)= erCr>g '(x)
dx
!!.._(a'''l) = ln(a)a ,er> g '(x)
dx
Derivative Rules for Logarithmic Functions
-(dlnx)=-,x>O 1
dx
x
-ldn(g(x))
dx
= _"o_'·(_x)
g(x)
-d(log
dx
-(dlog
dx
0 x)=
--,xI
x In a
>0
g(x)) = g "(x· )
0
g(x)ln a
Basic Integration Rules
f I. alfr=ax+C
fn
x•·•
1. X lfr=--+C,
11+1
11#-I
3. f-!_{l\\=- In1-+'l C
fX
4. ,t' clx= e·' + C
5. a'dx=-+Ca'
f Ina
f 6. lnxclr=xlnx-x+C
Integration by Substitution
The following are the 5 steps for using the integration by
substitution metthod:
• Step 1: Choose a new variable u
• Step 2: Determine the value dx
• Step 3: Make the substitution
• Step 4: Integrate resulting integral
• Step 5: Return to the initial variable x
Integration by Parts
The formula for the method of integration by parts is:
.l .l = 1.tdv 'lL • v -
vd1.1.
There are three steps how to use this formula:
• Step 1: identify u and dv
• Step 2: compute u and du
• Step 3: Use the integration by parts formula
Unconstrained optimization: Univariate functions
The following are the steps for finding a solution to an
unconstrained optimization problem:
• Step 1: Find the critical value(s), such that:
t '(a) = o
• Step 2: Evaluate for relative maxima or minima
o If f "(a) > O-> minima
o If f "(a) > O-> maxima
·Unconstrained optimization: Multivariate functions
The following are the steps for finding a solution to an
unconstrained optimization problem:
f'(a) = 0
f'(a) = 0
f'(a)>O:
f'(a) < 0:
relative minimum at x = a
relative maximum at x = a
Conc/i1iv11
Minimum
FOCs or ncccssar.· co11Ji1ions
SOCs or sufficicni conditions
/1 =h = 0
Ji1 > 0. /~2 > 0. ::rnJ
/11/-22> ((12/
/1=h=0
/1 I < 0. /12 < 0. and
/11 J!2 > (fi111
lnncclion point
/11 < 0. /21 < 0, ond/i1,/~1 < if121 2 or
/11 < 0, /21 < 0, and/11 J!1 < (!'i2J2
SaJdlc poinl
/11 > 0. h1 < 0, and/11J12 < (fi1) 1• or
/1 I < o.h1 > ll, ;111dJI1/22 < Vi2) 2
lnconclusiV<.·
ConstrainedOptimization
The following are the steps for finding a solution to a
constrained optimization problem using the Langrage
technique:
• Step 1: Set up the Langrage equation
• Step 2: Derive the First Order Equations
• Step 3: Solve the First Order Equations
• Step 4: Estimate the Langrage Multiplier
Second Opportunity Question Paper
Page 6 of6
July 2024





