 |
ETP720S - Experimental Techniques for Process Engineers 324 - 1st Opp. Nov 2022 |
 |
1 Pages 1-10 |
▲back to top |
 |
1.1 Page 1 |
▲back to top |

 |
1.2 Page 2 |
▲back to top |

 |
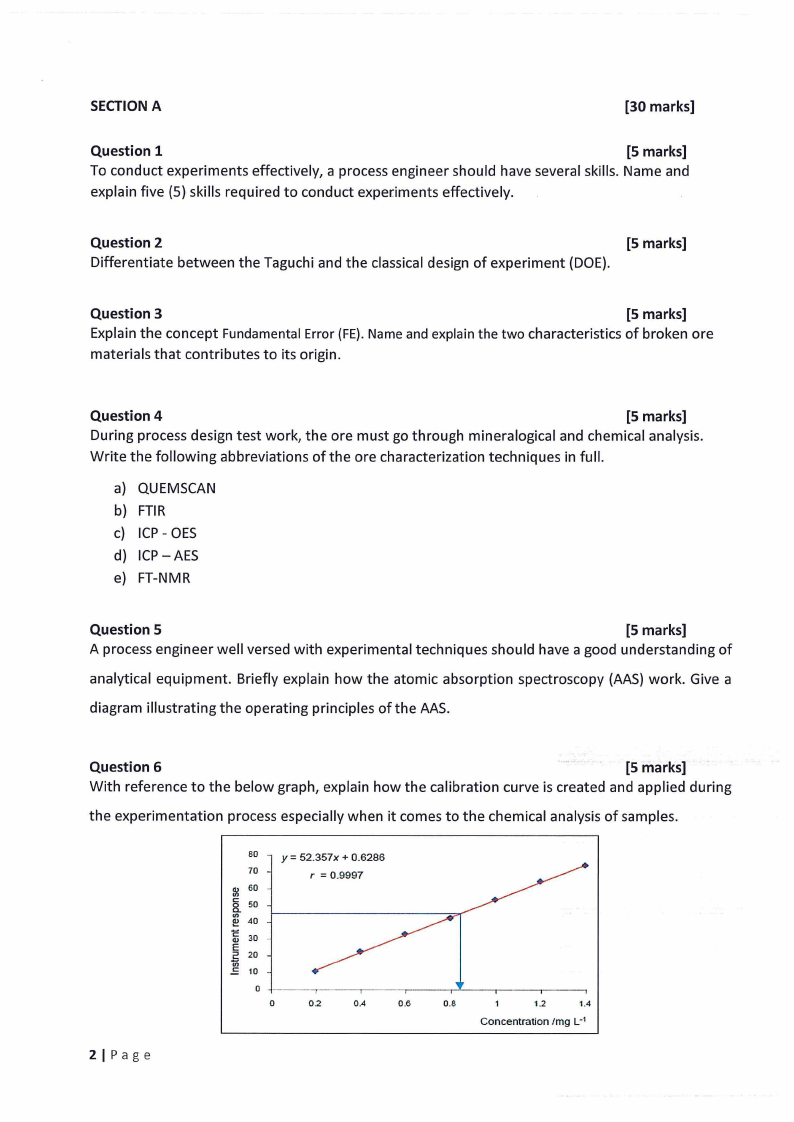
1.3 Page 3 |
▲back to top |
 |
1.4 Page 4 |
▲back to top |

 |
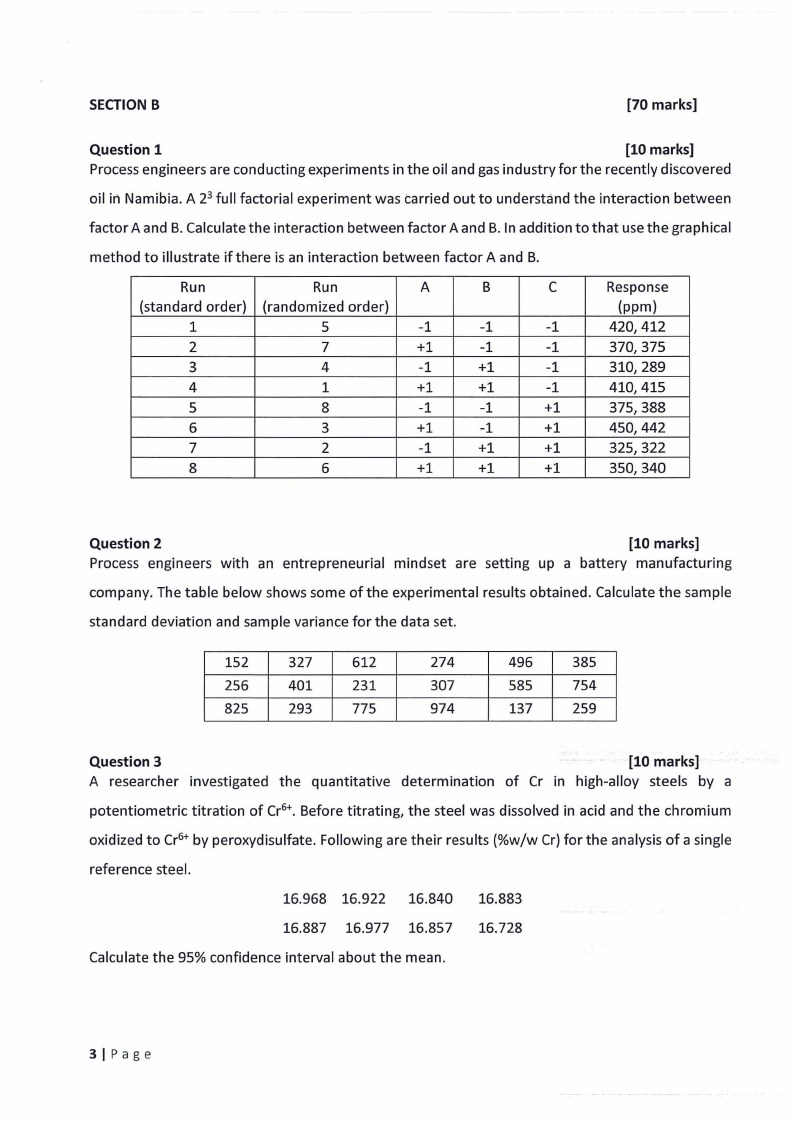
1.5 Page 5 |
▲back to top |
 |
1.6 Page 6 |
▲back to top |

 |
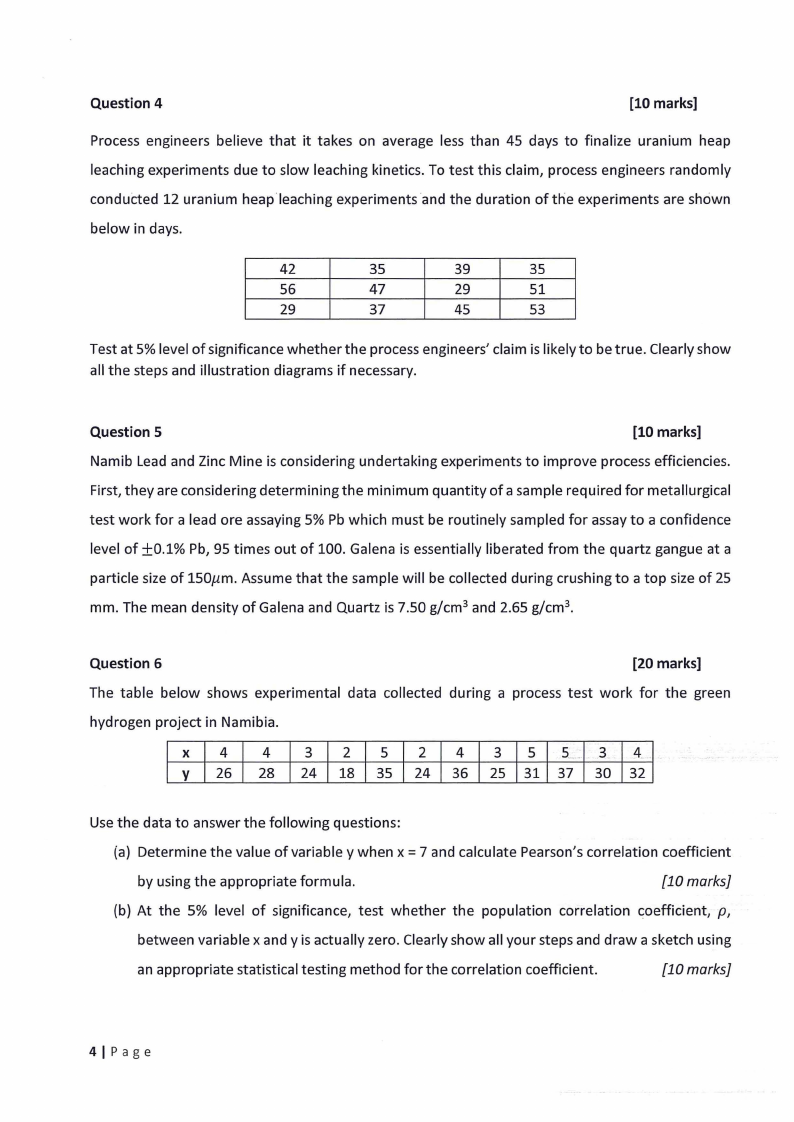
1.7 Page 7 |
▲back to top |
 |
1.8 Page 8 |
▲back to top |

 |
1.9 Page 9 |
▲back to top |

 |
1.10 Page 10 |
▲back to top |

 |
2 Pages 11-20 |
▲back to top |
 |
2.1 Page 11 |
▲back to top |
 |
2.2 Page 12 |
▲back to top |

 |
2.3 Page 13 |
▲back to top |
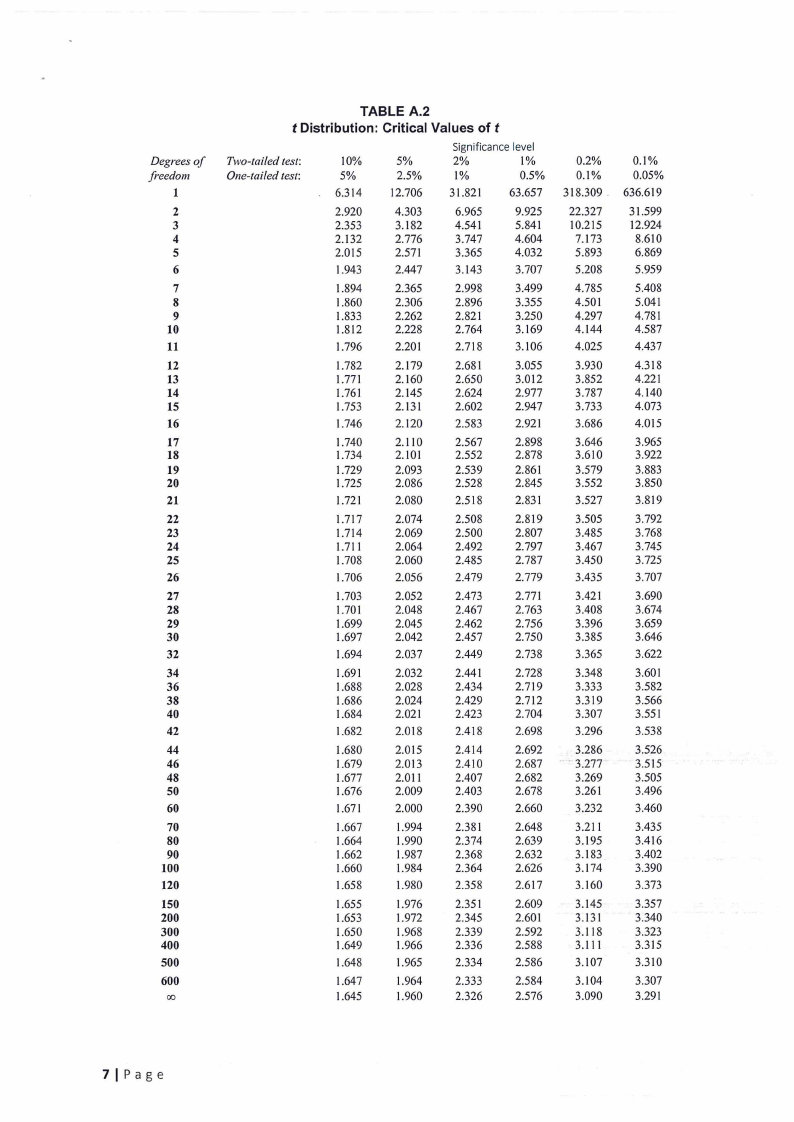
 |
2.4 Page 14 |
▲back to top |

 |
2.5 Page 15 |
▲back to top |
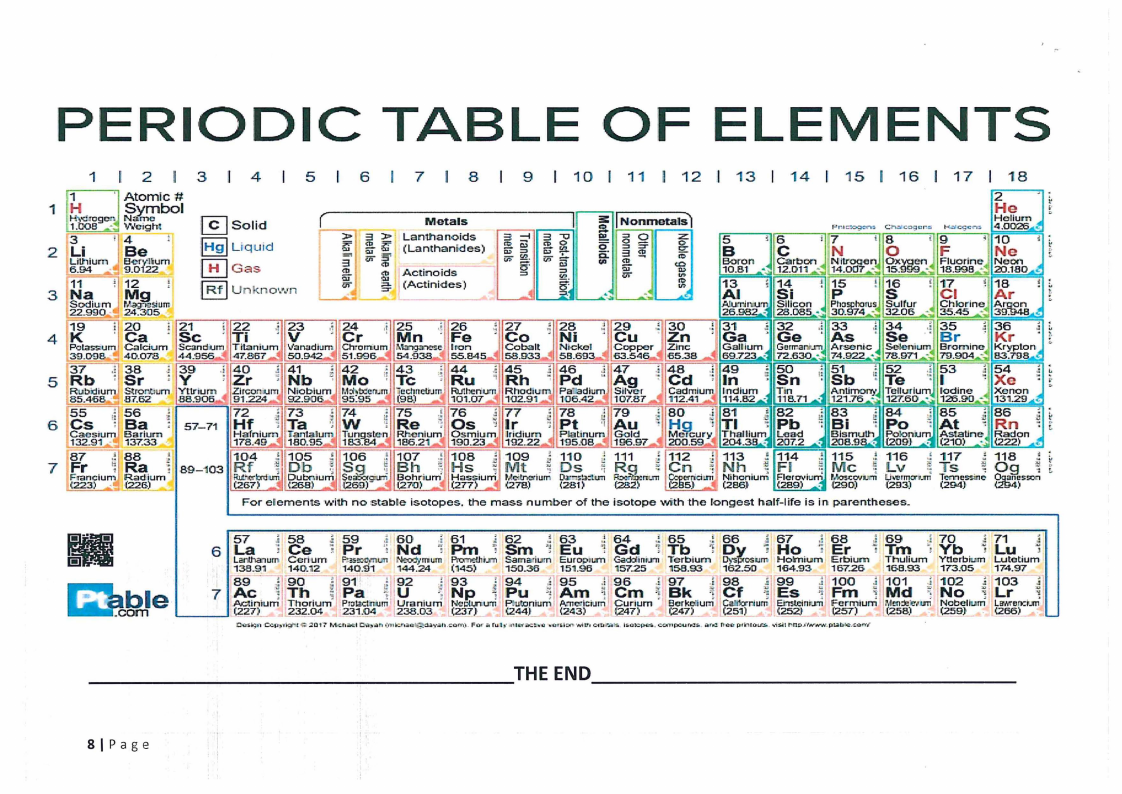
 |
2.6 Page 16 |
▲back to top |






