 |
LIA601S - LINEAR ALGEBRA 2 - 2ND OPP - JAN 2023 |
 |
1 Page 1 |
▲back to top |

(
n Am I BI A u nl VE Rs ITV
OF SCIEnCE Ano
FACULTY OF HEALTH, APPLIED SCIENCES AND NATURAL RESOURCES
DEPARTMENT OF MATHEMATICS AND STATISTICS
QUALIFICATION: Bachelor of Science in Applied Mathematics and Statistics
QUALIFICATION CODE: 07BSOC; 07BSAM
LEVEL: 6
COURSE CODE: LIA601S
SESSION: JANUARY 2023
COURSE NAME: LINEAR ALGEBRA 2
PAPER: THEORY
DURATION: 3 HOURS
MARKS: 100
SUPPLEMENTARY/
EXAMINER
MODERATOR:
SECOND OPPORTUNITY EXAMINATION
DR. NEGA CHERE
DR. DAVID IIYAMBO
PAPER
INSTRUCTIONS
1. Answer ALL the questions in the booklet provided.
2. Show clearly all the steps used in the calculations.
3. All written work must be done in blue or black ink and sketches must
be done in pencil.
PERMISSIBLE MATERIALS
1. Non-programmable calculator without a cover.
THIS QUESTION PAPER CONSISTS OF 3 PAGES {Including this front page)
 |
2 Page 2 |
▲back to top |

QUESTION 1 [36]
Let V and W be vector spaces over a filed lit and T: V W be a mapping.
1.1. State what does it means to say Tis linear.
[3]
([rn 12. LetT: ~ 3 ~ 3 be defined by T
= [:;]
(a) Show that Tis linear.
[14]
(b) Find the matrix of T with respect to the standard basis of 1I3t •
[7]
(c) Use the result in (b) to find the Characteristic polynomial of T.
[5]
1.3. Let T: 1I3t -+ 1I2t be given by T(x, y, z) = (lxl, y + z). Determine whether Tis linear on not.
[7]
QUESTION 2 [23]
2.1. Let 'B = {vi, v2 } and C = {ui, u2 } be bases for a vector space V and suppose
(a) Find the change of coordinate matrix from 'B to C.
[S]
= (b) Use part (a) to find [x]c for x -3v 1 + 2v2 .
[S]
2.2. In P2, find the change-of-coordinates matrix from the basis
= = 'B {1- 2t + t 2 , 3 + 4t 2 , 2t + 3t 2 } to the standard basis S {1, t, t 2 }.
[S]
= 2.3. Let 'B {vi, v2 , v3 } be a basis of 1I3t in which v1 = (1, 1, O), v2 = (O, 1, 2) and
= = v 3 (1, 0, -1). Find the coordinate vector of v (1, 2, 3) with respect to the basis 'B. [8]
QUESTION 3 [8]
Let A = PDP- 1 where P = [~ ;] and D = [~ ~].Then Compute A10 .
QUESTION 4 [10]
Find the quadratic form q(X) that corresponds to the symmetric matrix
i ~]-
[10]
3 -2
1
 |
3 Page 3 |
▲back to top |
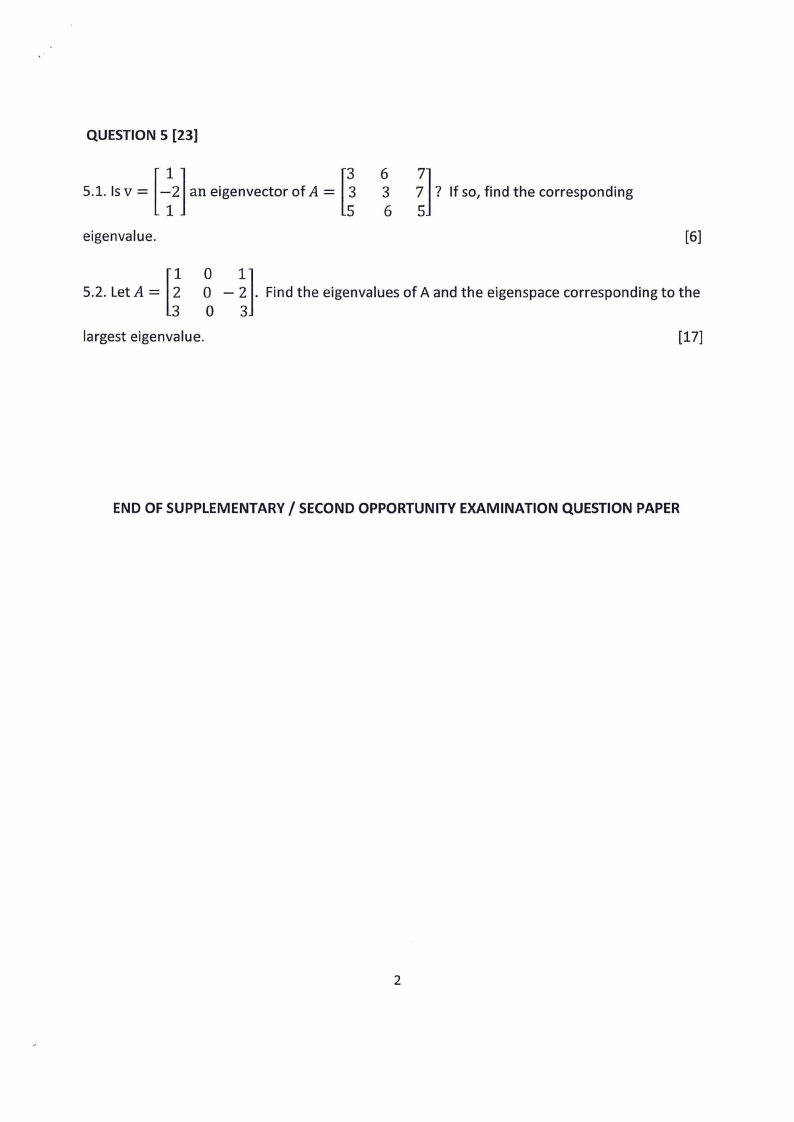
QUESTION 5 [23)
[! 6
=[ = 5.1. Is v ~2] an eigenvector of A
3
6
rl?If so, find the corresponding
eigenvalue.
[6]
[i= 5.2. Let A
-ilFind the eigenvalues of A and the eigenspace corresponding to the
largest eigenvalue.
(17]
END OF SUPPLEMENTARY/ SECOND OPPORTUNITY EXAMINATION QUESTION PAPER
2





