 |
QCM701S - QUANTUM CHEMISTR AND SPECTROSCOPY - 2ND OPP - JULY 2023 |
 |
1 Page 1 |
▲back to top |
nAm I BI A un IVE RSITY
OF SCIEnCE Ano TECHnOLOGY
FACULTYOF HEALTH,NATURALRESOURCESAND APPLIEDSCIENCES
SCHOOLOF NATURALAND APPLIEDSCIENCES
DEPARTMENTOF BIOLOGY,CHEMISTRYAND PHYSICS
QUALIFICATION:BACHELOROF SCIENCE
QUALIFICATION CODE: 07BOSC
COURSENAME: QUANTUM
CHEMISTRYAND SPECTROSCOPY
LEVEL:7
COURSECODE:QCM701S
SESSION:JUNE/JULY 2023
PAPER:THEORY
DURATION: 3 HOURS
MARKS: 100
SUPPLEMENTARY/SECONDOPPORTUNITYEXAMINATION QUESTION PAPER
EXAMINER(S) Prof Habauka M Kwaambwa
MODERATOR: Prof Edet F Archibong
INSTRUCTIONS
1. Answer ALL the SIX questions
2. Write clearly and neatly
3. Number the answers clearly
4. All written work must be done in blue or black ink
5. No books, notes and other additional aids are allowed
6. Mark all answers clearly with their respective question numbers
PERMISSIBLEMATERIALS
Non-programmable Calculators
ATTACHMENT
List of Useful Constants
THIS QUESTION PAPERCONSISTSOF 5 PAGES(Including this front page and list of useful
constants as an attachment)
1
 |
2 Page 2 |
▲back to top |

QUESTION 1
[16]
(a) State briefly what is meant by blackbody radiation. Show graphically the effect of
temperature a typical wavelength distribution curve of the emitted blackbody
radiation.
(5)
(b) Explain briefly quantisation of energy, particle-wave duality and degeneracy as used
in quantum mechanics.
(3)
(c) The photoelectric effect experiment demonstrates that light has particle-like
properties. What is the effect of increasing (i) the frequency of incident light and (ii)
intensity of the incident light.
(4)
(d) Calculate the energy of photon and an electron when each has a wavelength of 1 A
and comment on the relative magnitude of your answers.
(4)
QUESTION 2
[21]
(a) Investigate whether the function y(x) = Acosx + Bsinx (where A and Bare constants) is
a solution to the differential equation:
d2y\\x) +y(x)=O
(4)
dx-
(b) Explain using mathematical expressions what you understand by the following terms
as used in quantum mechanics:
(6)
(i)
Linear operators
(ii) Normalised wavefunction
(iii) Expectation value
(c) The normalised wavefunction for a particle-in-a-box is of the form
I
tr=(¾Y si{n;x} forO x a
Calculate the probability that a particle in a one-dimensional box of length a is found
to be between O and a/2.
• (5)
f Note: sin2kxdx =f(½(1-cos2kx)}x
(d) Using the wavefunction in (c) above, sketch e variations of tr(n =4) and tr 2(n =4) in
the range O x a. At what values of x in terms of a is tr(n = 4) = 0 in the range
0 X < a.
(6)
2
 |
3 Page 3 |
▲back to top |
QUESTION 3
[10]
(a) If <IA> and <IA> are real normalised and orthogonal atomic orbitals belonging to A and
B, respectively, show that the molecular orbital of their linear combination below is
also normalised.
(5)
/\\ 2
(b) Using the Quantum theory rules, construct the operators T .
(5)
QUESTION 4
. [10]
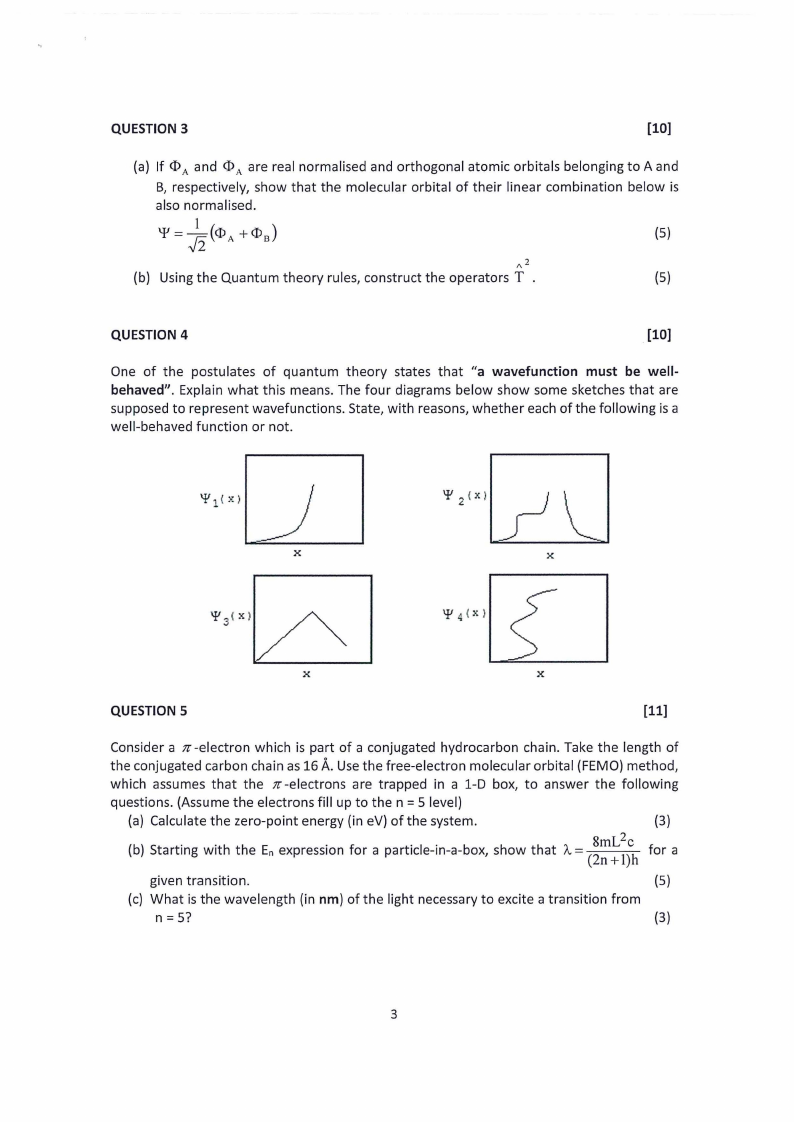
One of the postulates of quantum theory states that "a wavefunction must be well-
behaved". Explain what this means. The four diagrams below show some sketches that are
supposed to represent wavefunctions. State, with reasons, whether each of the following is a
well-behaved function or not.
_J
X
X
X
X
QUESTION 5
[11]
Consider a tr-electron which is part of a conjugated hydrocarbon chain. Take the length of
the conjugated carbon chain as 16 A.Use the free-electron molecular orbital (FEMO) method,
which assumes that the tr-electrons are trapped in a 1-D box, to answer the following
questions. (Assume the electrons fill up to then= 5 level)
(a) Calculate the zero-point energy (in eV) of the system.
(3)
(b) Starting with the En expression for a particle-in-a-box, show that 11=, (~::
for a
given transition.
(5)
(c) What is the wavelength (in nm) of the light necessary to excite a transition from
n=5?
(3)
3
 |
4 Page 4 |
▲back to top |

QUESTION 6
[15]
(a) Which of the following molecules will possess a (i) rotational microwave spectrum,
and; (ii) vibrational (infrared) spectrum: N2,IBr, CS2,CH3CI?Give brief reasons for your
answers.
(5)
(b) The allowed rotational energy levels of a rigid diatomic molecule are given by:
h2
EJ=-, J(J+ 1)
81t-1
(i) State what all the symbols in this equation represent.
(2)
(ii) What is the selection rule for the rotational energy transitions and hence show
that the separation between the successive spectral absorption lines is always
2B, where Bis the rotational constant.
(4)
(iii) The rotational constant of 1H35CI (hydrogen chloride) is greater than of 2035CI
(deuterium chloride). Explain, with reasons, this statement.
(4)
QUESTION 7
[17]
After a freaky accident in the lab only a small part at the centre of the ro-vibrational spectrum
of 1H127/, with peaks at 2296.40, 2322.60 and 2335.70 cm-1, was recovered. From the
recovered data of the spectrum:
(a) Assign the transitions to each of the peaks.
(6)
(b) Calculate the bond length.
(7)
(c) Calculate the force constant.
(4)
Atomic masses (amu):
127/ = 126.90
END OF EXAM QUESTIONS
4
 |
5 Page 5 |
▲back to top |

USEFULCONSTANTS:
Universal Gas constant
Boltzmann's constant,
Planck's constant
Debye-Huckel's constant,
Faraday's constant
Mass of electron
Velocity of light
Avogadro's constant
1 electron volt {eV}
R=
k=
h=
A=
F=
me =
C
=
NA =
=
8.314 J K·1 mol·1
1.381 X 10-23 J K·1
6.626 X 10-34 J S
0.509 {mol dm·3) 112 or mo1·05 kg0-5
96485 C mo1·1
9.109 X 10-31 kg
2.998 x 108 m s·1
6.022 X 1023
1.602 X 10-19 J
5





