FAS411S - FUNDAMENTALS OF AGRICULTURAL STATISTICS - 1ST OPP - JUNE 2024 :: NUST past examination papers between 2020 and 2024

Expand document

Collapse document

FAS411S - FUNDAMENTALS OF AGRICULTURAL STATISTICS - 1ST OPP - JUNE 2024

1 Pages 1-10

1.1 Page 1

1.2 Page 2

1.3 Page 3

1.4 Page 4

1.5 Page 5

1.6 Page 6

1.7 Page 7

1.8 Page 8

1.9 Page 9

1.10 Page 10

2 Pages 11-20

2.1 Page 11

FAS411S - FUNDAMENTALS OF AGRICULTURAL STATISTICS - 1ST OPP - JUNE 2024

1 Pages 1-10

▲back to top

1.1 Page 1

▲back to top

(

nAmlBIA unlVERSITY

OF SCIEnCE Ano TECHn OLOGY

FACULTY OF COMMERCE, HUMAN SCIENCES AND EDUCATION

DEPARTMENT OF TECHNICAL AND VOCATIONAL EDUCATION AND TRAINING (TVET)

QUALIFICATION : NUST BRIDGING PROGRAMME -TVET AGRICULTURE

SPECIALISATION

QUALIFICATION CODE: 04NBTA

LEVEL: 4

COURSE CODE: FAS411S

COURSE NAME: FUNDAMENTALS

OF AGRICULTURAL STATISTICS

SESSION: JUNE 2024

DURATION: 3 HOURS

PAPER: 1

MARKS: 100

FIRST OPPORTUNITY EXAMINATION QUESTION PAPER

EXAMINER(S) Ms. E MATALI

MODERATOR: Mr. M Lubinda (Internal)

INSTRUCTIONS

1. For SECTION A write down the letter corresponding to. the best option for each

question

2. For SECTION B show clearly all your work

3. All written work MUST be done in blue or black ink

ATTACHMENT: Formula sheet, t-table, z-table, chi-square table

PERMISSIBLE MATERIALS: 1. Non-Programmable Calculator without the cover

THIS QUESTION PAPER CONSISTS OF _11_ PAGES (Including this front page)

1.2 Page 2

▲back to top

SECTION A

QUESTION 1

[20 marks]

Write down the letter corresponding to your choice next to the question number.

1.1. What does Central Limit Theorem state about the distribution of sample means?

a) It is normally distributed regardless of the population distribution

b) It is always skewed to the right

c) It is not affected by sample size

d) It is determined solely by the population standard deviation

(2)

1.2. Primary data is a type of data that is collected by researchers directly from main

sources through interviews, surveys or experiments.

a) True

b) False

(2)

1.3. A confidence interval estimate for the population mean is constructed using:

a) Sample standard deviation

b) Population standard deviation

c) Both sample and population standard deviation

d) Mean deviation

(2)

1.4. In hypothesis testing, the alternative hypothesis is always denoted by:

a) Ho

b) H1

c) Ha

d) H2

(2)

1.5. Which distribution is used when dealing with binary data?

a) Normal distribution

b) Binomial distribution

c) Poisson distribution

d) Exponential distribution

(2)

1.3 Page 3

▲back to top

1.6. If event A and event B cannot occur at the same time, then events A and Bare said

to be:

a) Mutually exclusive

b) Statistically dependent

c) Joint events

d) Collectively exhaustive

(2)

1.7. The coefficient of determination measures:

a) The strength of the relationship between two variables

b) The slope of the regression line

c) The residual variance

d) The standard deviation

(2)

1.8. Which sampling method involves dividing the population into groups and randomly

selecting groups to sample?

a) Simple random sampling

b) Stratified random sampling

c) Systematic sampling

d) Convenience sampling

(2)

1.9. What is the formula to calculate the coefficient of variation (write out)?

= __ a) CV

A_lp_h__a(a_)_

Sample Mean (x)

= b) CV Standard Deviation (s)

Sample Mean (x)

= c) CV Standard Deviation (s)

Alpha (a)

d) CV =

Alpha (a)

Standard Deviation (s)

(2)

1.10. All the events in the sample space that are not part of the specified event are called:

a) Simple events

b) The sample spaces

c) Joint events

d) The complement of the event

(2)

1.4 Page 4

▲back to top

SECTION B

QUESTION 2

2.1.

[26 marks]

[8]

2.1.1. Using classes 20 to less than 30, 30 to less than 40, 40 to less than 50..., construct

frequency distribution table for the data. NB your frequency distribution table must include;

less than cumulative frequency, percentage frequency and class midpoint. As part of their

national crusade to eradicate unemployment in Namibia. Meatco management recently

employed some workers with the following ages.

25 32 21 60 22 30 42 45 50 50 36 25 32 21

43 52 64 40 44 40 55 48 46 59 60 43 52 64

(5)

2.1.2. Plot a histogram using the data of your frequency distribution table.

(3)

2.2. Over the course of 3 hours 55 batches of harvested crops arrive at a processing facility.

The processing facility has been recording the delay in the arrival of each batch, measured in

minutes. The number of minutes they were late is shown in the grouped frequency table

below.

[18]

Minutes late

Frequency

0-10

10-20

20-30

30-40

40-50

50-60

2.1.1. Estimate the mean late arrival time for the batches of crop

(9)

2.1.2. Estimate the variance and the standard deviation for late arrival time for the batches of

crop

(9)

1.5 Page 5

▲back to top

Question 3

[28 marks]

3.1. Namib Mills has submitted bids on two separate government contracts A and 8. Namib

Mills feels that it has 60% chance of winning contract A and 40% chance of winning contract

8. Furthermore, it believes that winning contract A is independent of winning contract 8.

[12]

3.1.1. Draw a tree diagram to represent the above experiment and list the possible outcomes

(Hint: Use W to denote Win and F to denote Lose)

(4)

3.1.2. Write down the sample space.

(2)

3.1.3. What is the probability that Namib Mills will win both contracts?

(3)

3.1.4. What is the probability that Namib Mills will win at least 1 of the contracts

(3)

3.2. Five hundred farmworkers were selected from various agricultural enterprises within the

Otjozondjupa region. The farmworkers were asked whether or not they have any retirement

benefits provided as part of their employment package. The table below summarises the

responses collected from the farmworkers.

[16]

Have Benefits

Have No Benefits

Men

225

Women

150

If one employee is selected at random from these 500 employees, what is the probability (P):

3.2.1. P(women)

(2)

3.2.2. P (has retirement benefits)

(3)

3.2.3. P (has retirement benefits given the employee is a men)

(3)

3.2.4. P (women and has benefits)

(3)

3.2.5. P (women or has benefits)

(3)

3.2.6. Are the events "have benefits" and "have no benefits" mutually exclusive? Why or why

not?

(2)

1.6 Page 6

▲back to top

Question 4

[26 marks]

4.1. A factory has a machine that dispenses 80 ml of fluid in a bottle. An employee believes

the average amount of fluid is not 80 ml. Using 40 samples, he measures the average amount

dispensed by the machine to be 78 ml with a standard deviation of 2.5.

[10]

(a) State the null and alternative hypothesis.

(2)

(b) At a 95% confidence level, is there enough evidence to support the idea that the machine

is not working property?

(8)

4.2. Find the Quartile Deviation for the following observations on number of Mesta plants in

10 equi-sized plots: 13, 9, 16, 4, 8, 19, 7, 23, 21, 12

[10]

4.3. The sample variance for ages (in years) of a sample of 11 mopane trees in the northern

part of Namibia, was estimated at 10.27 years. The interval estimation for the variance of the

entire population of all mopane trees with a 90% degree of confidence is

[6]

END OF PAPER [100]

1.7 Page 7

▲back to top

FORMULASHEET

ctO.Sn-CFJ

i tf.r

Z=-r

1-JJ

S(X) = LX{P1

= b nl:.x;y-t:x,ty

itt,-: 1 -0: i

s2 l=~;1,-R)l /1

n-1

1.8 Page 8

▲back to top

2-Table

The table shows cumulative probabilities for the standard normal curve.

Cumulative probabilities for NEGATIVEz-values are shown first. SCROLLDOWN to the 2nd

page for POSITIVEz

,. •z lo'• 1,;:•::90·,·-~f.01 ,, .• 02' r .03 r. ~04 ' ', ..-.05.~· ~Q6'' ,~~-07 '".08 1-J{.09 .

~ooos ,t

-~' ·

i~:r

-3A

-3.l

-32

>!0003. .0003 ,.0003

.QOOS \\,0005

::0001 J'J¥)7- ) ':()006

.0003 .0003 .0003

...0o0o0o4 s

.0004

.0006

.0004

.•0006.

·~~1. ''; .0010 . ..ooo9 '.0009 .9()® ·. j)()l)8 • .O(q

,0003

.•0003..

1·

.0003

.0004 .0004 .0004

,0006 .0005 . .0005

10008 .0000 .00()1

.0002

.0003

.0005

.«m

-3.0

" . -2.9

J -.2z.8.r

-~: -~•~-' ..0013 -.0013. .Q0-13 · .00t2,· :.0012 .0011 I ,0011

.()919. .0()t8· .

J,017 <+ .0016 ·.0016 .0016

,..0026,..,

L< .0024 .0023· .O(l23• .0022 ~,.oo21

.C)036· .op34 .. 0033, .;:0032. ·:.0031, .0030 .0029..

,0011,

.0015

.0021

.0028

.U>10 · .0010·

.0014 .0014

.0020 .0019

.0021 .0026-

! ' -2.6 ri, \\0047- .,0045, .. 0044. .()043 .0041 .~. o . •.O!JFl. 9 .0038 JXl37 .0036

s -2.5 ..0062.,, .0060 \\0059 .0057 .0055 .00&1 .0052 .0()51 .0049 .0048

-2.4 .0062. .0080 0078 .0075 .()073 .0071 .0069 .0068 .0066 .0064

i -2.3 .0107 .0104 .0102 .0099 .0096 .0094 .0091 .0089 .0087 .0064

-2.2 .0139 , .0136 .0132 .0129 .0125 ~122 .011i r .0116 .0113 .0110

·1 -2.1

. -2.0

-1.9

.0'179 ',0174 I .0170 .Ol66 .0162 .0168 .0164 ·.OrlSO .0146· .0143

.0228_,.• 0222 .0217. ,0212 .02Q1 .0202 ,0197 .0192 .0188 .0183

.0287 .0281'' .0274 .0268 .0262 .0256' ;0250 .0244 .0239 .0233

-1.8 .0369 ' .0351 ·.0344. .D336 .0329 .0322. :0314 ;0307 .0301 .0294

t .1.1 ·

-1.6

i -1.5

tf• -1.4'

j -1.3

...I,'• ' .1.2

'.' -M ..

-1.0 .

.O!W6

.0548

.0668

-~.0808

.1151

.1357'

.1687.

.O.S36 .0427 .0418 .0409 .J>40I

.0537 .0526 .OS16 .osos .0495

.0655 .0643 "'.0630 .()618 .0606

.0793 .07J8 ,0764 .o749 .0735

.0951 .0934 . .0918 .0901• 10885

.1131 .1112 ;t093 .1075 .1056

.1335~ ,.1314 .1292 : .1271 . ;1261

.1562 .1539 .1515 .1492 .1469

.0392 .0384- ,0375

.0485 .0475,. .0465

;0721

,.'·.g582

.oroe

.<b,1

.0694

.0367

.0456

.0559

.0681

.0869 ,0853-,, .0838 .0823

.1038 .1020 · .1003 .0985

.1i30 .1210 .1190 .1170

.l<WS .1423 •1'401' ,1319

.0.9 .184t

.o.a · .2119

.0.7·' .2420

-.1814

.2090

.2389

.17~

-~1

2358

.1762

2033

.2327

,.0o.5.~•

'.2743

.ao85,,

.2709

.3050

., .2676 1•.2643

.3015 .2981.:

rId . .O.A .3446 .3409 .33n i• ~3336

I• .0.3 .382.1 ,3783 .3745 ,•. 3707

.1736 .1711

.2005 .19n

.2296 ,2266

.2611 .2578

.29-16. .2912

.3300 .

i,~

.3669 .3632

.1685

.1949

.2236

:JB71

.3228

. .3694

.1660

.1922

.2206

.251•

.2843

. .3192

.3557

.1635

.1894

.2177

.2483

.2810

.3156

.3620

.1611

.1867

.2148

.2451

.3121

.3483

'! ~.2 .4207 .4168 .4129 .4090 .4052 .4013 .3974 .3936 .'J1?Hl .3859

:0.1

.,..' 0.0

.4602 AS&2 ,4522 .4483 .4443 .4404 ,'4364 .4325 .4286 .4247

.5000 .4960 ,4920· ,488() .4840 .4$01 .4761 ,4721 .4681 .4641

1.9 Page 9

▲back to top

Cumulative probabilities for POSITIVEz-values are shown below.

! -~.z.~"d't

0.0 <.

I 0.1"'

';00.,

.5000

.5398

· .01 .J>2,

.5040 -~

~5438 .5478

i 0.2

.5793 .5832 .5871

0.3

' 0.4 ..

0.5

.6119 .621_7 .6250

.6554 -~L ,' .6628

.6915 .6950 .6985

0.6 .7251 .7291 .7324

('.; 0.1

. 0.8

~7580 .7611 .7642

.7:8&1 .7910 .7939

0.9 '.8159 :8186 .a.212

i 1.0 .8413 .8438 .8461

·t 1.1 , .8643 ,8665 .8686

r 1.2

.8849. .8869 .8888

1.3 .9032 .9049 .9066

1.4 .9192 .'1207 .9222

. 1.5 -,.9332

:' 1.6 . .9452

.. 9345

.9463

~9357

~9474

ft 1,1· .9564 .9564· .9573

l'f 1,8

t" 1.9

p 2.0

.9641- ·~.9649

.9713 .9719

.9772 .~78

,9656

.9726

.9783

2.1 .9821 .9826 .9830

(...

2.2

2.3

.9861 .9864 .9868

.9893 .9896 .9898

~1 2A

.99(8 .9920 .9922

~5 .9936 .9940 .9941

f 2.6 .9953 .9955 ,9956

J_ 2.7

2.8

.9965 .9966 '.9967-

.9974 .9975 .99!6

I 2.9

.9981 .9982 .9982

3.0 !J98"T .9981 .9987

. 3.1 .9990" .9991 .999f

;'•" 32 ~

L1, - 3.3

{__ J.4

.9993

.9995

.9997

.9993

.ms

.9997

.9994

.9995

.9997

~03

.04

i.05

.06 · i

.5.120 .5160 .5199 · .5239

.5517 5557 ,5696 .6636

.5910 .5948 .5987 .6026

.6293 .6331 .6368 .6406

.6664 .6700. '»736 .6772

.7019 .7054 .7088 .7123

.7357 .7389 .7422 .7454

.7673 .7704 .7734 ,7164

.7967 .7995 .8023 I~ .8051~

.8238 .8264 .8289 .8315

.8485 .8508 .8531 .8554

.8708 .8729 .8749 .8770

.8907 1, .8925 .8944 .8962

-~ .9082 .®99 .9115 .9131

.9236 .925t

.9279

.9370 .9382 .9394 .9406

.9484 I• ,~95 .9505 -~15

.9582 '.9591 .9599\\ .9608

.9664 .9671 .9678 .9686

.9732 .9738 .9744 .9750

.9788 .9793 .9798 .9803

.9834 .9838 .9842 .9846

.9871 .9675 I• .9878 .9881

.9901 .9904 .9906 .9909

.9925

.9943

.9957

.9968

.9977

.9!af

.9945

-~.9959

.9977

.9929

.994~

.9960

.9970

.9978

-~.9931

.9961

.9971

.9979

.9983' .9984 .9984 .9985

.9988· .9988 .9989 .9989

.9991 .9992 .9992 .9992

.9994 .9994 .9994 .9994

-~ .9996 .S995 .999ti

.9997 .9997 .9997 .ffi7

.07

.5279

.5675

.6064

.6443

.6808

.7157

.74$

.7794

.8078

.8340

."8577

.8790

.8980 '

.9147

.9292

.9418

.9525

;9616

.9693'

.9766

.9808

.9850

.9884

.9911

.9932

.9949

.9962

.9972

.9979

.9985

.9989

.9992

.9995

.9996

.9997

.oa

.5319

.5714

.6103

.6480

.6844

.n90

.7517

.7823

.8106

.8366

.8599

.8810

,8$97

.9162

.9306

.9429

.9536

.9625

:9699

.9761

.9812

.9864

.9687

.9913

.9934

.9951

.996;3

.99,7~

.9980

.9986

.9990

.9993

.9995

.9996

.9997

.09

.5359

.5753

.6141

.6517

.6879

.1'22A

.7549

.7852

.8133

.8389

.8621

.8830

.9015

.9177

.9319

.9441

.9546

:9633

.9706

.97ol

.9817

.9857

.9890

.9916

.9936

.9952

.9964

.9974

.9981

.9986

.9990

.9993

.9995

.9'J97

.9998

1.10 Page 10

▲back to top

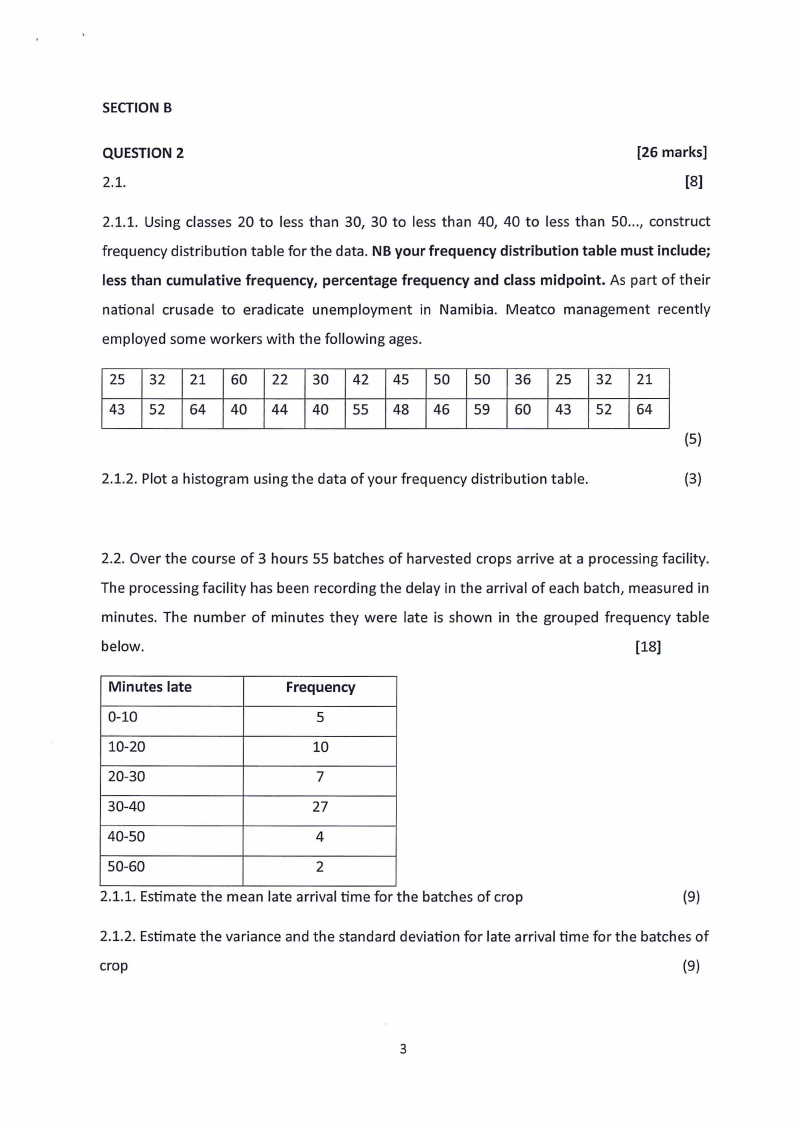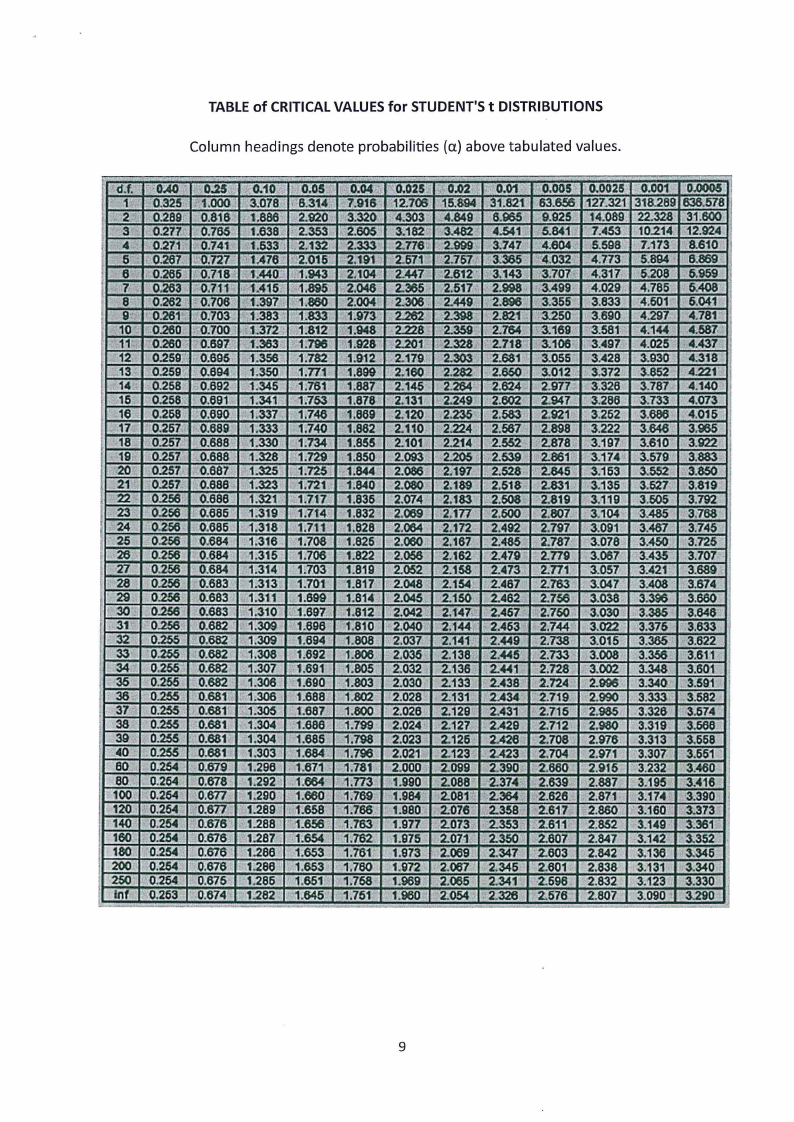
TABLEof CRITICALVALUESfor STUDENT'St DISTRIBUTIONS

Column headings denote probabilities (a) above tabulated values.

',cf.f. "'-·O.AO._,0.25 ··0.10. 1, o.os·:r o.o.r-.0.025 .,O.o2 O.G'I·· 0.005'>1,0.0025 ·0;001 ·"0Jl005'

1· "'0.325 ~f.000' 1 3.'078.J1i~1&.'314.7.916'• '12.708 .,.5.894 '31.82:1 83.85e 127.321 318.289 638..578

2 . 0.289( 0.818 1.886 r 2.920 3;320 4,303 '4.849 8.965 O.Q25r f4.089 22.32.8 31.600

3 0.27l 0.785 1.638 2.~- 2.605 3.182 3.482 4.541 6.841 7.453 "10214 12.92.4

,a.869 ,4 0.271 I 0,741 1.633, .'2,132· 2.333 2.778. 2.999 3:.747 4.804'. 5.598 V173 &610

6 <.Q.2.67 0,727 •1.476_ 2.016, 2,191 ,2.57L ·2.151 :3.365 4.032. 4.773 5.894~

r6 ..t . 0~266 • 0.718 1.440 1.943 1,2,104 2.MT· ,.2.612' 3.143-, 3i707 4.317 5.208 6.959

0.263 0,711 1.•415- i1,895 ; 2.048- . 2.365 2.517 2.998 3..499 4.029 4.785 &408

8 ''0.262 "0.706 7 :t.397 "'1.860 2;004 2.306 2.~9~ 2.896 "3.355 3.833 -4.601 6.041

9 -0.261~ 0,703 ·1.383 1.833 1'.973 2.262 • 2.398 2.821 .• -3.250, 3.690 4.297 4.781

10 0.290 . 0.100 . :1..372, 1.812 II UM8. 2.228 ~"

2.7&4 •3.169 3.58f 4,144 r4,5S7

'11( 0.260 "0,697 I', 1.363 1.1795 '..,,1.928 2.201 . 2.328 -2.718' 3.108 3.497 4.025 4.437

12 0.259,' 0,695. 1.35tL ,.,1;782. 1.&12.. 2.179. 2.30a ,.,_2,681 . 3.055 3.428 3.930, 4.318

.13, 0.258 0.894• 1.350 1.771 1.899 2.160 2.282 2.660, 3.-012 • 3,372 3-852 4.221'

14 .0.268 ,. 0.882 1.345 1.781, 1.887 2.146 2.264:.. I~ 2,ISZ4 2.977 3.328~ 3.787 4.140

16 0:268· 0.681 1.341 1:763. 1,878 2;131 2.249 2.602 2..947 3.286 3.733 4.07.3

18 · 0.268 _ 0.680 ·'1.337 1,746 1.869 2.120 i, 2.236 I, 2.683 2.921 S.252 3.680 4.015

17 .0.257 0.689 1.333 , 1.740 1.862 2.110 2.224 I 2.6&7 2.898 3.222'' 3.646 3.965

18 0.257 0.688_ 1.S30 1.734' 1.!65 , 2.101· 2.214 •2.!62 2.878 • 3.197 3.610 3.922.

"'19~ 0.257 1n0.688 I• 1.328 1.729 1.850 2.093 2:20$ 2.539 2.861, 3.174 3.579 3.883

20' 0.257 0.887 ~.325 t 1.725 1.844 2.088 I• 2.19] II _2,528 2..845 3.163 3:.552 3.850

~21 0.251 0.888 1,323 I 1,721 1.840 2.DHD 2,189 la 2,618" 2.831 3.135 3.627 3,819

22 0.256;; 0.888

23 . 0.2Ca 0;685

24 0.256 0.685

1.321 1t7t7

·1:3.19 1.7t4

1,3,f8 1.711

1.836

1.832

1.828'

2.074

2.069

2.064

2,183 .2.608 2.819

2.1n

2.600 2.807

2.492 .• 2.797

3.118

3.104

3.091

3.605 3.792

3.-485 I• 3,768

3.~

3.745

25 0.256 0,684 '1.316 1.708 I 1.826 2.060 2.187 2.485" 2.787. . 3.078 3.~

3.72.5

26 0.256 0.684 1.315 1.706 1.822 -~2.068 '2,162 V.79~ 2.779 3.067 3.435' 3.707

27 0.256 0,684 -1.314 . 1~703 .1.819 2J)52 .2..158 2.473 .. 2.Uf 3.057 3.421 - 3.689

28 0256 0.683 1.313 1.701 1~817 2.048 2.1~ 2.487 2.783 3.047 3.408 3.874

o.es, 29 0.2!8 0.683 1.3tt 1.899 1.81" 2.045 . .2.150 2.482 2..766 3.038 3.$96 ,. 3.660

30;; 0.256

1.310 1:697,, 1.812 2.042 2.147 2.467 ,2.760 3.0SO 3.386 '3,84&

SL 0.256. 0.682 .1,309 1.698 1.810 '2.040 2.144 2.463 2,744 3.022 3,376 3.633

32 0.256 .0.682 1,-309 1.894 1.808 · 2,037 2.141 2.449 2.738 3.015 3.366 · 3.622

3S 0.266 0.682 II 1,308 l.892 1.806 1t2,036 2.138 2.445 2.733 3.008 3.356 3.811

34 ·0.266 0.682 111.307 l.691 1.805 2.032 2.136 2.441 2.128 113..002 3.348 3.60·1

36 0.265 0.682 1.306 1.690 ~1.803-,1,2.030 2.133 2.438 2.724 2.996 3.3,CO 3.591

38 0.25$ 0.681' f1,S06 ·1.688; 1.802 .2.028 2.131 2.434 I· 2.719_ 2.990 3,333 ~S.~2

I~37 0.255. 0.-681 " 1~305 .• f.681 1.800 i, 2.028 2.129 2.481 2.716 2.985 3.328 3.57-4

38 0.265 ·0.681 ,. t.304 1.688 1.799 '2.024 2.127 2A29 2.712 2.980 3.319 3.668

39 '" 0.255 0.681 1.304 1.685 1.798 2.023 2.126 2..426" 2.706 2.976 3.313 3.558

-40 0.255 _0.681 1,303 1.684 1,tw 2.021 2.123" 2.423' 2.704 2.971 3,307 3.551

80 .0.2.54 0.878 1,298 .1.67-1 1.781 2.000 2.099 2.390 2.860 2.916 3.232 3.460

80 0.264 0.678 1.292 1.684 1.773 1.990 2.088" 2,374~ 2.639. 2.887 3.195 3.416

100 0.254 0.677 1.290 1.660 11.769 1.984 ,2.081 · 2.-364 2.628 2.871 .3.174 3.390

120 ,;0.254 0.677'' t.289\\ U~58~ 1.766 · 1.980 . 2.076, 2.358. 2.617 2.880, "3.160 3.373

140 ii 0.264 0.676'" 1.288 'f.656 1.l83 1.97l 2.073 2.353. 2.811 2.862 3.148. 3.361

160 .0.254 0.618 1.287 1.654 1.762 1.876 ,2.071 .2.350 2.607 2.847 .,3.142 3.352

180 o.~ 0.676 1.288 1.653 1.761 1.873 2.069 2.347, 2.603 2.842 3.138 3.:345

200 0.254 0.676 1.286 1.853 1.760 1.9n 2.067 2.345 2.601 2.838 ~131 3.340

250 0.264 0.676 1,286 1,651 1,768 1-969 2.065 2.341 2.698 2.832 3.123 3.330

Inf 0.263 0.674 t.282 1,645 111.1.751 1.960 2.054 2.326 2.576 2.807 3.090 3.290

2 Pages 11-20

▲back to top

2.1 Page 11

▲back to top

'F'.',....,,. -.1 • I ..,. I..m l ..,..l • :I ·• J .- .t 491 t .,. 1.ISi I .ms I ·•• I ...,

fi1:',-u1_0IX·•Jofo.osJ>6'ff,...'-.•.,~.fj.uuto""n Jlo~.:•~fI»t..3.1.6.2.9,.I.2,.~....,·1.~I.'6:0,5.11,..1u..3,1_'4.6·.J.j,.1,....J..mfI6.~_~._-1G1~.1,,..

~;_unn:J~•Ju~l5u80 fu1as J~j•~am. J~ -t10ik · "2S11t· 1.u•n .(U4MO

j~ l' f°'20·6.f09.19?{1O·1M-KJluim ftM.l62 Jam16,f,~ f5.31i,m.~ fj.4'1'» I1u•si,{1U16j110usm6

t",iuuu l...;u(,uua .,,..,.,,·1...-·...,.. I~ I••· ju..-IwmoI......,,1,,_.

7 f G.ffl1J ·.:n.mo.~;Ul?J&UlSl& f2.20C111-1~ . uca12 f'1MONI10.~ I I !utlS9 1•.4'931j1u.ne (!uc1sa

~.o{:m~ : 1 fo.tl!P1~K I•• st f11.~I~;,, ·I.~~- '-~• f

! I~ 110t10t'l •~-96l0•1~~' J1!~'•-

• •~• fL~ J11m1. {2.7l2-tf4lAIHt 1~1z {1'2JUS1J.J61US.j107j.)J Il7.'34S3 jllG.M1I2M.USff

t •·734J9!1~'f~ IJ~nI'-•"'f's.aaJu,oo (u.m,s:f1U&U6jru1&nju.ca:mf21.umf2

I~• 11 2.1SSM JU469f7UtMfO4M.5tat'-ffl201,.J3aU4C111126Jas"'111j1uno.j20m11fwonslzs,1

11 imm l1o.sfuuauu t~• (rnm-f,~14 f10.?'_i~.f.1>..,.11i~1f1_P:67Sl4j21.VJ10Sf>

ls.mtt 11

! J.!lOS1 4.ACD~

I~ f1.43142Iu~ J1~·1•~ ftl.o2'07 12l.D'6&')1('21-~2fm

t IS 1""5.(,.U1002 cs.-,s (S.1Jl86 JrMISO J9.l9Sf'l120.»7m15f..m,~rUf ll9J 122.3Q!:Qu3:nS6}021.am j2UIM7

:"'35.~.+.~,_~;,, "~ '4#Hl7 ·l•U600 U287l !6.570j1.611)9Sl f10.1mfu1.nn, IJ1.tl~ j2t.0'414fn•,ilu.um12'.~(1,u2141W

as UJ092 :r;'~sl~1, j1~ Ju.m(11,~ (14.mMJ1~(2l.l01f1uJ,99s1ilzum9J,o.s(m,21.mm

,. ,.,.,.l,s.am1.,- I•~., 1.,..,.I......I,.,.,,.I,w.ioi.f;ru.ilD"I~ 121

. 11f,~ l'-~6 1.56419t~0?•'.·71'1~1,}12.79f11u9,3a1•l~lldi.~ 121.m(1»11,a_o11~ '~·!1147

I lU6'4ao l I I I,,~ IJU:mlf -·II

J ff

t1.01,m Ul07S 9->,1)4(i 1~

i l2U6930-

J3..67SD"17.»'1902l.604ff 2S.9l90

l1.1"'5

17,"foon j1.m73Ja.,90-f6io1:2',i,or 11.GOP1IU42G•O"m6Sfwmt j21.201l1S01.ttm.jnattu,{l&.1tf03t1.S1226

J . M3~M 1..26040 ,.m11 1o~i{llMl6(11s.,4s1191~110ltlf"9i2S.Afn,u•100(34.1•1 J».""3

J~~-lU912! I~ f1'SJm n_

j1~ {11-'?1jl,i,1t1960jt&34431

(2'".61.- lli70S7jJsA1A1 1Jll.m1~4tAll106

fuu,z1,.solAt I I 21

f j j».~ tUm2 JU3IOJ 1u.1e 11.DM2 21.Jno,1 ~m fJ0..11m

i42.-ffl6S

f,.2QI)Q ! f u

f l31.01S63 10.1~n 11~

j 11~1 104796 1L1mo 2U36II %Ulll4 (:n0000 f>S.t72A6

l4t.Qf40 (44.11121

u fuawf10.~(11AOl!lf1J.14l•W~[•u:mslnllm]~risln~~ ~•~soj[~•~:f~~

r--- 2S1Jo.sl96Sf11~11jt1.u1mj HII•~r) 1g.mJ4:124~ f.~~J~~~[9!7..·~- 1~64647j~t[4~lo

• 1,·,:•dak, J™ t ui,u, Jl.843901 U-'1916·' 17.ltlBI (is.»6J43G6A3,4~S•'J11,uasls•••.m•tlUi~l~

I •'%711.amt,1I 2.llfflO11,un3-

_,,._f ,.;fUA<iluu,..,n j,,..,,,.

1164.f-fl•lsl •I•11.1u,.j9m3,ua'J2t..1GI2C~7O,DW}!.1.U._2M,fJ1U,1..,>.fl1•,"1.2.,a.m2m. 1"(o...1.9.'.1,.1,,4:'1.t'.,f2•...MM.f,.fl..,.,.

'"·ll"' -•~J 31 fu.?lffl

If.,~,.~..~..Ih.6...?.,9.,0'!1"?1'7~""''!I2"0.·sm"J"'1wUooA,, IOlt.JUOOJ

1-·1~ 33.7lllli

I~,_ ,~-: S2.:lllQ

34.~ J40.2$60;lf-u.nwi(46.?m4:l~m$J1S171ff