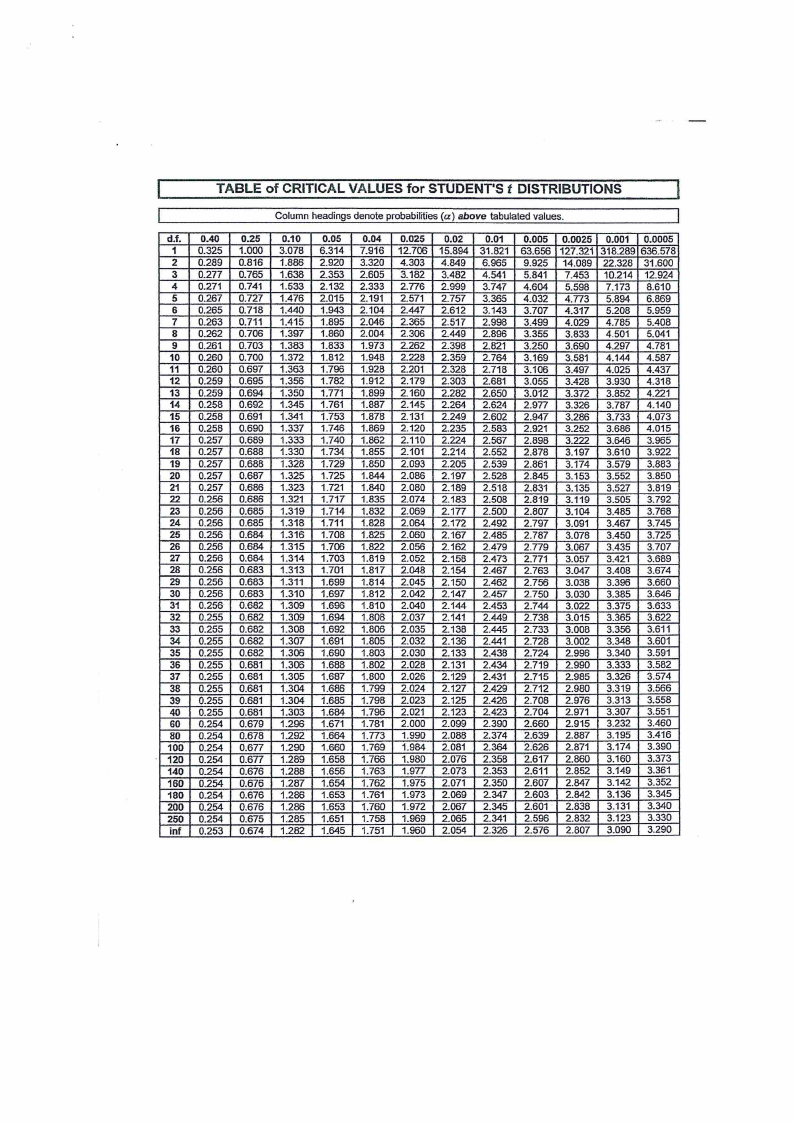
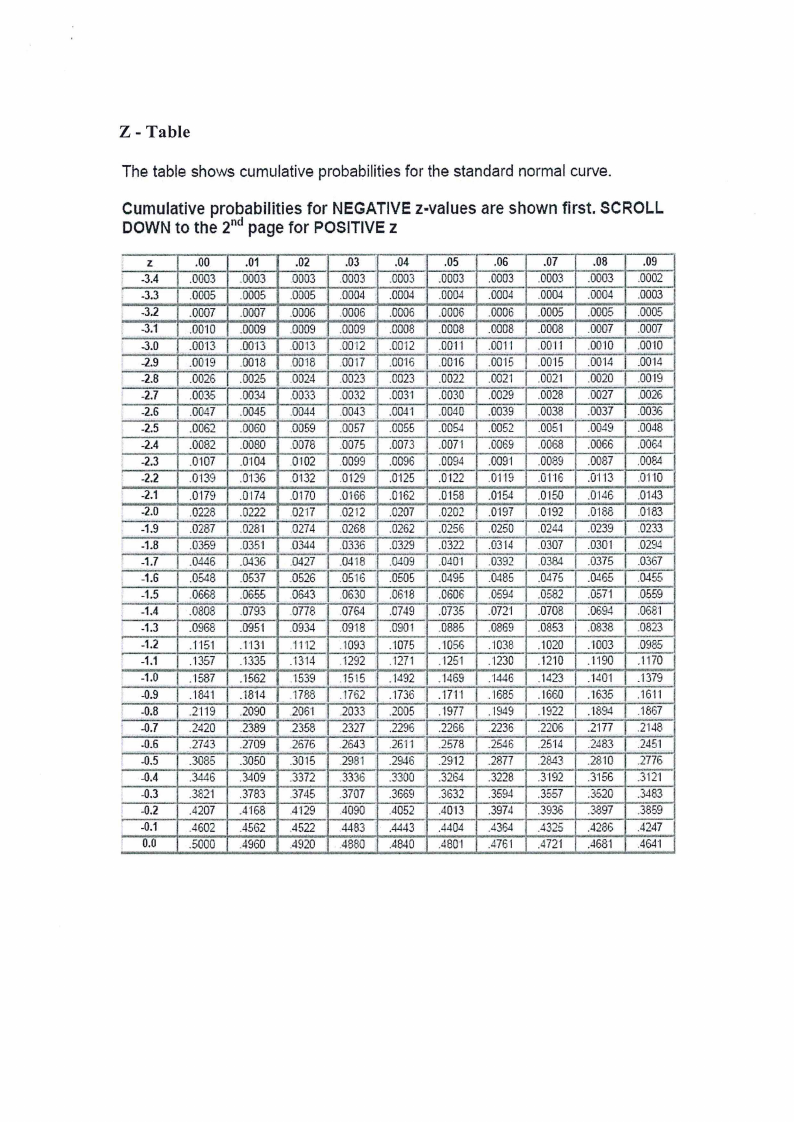
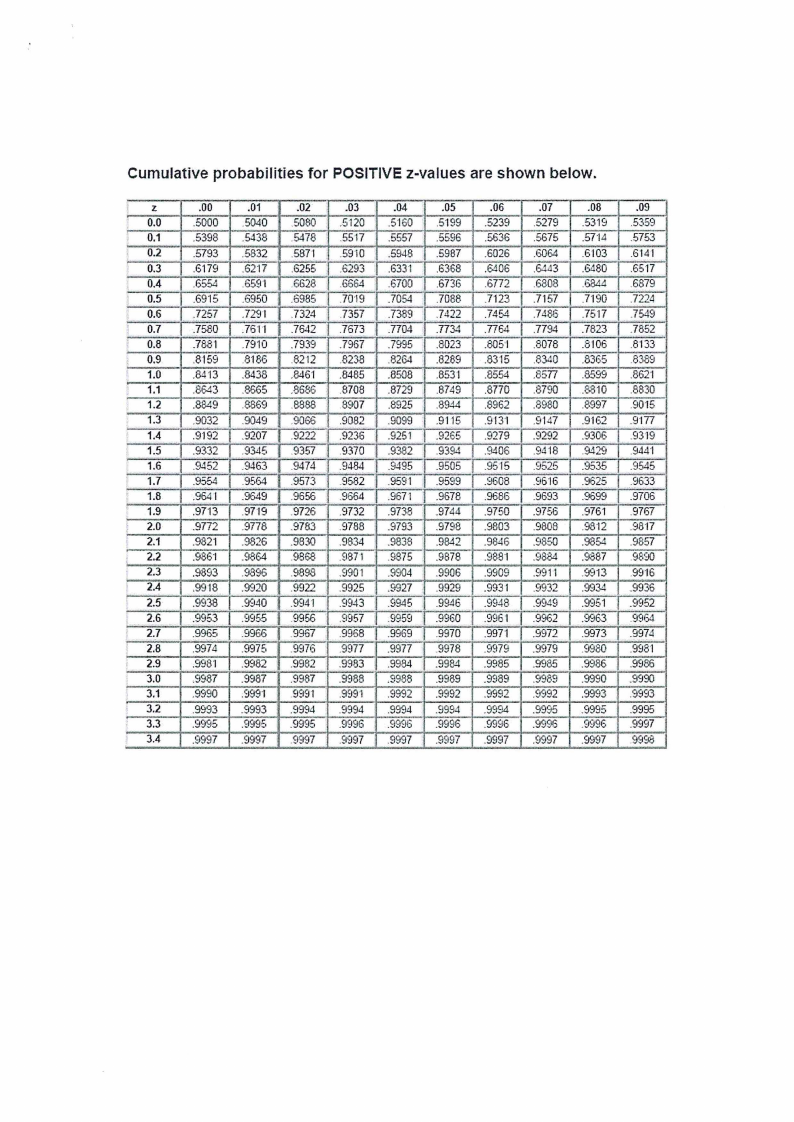
APPENDIX E: The Chi-Square Distribution
~t(,>-'.
1 --r-;;;;-·i-~:-~1~:-soo~: -r:asii-T !-DIO~, idJ\\p-:99s -i---_990
.100
.025
I I ! I i i I I 0.00004 r-;00016 fo.ooo98 0.00393 0.01579 [0.10153 ) 0.45494 j 1.32330 j 2.70554 3.84146 j 5.02389 f 6.63490 7.87944
L .1 I ! ~i 2_ i?·0_1_003 omo10 0.05064 0 !0259
I '! i I ! r 0.57536 1.38629 f2_772;9 4 6051_7}5.99146 ;,37776 9.2!034 . 10 59663
! 3_10.07172 [o.il483 !0.21580_\\Q.35l85 !(l.58437 ~53
j2.36597 _14.l0834 __j6.25139_(781473 j9.34840 _iu.34487f!2_-838161
r r 1 114 , 4 10.20699 0.2971 I 10.48442 10.71072 1.06362 11.92256 3.35669 15.38527 17.77944 i9.48773 1I 1.14329 j 13.27670 .86026 1
f I i ! ! ii S
j 0.41174
,
rl0.55430
I 0.83121
I
~1, .14548
..
1.61031
,
2.67460
I
i!,<i,3j 5146
6.62568
l
1' 9.23636
: I l.07050
•
!I 12.83250
j
!
15.08627
16.74960
1
----·-----r---------------I---------·---c---·-·-i··----·--------i!-------!·-·-·--!--·--------.----·· jr!·-67---·j!'D0·..-69-78·5-97•2-36···j!r··01--.·.8·2-.·73.-2-9·00-94····Jj1-l·1-.-6.·2.8-.3_-9.7.8_.37_4__,!_-21-.·.16-6-3·75-3-35·8-·--\\!,22-,.82·30-3·4·11·13·--\\j-34r.,-42-55-4--16r80·5---j6-5,..334•1588112
i 7.84080 10.644M 12.59159 14.44938
-·--··•·--.---·----·-1-·-·--.-..--.-. --·-··---------
!9.03715 12.01704, 14.06714116.01276
j 16.81189
I··1-8-.-4·7-5-31
!r1-~-:-5-4758
jl0.2777_4
1
i ! f i ! s 1.34441 f 1.64650 2.17973 12.13264 J3.48954 f5.01064 [7.34412 flo.21ss5 13.36l51 1s.so131!11.53455120.09024 !21.95495
! ! i4J6816i i 9 Jl.TI49312.08790 {?0039 !3.32511
5.89883 18,34283 JI l.38875 14.68366: 16.91898 jl9.02277 ~9123.58935
! ! ·10-·12.15586 12.55821 ·13.24697-i3,94030 .. 'l4.86518 ..T6.73720--·19.34182 !12.54886-!15.98718 18.30704•120.48318 l23.20925 j25.18818.
~!2.60322
13.05348 13.81575 j4.57481 is.57778 i1.s8414 ~34100 j13-10069 f!7.21so1 i19.67514 i21.92005 j24.7249; fu.156s5:
ii. ! JJ:oi1sr2is··10:s1::;:;4·1Jj:,9-r;;:22w:i
r! ,4I ! 13 3.56503 14.10692 5.00875 Js.89186
14.07467 -14,66043 !s.62873 )6.57063
! i i6~jo:is·;;·-18.43842 11.34032 14 s4s40J1&.S:i;jjs"Fi~o216U:02116·?1r;j:23s~~2939532··1666-
! i i ! 17 f .04150 9_29901 112.33976 is.98391 19.81193 22.36203 24.73560 ~129.81941;i
17.78953 [J0.16531 [13.J3927i11.11693i21.06414 l23.68479l26.11895j29.14124'31.31935·
! ! \\ rs 14.60092 _fs:22935 j 6.26214 j 7.26094 js.54676 11.03654·114.33886 18.24509 jn.30113 ~9
I j21.48&39 130.51791 32.80132
!16!5.14221 15.81221 JG.90766 j7.96165 j9.31224 111.91222 ~s5oj19.368&6!23.S4:83
\\26.29623 [2s.84535-i31:99993r"-26119
i i :
'
11
'15.69722
.------16.4077.656419
.
•
_18.67176
'
!1 10.08519
1112.19193
'
16.33818 i 20.48868
!.
'I24.76904
·121.ss111
!. .10.19101
fo.40866
I
'35.71847
,.,;.:,,
l
! i ! J i ! ~! I 18 6.26480 11.01491 s.23075 9.39046 _!10.86494 fo.61529 fl7.3379o 21.60489 !25.98942 28.86930
34.80531 37. 15645
! I I ! ! i i p [_19 6.84397 )7.63273 8.90652 ['io.11101 t.65091 14.56200 tS.33765 j 22.111s1 21.20351 30.14353 32_s5233 j 36.19os1 j3&.58226.
L ! i I I i i ! .i 20 r-43384 f'ii604o i9.59078 10.s5081 12.44261 15.45111 19.33743 23.82769 28.41198 : 31.41043 34.16961 37.56623 9.99685
I I i i I l 13 I 21 j8.03365 fs.891_20 10.28290
! if3.23960 1.r6.H138 20 33723 24.93478 29.61s09. n.67057'! 35.47888 38.93217 J41.40106
I f l I I .I : 22 fs.54212 9.54249 1ni.98232 12.33801 114.04149 11.23962 2u3104 : 26.03921 : 30.81328
40.28936 42.79565
/nf 1 11 i 9.26042- l0.19572 ~
3~09051 114.84796 Gs.J;;;;;;-~sf2ii.i134
13 132.00690 s.17246 i38 o7563 i4t.63840144.18128
i ~ r 19.88623 110:85636 : 12.40115 113.84843 115.65868 19.03725r23.33673 128.24115 133.19624 : 36.41503 13936408 [42.97982 145.55851
l ! r ! i 25 flo.s1965 jll-52398 : 13.11972 !14.6114I 116.47341 19.93934 24.33659 i29.33885 -!~4.:3_8_15193?.65248_ ~0.64647 i44~314J(Jr46.92789
i26l 1 r r 13 .r I 1.16024 rru9815_ 13.84390; 15_.37916117.29188 20.84343 125.33646130.43457 35.56317 s.88514 41.9231: f45.~1_68 ,48.28988
1
! ! i ! i i f21-fli.so159,/ 12.87850 14.57338 16.15140 18.11390 j21.14940 26.336J4 j 3t.52841 36.74122 40.11n1 j43.19451 ~!49-64492-.
fl8TJ'2A6134rl3:56471
I ! 15.30786 16.92788i18.93924122.65716 j27.33623 !32.62049 ["n9isi?:fii·:;;:;1·,i·r«~46079~!48.27s24
iso.99338
! i 1 ! i29i13.121 )5 114.25645 16.04101 111.10837 19.76774 23.56659 28.336!3 [33.11091 139.08747
I J45.12229 49.58788 rs2.33562
! 30 j 13.78672·114.9534611~.79077 Jl8.49266 fio.m23 J24.4776I /29.33603 j34.79974 !40.25602 !43.77297 \\46.97924 l.50.892l8 f.sJ.67196
Page7 ol7