 |
TSA701S - TIME SERIES ANALYSIS - 2ND OPP - JULY 2022 |
 |
1 Page 1 |
▲back to top |
NAMIBIA UNIVERSITY
OF SCIENCE AND TECHNOLOGY
FACULTY OF HEALTH AND APPLIED SCIENCES
DEPARTMENT OF MATHEMATICS
| QUALIFICATION:
QUALIFICATION
CODE:
COURSE CODE:
| SESSION:
| DURATION:
BACHELOR OF SCIENCE IN APPLIED MATHEMATICS AND
STATISTICS
07BAMS
LEVEL: 7
TSA701S
JULY 2022
3 HOURS
COURSE
NAME:
| PAPER:
| MARKS _
TIME SERIES
THEORY
100
ANALYSIS
| SUPPLEMENTARY/ 2ND OPPORTUNITY EXAMINATION QUESTION PAPER
| EXAMINER
| Dr. Jacob Ong’ala
| MODERATOR
| Prof. Lilian Pazvakawambwa
|
|
INSTRUCTION
1. Answer all the questions
2. Show clearly all the steps in the calculations
3. All written work must be done in blue and black ink
THIS
QUESTION
PERMISSIBLE MATERIALS
Non-programmable calculator without cover
PAPER CONSISTS OF 3 PAGERS (including the front page)
 |
2 Page 2 |
▲back to top |
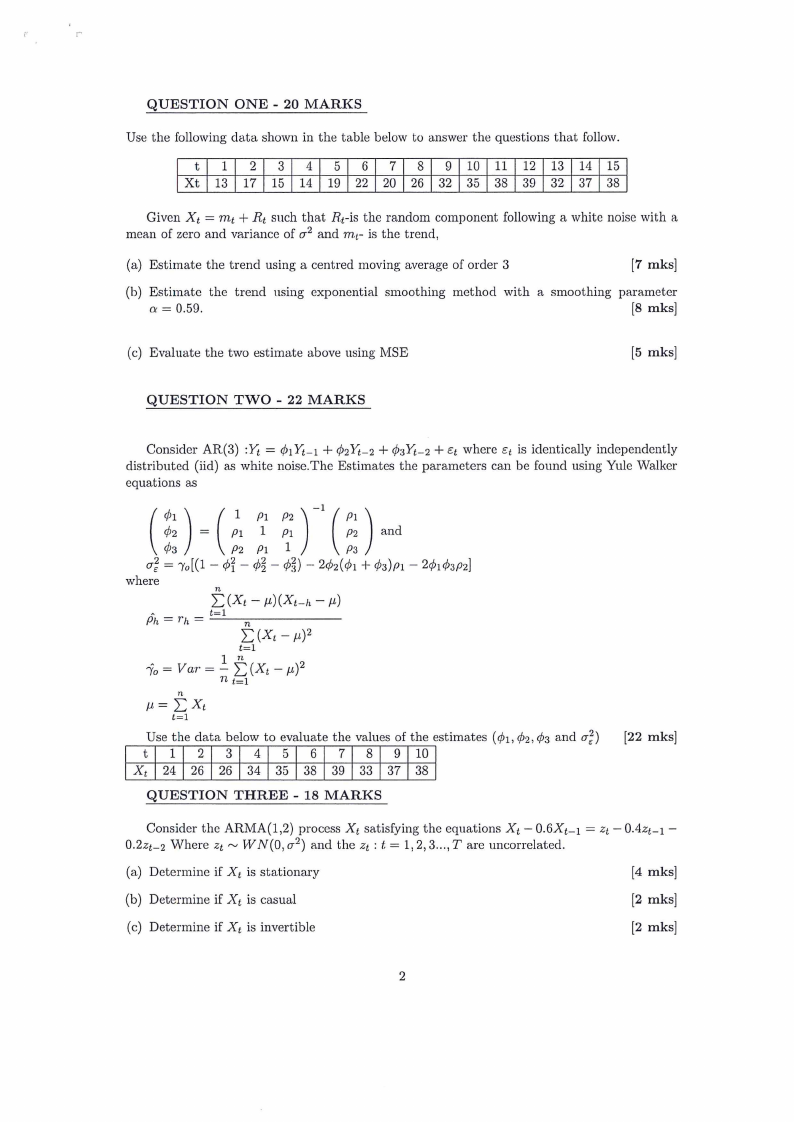
QUESTION ONE - 20 MARKS
Use the following data shown in the table below to answer the questions that follow.
t} 1] 2} 3] 4] 5] 6] 7] 8] 9} 10} 11} 12] 13] 14] 15
Xt | 13 | 17 | 15 | 14] 19 | 22 | 20 | 26 | 32 | 35 | 38 | 39 | 32 | 37 | 38
Given X; = m+ R; such that R;,-is the random component following a white noise with a
mean of zero and variance of o? and m- is the trend,
(a) Estimate the trend using a centred moving average of order 3
[7 mks]
(b) Estimate the trend using exponential smoothing method with a smoothing parameter
a = 0.59.
[8 mks]
(c) Evaluate the two estimate above using MSE
[5 mks]
QUESTION TWO - 22 MARKS
Consider AR(3) :¥; = $1¥:-1 + $2Yi-2 + 3Y:-2 + €: where e; is identically independently
distributed (iid) as white noise.The Estimates the parameters can be found using Yule Walker
equations as
1
lan p\\
(nn
g2 =} a 1 pr
p2 } and
$3
p2 pr i
p3
a2 = yo[(1 — ¢? — $3 — $2) — 2b2(¢1 + 63)p1 — 2614372]
where
n
2 (Xt — p)(Xt-n — pb)
br =) = — = _
foa =
Var
=
=L
9&t°=1((XX—i
— yu)?
a)?
nN t=1
w= tD=1X
Use the data below to evaluate the values of the estimates (¢1,¢2,¢3 and a?)
t/ 1} 2] 3] 4] 5] 6] 7} 8] 9} 10
X;, | 24 | 26 | 26 | 34 | 35 | 38 | 39 | 33 | 37 | 38
QUESTION THREE - 18 MARKS
[22 mks]
Consider the ARMA(1,2) process X; satisfying the equations X; — 0.6X;_-1 = 2 —0.4z%-1 -
0.2z4-2 Where x ~ WN(0,o?) and the x : t = 1,2,3...,T are uncorrelated.
(a) Determine if X; is stationary
[4 mks]
(b) Determine if X; is casual
[2 mks]
(c) Determine if X; is invertible
[2 mks]
 |
3 Page 3 |
▲back to top |

(d) Write the coefficients VY; of the MA(oo) representation of X;
QUESTION FOUR - 20 MARKS
[10 mks]
(a) State the order of the following ARIMA(p,d,q) processes
[12 mks]
(i) Y; = 0.8Yi-1 + e¢ + 0.7e:_-1 + 0.6e:-2
(ii) Y; = Yi + e¢ — Oez-1
(ili) Y, = (1+ ¢)Yin — 6Ni-2 + e&
) (iv Yi =5+ et — $et-1 = $et-2
(b) Verify that (max p; = 0.5 nd min p; = 0.5 for —oo < @ < oo) for an MA(1) process:
X;, = €; — Ge4_1 such that €; are independent noise processes.
[8 mks]
QUESTION FIVE - 20 MARKS
A first order moving average MA(2) is defined by Xz; = 2 + 0124-1 + 0224-2 Where z ~
WN(0,o7) and the y% : ¢ = 1,2,3...,T are uncorrelated.
(a) Find
(i) Mean of the MA(2)
(ii) Variance of the MM A(2)
(iii) Autocovariance of the M A(2)
(iv) Autocorrelation of the MA(2)
[2 mks]
[6 mks]
[8 mks]
[2 mks]
(b) is the MA(2) stationary? Explain your answer
[2 mks]





