 |
MTA611S - MATHEMATICS FOR AGRIBUSINESS - 2ND Opp - JULY 2022 |
 |
1 Page 1 |
▲back to top |

nAmlBIA unlVERSITY
OF SCIEnCE Ano TECHnOLOGY
FACULTYOF HEALTHAND APPLIEDSCIENCESAND NATURAL RESOURCES
DEPARTMENT OF AGRICULTURE & NATURAL RESOURCESSCIENCES
QUALIFICATION: BACHELOR OF SCIENCEIN AGRICULTURE
QUALIFICATION CODE: 07BASA
LEVEL: 6
COURSE CODE: MTA611S
COURSE NAME: Mathematics for Agribusiness
DATE: July 2022
PAPER: Theory
DURATION: 3 Hours
MARKS: 100
SECOND OPPORTUNITY EXAMINATION QUESTION PAPER
EXAMINER(S)
MODERATOR:
Mr. Mwala Lubinda
Mr. Teofilus Shiimi
INSTRUCTIONS
1. Attempt ALL the questions.
2. Write clearly and neatly.
3. Number the answers clearly and correctly.
PERMISSIBLEMATERIALS
1. All written work MUST be done in blue or black ink
2. Calculators allowed
3. No books, notes and other additional aids are allowed
THIS QUESTION PAPER CONSISTS OF 6 PAGES (including this front page).
 |
2 Page 2 |
▲back to top |

QUESTION ONE
a. Define the limit of a function at a point a. Explain how you would use the limit
concept to determine whether a function is continuous at a point a.
= b. Suppose the profit from selling n items is known to be P(n)
+ - 3.
2
Find P(7) and P(28).
c. Use interval notation to express the domain and range of the following function:
P(n) = n-2 + - 3
[MARKS]
(4)
(4)
(4)
d. Suppose the production function for a food processor is represented by a
quadratic function with maxima and roots at -10 and 5. Based on this
information, answer the questions below
i. Derive the mathematical equation of the production function.
(3)
ii. Find the critical point of the production function you have derived in d(i).
(5)
iii. Draw and label a graph that illustrates the production function. The graph
must clearly show the roots, maxima, and y-intercept points of the
(5)
production function.
TOTAL MARKS
[25]
a. Given a function:
QUESTION TWO
[MARKS]
(6)
Find Zxx, Zyy and Zxy·
= b. Let p 100 - q2 be the demand function for an Agribusiness's product. Find
the rate of change of price, p, per unit with respect to quantity, q. How fast is the
= price changing with respect to q when q 5? Assume that pis in dollars. (Hint:
(4)
the rate of change implies the derivative).
c. Find:
i.
Iim _(x_-_h_)-__zx_z
h->O
h
(3)
ii. I1. m-3-t-12
t->4 t - 4
(3)
Find an equation of the tangent line to the curve
(9)
 |
3 Page 3 |
▲back to top |
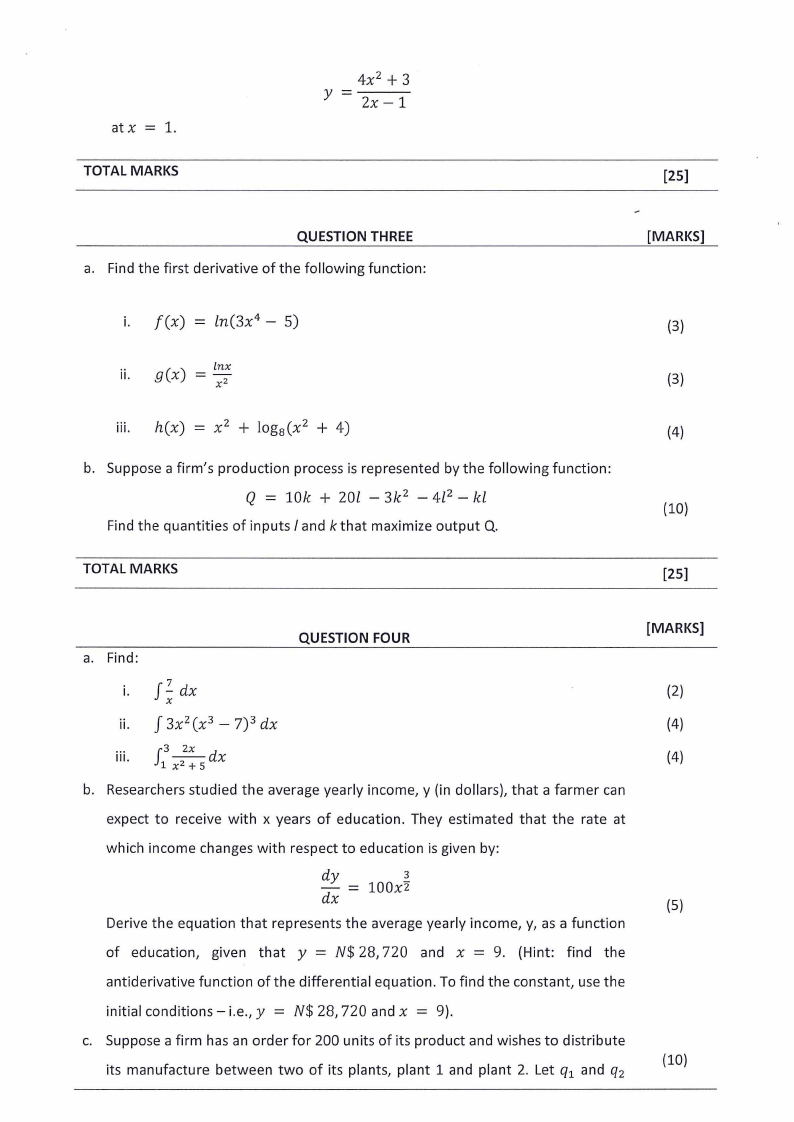
= at X 1.
4x 2 + 3
y
2x-1
TOTAL MARKS
[25]
QUESTION THREE
a. Find the first derivative of the following function:
i. f(x)
ln(3x 4 - 5)
ii. g(x)
lnx
xz
= iii. h(x) x 2 + log8 (x 2 + 4)
b. Suppose a firm's production process is represented by the following function:
= Q 10k + 20l - 3k 2 - 4l 2 - kl
Find the quantities of inputs/ and k that maximize output Q.
TOTAL MARKS
[MARKS]
(3)
(3)
(4)
(10)
[25]
a. Find:
QUESTION FOUR
i. J?.dx
X
J ii.
3x 2 (x 3 - 7) 3 dx
f iii.
3 ~dx
1 x2 + 5
b. Researchers studied the average yearly income, y (in dollars), that a farmer can
expect to receive with x years of education. They estimated that the rate at
which income changes with respect to education is given by:
-dy
=
3
lOOxz
dx
Derive the equation that represents the average yearly income, y, as a function
of education, given that y = N$ 28, 720 and x = 9. (Hint: find the
antiderivative function of the differential equation. To find the constant, use the
initial conditions - i.e., y = N$ 28, 720 and x = 9).
c. Suppose a firm has an order for 200 units of its product and wishes to distribute
its manufacture between two of its plants, plant 1 and plant 2. Let q1 and q2
[MARKS]
(2)
(4)
(4)
(5)
(10)
 |
4 Page 4 |
▲back to top |

denote the outputs of plants 1 and 2, respectively, and suppose the total-cost
function:
C = /(qi, qz) = 2qf + q1q2 + q~ + 200
How should the output be distributed to minimize costs? {Hint: the constraint
faced by the firm is: q1 + q2 = 200).
TOTAL MARKS
[25]
THE END
 |
5 Page 5 |
▲back to top |

FORMULA
Basic Derivative Rules
Con~1:u11Rule. ~(c)- 0
ax
Conmm
~!ultiplc Rule
~[c/(x)I-
de
cf"(x)
Sum Ruic- ~li(x)-g(x)I
ax
- /Xx)- g·(x)
P,orln,, Rnlr ~[f(r)g(r)J
ax
/(x)g'(x)-g(.,Jj'(r)
.!;- Ch.,in Ruic' ax /(g(x)) - f Xe(x))eXx)
Derivative Rules for Exponential Functions
!!_(e') = e'
dx
~(a')= a' In a
dx
~(e'<'>)
dx
= ·en'>g '(x)
~(a' 1n) = ln(a) a ,r,> g '( x)
dx
Derivative Rules for Logarithmic Functions
x) -,x -(dln
=I > 0
dx
x
-ldn(g(x))
dx
= -0" '( x)
-·-
g(x)
-dd(xlog.
x)= --,xI
x ln a
>0
- d ( lo o g ( X ) ) -_ ---"-g---'--'('--x--)
d x "• ·
g ( X) ln a
Basic Integration Rules
I. fa,fr=llx+C
2. fx"dx=;:·11:;,>e-l c.
f 3. ~cfr=lnJxl+C
f 4. e·' (b: = e' + C
5.
6.
a',fr=-+Cll'
f Ina
f lnxdx = xlnx-x+
C
Integration by Substitution
The following are the 5 steps for using the
integration by substitution metthod:
• Step 1: Choose a new variable u
• Step 2: Determine the value dx
• Step 3: Make the substitution
• Step 4: Integrate resulting integral
• Step 5: Return to the initial variable x
Integration by Parts
The formula for the method of integration by
parts is:
_/udv = ·u•v- ;·vd1t
There are three steps how to use this formula:
• Step 1: identify u and dv
• Step 2: compute u and du
• Step 3: Use the integration by parts
formula
Unconstrained
optimization:
Univariate
functions
The following are the steps for finding a solution
to an unconstrained optimization problem:
• Step 1: Find the critical value(s}, such
that:
f '(a) = 0
• Step 2: Evaluate for relative maxima or
minima
o If f "(a) > 0 minima
o If f "(a) > 0 maxima
Unconstrained optimization: Multivariate
functions
The following are the steps for finding ·a
solution to an unconstrained optimization
problem:
Constrained Optimization
The following are the steps for finding a solution
to a constrained optimization problem using the
Langrage technique:
• Step 1: Set up the Langrage equation
Re/alive 11wxi111111n Re/arive 111i11i11111111
I. f,.f,. =0
I. f,.f,. = 0
2. fu, f,.,<. ()
2. f,.,,f,.,>. 0
3. f,, ·/:...,>(f,,.}2 3. L -j,.,.>U,.Y
• Step 2: Derive the First Order Equations
• Step 3: Solve the First Order Equations
• Step 4: Estimate the Langrage Multiplier
 |
6 Page 6 |
▲back to top |

Additionally:
•
If fxx
· [yy
<
2
Ctxy)
, when
fxx
and [yy
have the same signs, the function is at
an inflection point; whenfxx and [yy
have different signs, the function is at
a saddle point.
•
If
fxx
· [yy
=
2
Ctxy)
,
the
test
is
inconclusive.





