 |
PBT602S - PROBABILITY THEORY 2 - 1ST OPP - JUNE 2023 |
 |
1 Page 1 |
▲back to top |

<
nAmlBIA UnlVERSITY
OF SCIEnCE Ano TECHnOLOGY
FACULTYOF HEALTH, NATURAL RESOURCESAND APPLIEDSCIENCES
SCHOOLOF NATURALAND APPLIEDSCIENCES
DEPARTMENTOF MATHEMATICS, STATISTICSAND ACTUARIALSCIENCE
QUALIFICATION: Bachelor of Science in Applied Mathematics and Statistics
QUALIFICATION CODE: 07BSAM
LEVEL: 6
COURSE CODE: PBT602S
COURSE NAME: Probability Theory 2
SESSION: JUNE 2023
DURATION: 3 HOURS
PAPER: THEORY
MARKS: 100
EXAMINER
FIRST OPPORTUNITY EXAMINATION QUESTION PAPER
Dr D. B. GEMECHU
MODERATOR:
Prof R. KUMAR
INSTRUCTIONS
1. There are 5 questions, answer ALL the questions by showing all the necessary steps.
2. Write clearly and neatly.
3. Number the answers clearly.
4. Round your answers to at least four decimal places, if applicable.
PERMISSIBLE MATERIALS
1. Nonprogrammable scientific calculators with no cover.
THIS QUESTION PAPER CONSISTS OF 3 PAGES (Including this front page)
 |
2 Page 2 |
▲back to top |
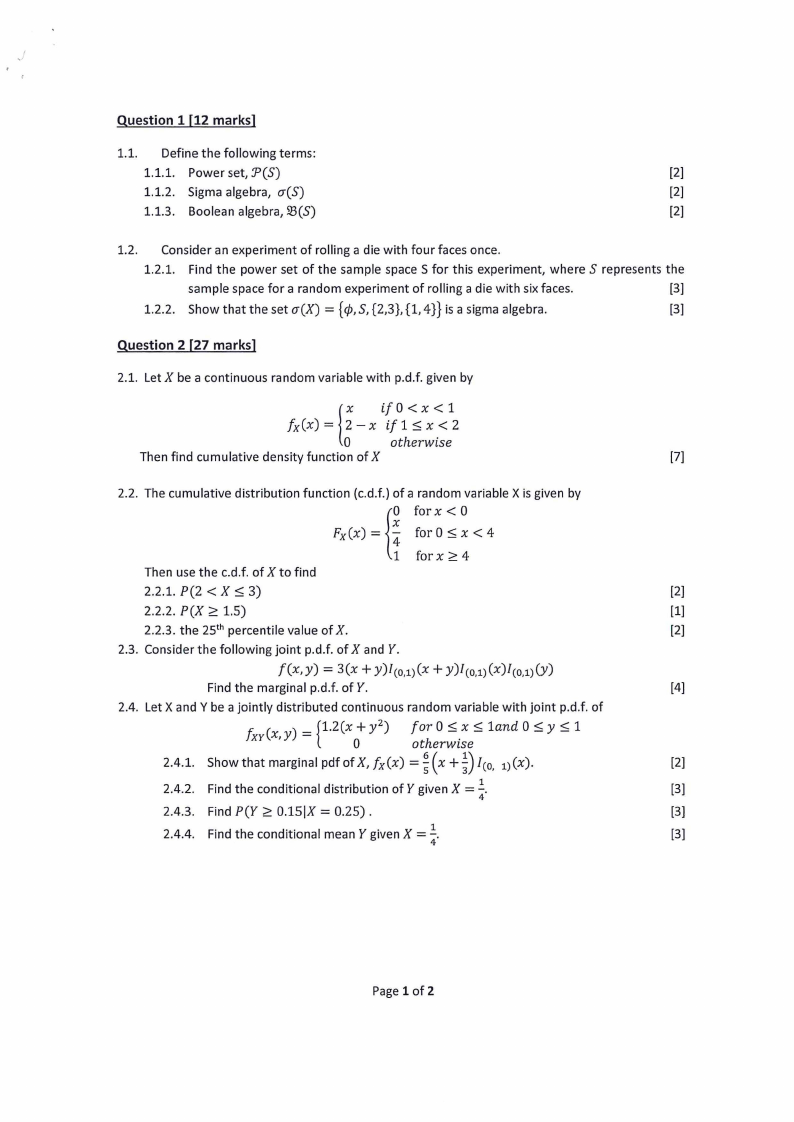
Question 1 [12 marks]
1.1. Define the following terms:
1.1.1. Power set, P(S)
[2]
1.1.2. Sigma algebra, cr(S)
[2]
1.1.3. Boolean algebra, 5B(S)
[2]
1.2. Consider an experiment of rolling a die with four faces once.
1.2.1. Find the power set of the sample space S for this experiment, where S represents the
sample space for a random experiment of rolling a die with six faces.
[3]
= { 1.2.2. Show that the set cr(X) ¢, S,{2,3}, {1, 4}} is a sigma algebra.
[3]
Question 2 [27 marks]
2.1. Let X be a continuous random variable with p.d.f. given by
!X if O < X < 1
fx (x) = 2 - x if 1 :s;x < 2
0
otherwise
Then find cumulative density function of X
[7]
2.2. The cumulative distribution function (c.d.f.) of a random variable Xis given by
~ for X < 0
Fx(x) = {-4 for O :s;x < 4
1 for X 2::4
Then use the c.d.f. of X to find
2.2.1. P(2 < X :s;3)
[2]
2.2.2. P(X 2::1.5)
[1]
2.2.3. the 25th percentile value of X.
[2]
2.3. Consider the following joint p.d.f. of X and Y.
f(x,y) = 3(x + y)Ico.i)(x + y)Ico.i)(x)Ico.1)CY)
Find the marginal p.d.f. of Y.
[4]
2.4. Let X and Y be a jointly distributed continuous random variable with joint p.d.f. of
[;XY (X,y
)
_
-
{1.2(x + y 2)
0
for O :s;x :s;land O:s;y :s;1
otherwis. e
2.4.1. Show that marginal pdf of XJx(x) = %(x +½)I co. 1)(x).
[2]
2.4.2. Find the conditional distribution of Y given X = ¾-
[3]
= 2.4.3. Find P(Y 2::0.15IX 0.25).
[3]
2.4.4. Find the conditional mean Y given X = .!..
[3]
4
Page 1 of 2
 |
3 Page 3 |
▲back to top |

Question 3 [24 marks]
3.1. Let X and Y be two random variables and let a, b, c and k be any constant numbers. Then
= Cov(aX + c, bY + k) abCov(X, Y).
[SJ
3.2.
Let Y1 , Y2 ,
and Y3
be three
random
variables with
E(Y1 )
= 5, E(Y2 )
= 12, E(Y3 )
=
4,
CJf=
1
2,
CJf=
2
3,
CJf=
3
1,
CJ1yy2
= -0.6,
CJ1yy3 =
0.3, and CJ2yy3 =
2. If R
=
2Y1 -
3Y2 + Y3, then find
3.2.1. the expected value of R.
[2J
3.2.2. the correlation coefficient between Y1 and Y3 and comment on your result.
[3J
3.2.3. the variance of R.
[SJ
3.3. The joint probability density function of the random variables X, Y, and Z is
[ixyz f(x,y,z) =
2
,
0 < x < l; 0 < y < 1; 0 < z < 3,
0, elsewhere.
Find the joint marginal density function of Y and Z. Hint: find fyz(Y, z).
[4J
3.4. If X1 , X2 , and X3 are DISCRETE random variables with joint p.m.f. f (x1 , x2 , x 3 ), then for any
constants c1, c2 and c3, show that E(If=iciXi) = Lf=iciE(Xi)-
[SJ
QUESTION 4 [17 marks]
4.1. Suppose that Xis a random variable having a binomial distribution with the parameters n and p
(i.e., X ~Bin(n, p)).
4.1.1.
4.1.2.
= ( f. Show that the moment generating function of X is given by Mx(t) l - p(l - et)
Hint: (a+ b)n = rr=oG)akbn-k_
[4J
Find the cumulant generating function of X and hence find the first cumulant.
[SJ
= 4.2. Let the random variables Xk~Poisson(l.k) for k l, ..., n be independent Poisson random
variables. If we define another random variable Y = X1 + X2 + ···+ Xn, then find the
characteristics function of Y, cpy(t). Comment on the distribution of Y based on your result. [Hint
c/Jxk(t) = eJ.k(eit_1)].
[8J
QUESTION 5 [20 marks]
5.1. Suppose that X and Y are independent, continuous random variables with densities fx(x) and
= fy(y). If Z X + Y, then show that the density function of Z is
fz(z) = J~ fx(z - y)fy(y)dy.
[SJ
00
5.2. Let X and Y be independent Poisson random variables with parameters il 1 and il 2 . Use the
convolution formula to show that X + Y is a Poisson random variable with parameter il 1 + il 2 .
[7J
= = 5.3. Let X1 and X2 have joint p.d.f. f(x 1, x2 ) ze-Cxi+xz) for 0 < x1 < x2 < l. Let Y1 X1 and
= Y2 X1 + X2 • Find the joint p.d.f. of Y1 and Y2 , g(y 11 y2 ).
[8J
===END OF PAPER===
TOTAL MARKS: 100
Page 2 of 2





