 |
MMP701S - MATHEMATICAL METHODS IN PHYSICS - 1ST OPP - JUNE 2023 |
 |
1 Page 1 |
▲back to top |

nAmlBIA UnlVERSITY
OF SCIEnCE Ano TECHn
FACULTYOF HEALTH,NATURALRESOURCESAND APPLIEDSCIENCES
SCHOOLOF NATURALAND APPLIEDSCIENCES
DEPARTMENT OF BIOLOGY,CHEMISTRYAND PHYSICS
QUALIFICATION: BACHELOR OF SCIENCE
QUALIFICATION CODE: 07BOSC
COURSECODE: MMP701S
SESSION:JUNE 2023
DURATION: 3 HOURS
LEVEL: 7
COURSENAME: MATHEMATICAL METHODS
IN PHYSICS
PAPER:THEORY
MARKS: 100
EXAMINER(S)
MODERATOR:
FIRSTOPPORTUNITY EXAMINATION QUESTION PAPER
Prof Dipti Ranjan Sahu
Prof S. C. Ray
INSTRUCTIONS
1. Answer ALL the questions.
2. Write clearly and neatly.
3. Number the answers clearly.
PERMISSIBLE MATERIALS
Non-programmable Calculators
THIS QUESTION PAPER CONSISTS OF 3 PAGES (Including this front page)
 |
2 Page 2 |
▲back to top |
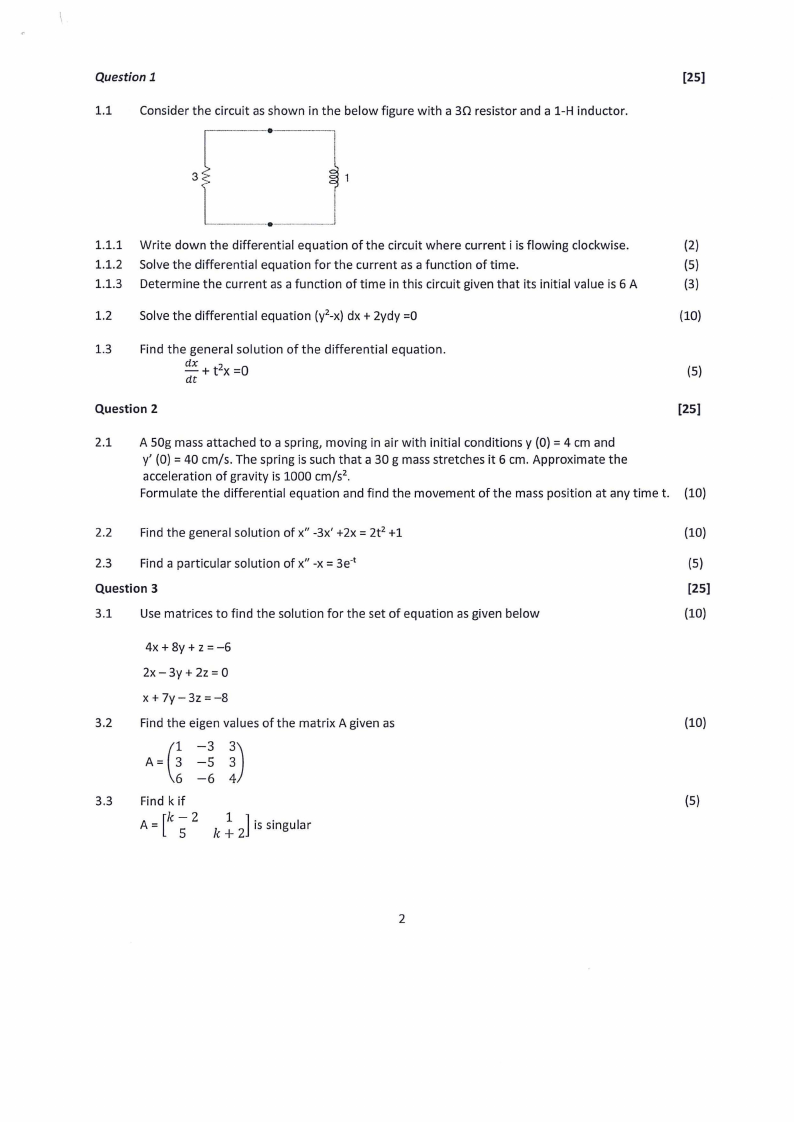
Question 1
[25]
1.1 Consider the circuit as shown in the below figure with a 30 resistor and a 1-H inductor.
1.1.1 Write down the differential equation of the circuit where current i is flowing clockwise.
(2)
1.1.2 Solve the differential equation for the current as a function of time.
(5)
1.1.3 Determine the current as a function of time in this circuit given that its initial value is 6 A
(3)
1.2 Solve the differential equation (y2-x} dx + 2ydy =O
(10}
1.3 Find the general solution of the differential equation.
-d+x t2x =0
(5}
dt
Question 2
[25]
2.1 A SOgmass attached to a spring, moving in air with initial conditions y (O}= 4 cm and
y' (O)= 40 cm/s. The spring is such that a 30 g mass stretches it 6 cm. Approximate the
acceleration of gravity is 1000 cm/s 2•
Formulate the differential equation and find the movement of the mass position at any time t. (10)
2.2 Find the general solution of x" -3x' +2x = 2t2 +1
2.3 Find a particular solution of x" -x = 3e-t
Question 3
3.1 Use matrices to find the solution for the set of equation as given below
(10}
(5)
[25]
(10}
4x + 8y + z =-6
2x-3y + 2z = 0
X + 7y-3z =-8
3.2 Find the eigen values of the matrix A given as
(10}
=~ ) A=(~
6 -6 4
3.3 Find kif
(5)
[k; A=
2
k
+1
]
2
.1ssm. guIar
2
 |
3 Page 3 |
▲back to top |

Question 4
[25]
4.1 Show that for inner product space C [-rr, rr], the functions Sint and Cost are orthogonal.
(5)
4.2. Obtain an orthogonal basis for the subspace of R4spanned by x1=(1, 0, 1, 0), x2=(1, 1, 1, 1),
X3 = (-1, 2, 0, 1) using Gram-Schmidt process.
(10)
4.3 Using the Laplace transform find the solution for the following equation
(5)
a~~t) - 5 y(t) = e (st) with initial conditions y(0) = 0 Dy(0) = b
4.4 Obtain the value of P3 (x) using Rodrigues' formula
(S)
P (x) = - -I-- -d-"---(x- 2 -1) ,,
II
(2 11)11!d'("
..................................................................... END...................................................................................................
3





