 |
MMP701S - MATHEMATICAL METHODS IN PHYSICS - 2ND OPP - JULY 2023 |
 |
1 Page 1 |
▲back to top |

nAmI BI AunIVE RSITV
OF SCIEnCE Ano TECHno LOGY
FACULTYOF HEALTH,NATURAL RESOURCESAND APPLIEDSCIENCES
SCHOOLOF NATURALAND APPLIEDSCIENCES
DEPARTMENT OF BIOLOGY,CHEMISTRYAND PHYSICS
QUALIFICATION : BACHELOROF SCIENCE
QUALIFICATION CODE: 07BOSC
COURSECODE: MMP701S
SESSION:JULY 2023
DURATION: 3 HOURS
LEVEL: 7
COURSENAME: MATHEMATICAL METHODS
IN PHYSICS
PAPER:THEORY
MARKS: 100
SUPPLEMENTARY/SECONDOPPORTUNITY EXAMINATION QUESTION PAPER
EXAMINER{S) Prof Dipti RanjanSahu
MODERATOR: Prof. S. C. Ray
INSTRUCTIONS
1. Answer ALL the questions.
2. Write clearly and neatly.
3. Number the answers clearly.
PERMISSIBLE MATERIALS
Non-programmable Calculators
THIS QUESTION PAPER CONSISTS OF 3 PAGES (Including this front page)
 |
2 Page 2 |
▲back to top |

Question 1
[25]
1.1 The law of decay states that the rate of decay for a radioactive material is proportional to the
number of atoms present.
1.1.1 Formulate the differential equation and determine the amount of radioactive material left
at any time, t by solving the differential equation.
(5)
1.1.2 Determine the half-life of a radioactive material using solution of differential equation. (5)
1.1.3 In two years, 3 g of a radioisotope decay to 0.9 g. Determine both the half-life T and the
decay rate k.
(5)
1.2 Solve the equation,
= -ddx+t t 2x Cost
(5)
1.3 Solve the differential equation (2xy-3x2) dx + (x2-2y) dy = O
(5)
Question 2
[25]
2.1 Suppose that a car is going 76 m/s when brakes are applied at t = 2 s. Suppose that the
nonconstant deceleration is known to be a= -12t 2. Formulate the differential equation
and determine the distance the car travels.
(10)
2.2 Find the particular solution of x' +x = e·1
(10)
2.3 Solve the equation: 5yll +2yl +2y = o.
(5)
Question 3
[25]
3.1 Find the eigenvalues and eigenvector of the matrix A given by
-1
2
-1
3.2 Solve the following system of equations using Gauss-Jordan Elimination:
-3x - 2y + 4z = 9
3y- 2z = 5
4x - 3y+ 2z = 7
3.3
! If [ 2x 3 ] [~ 3] [;] = 0, find the value of x
(10)
(10)
(5)
2
 |
3 Page 3 |
▲back to top |
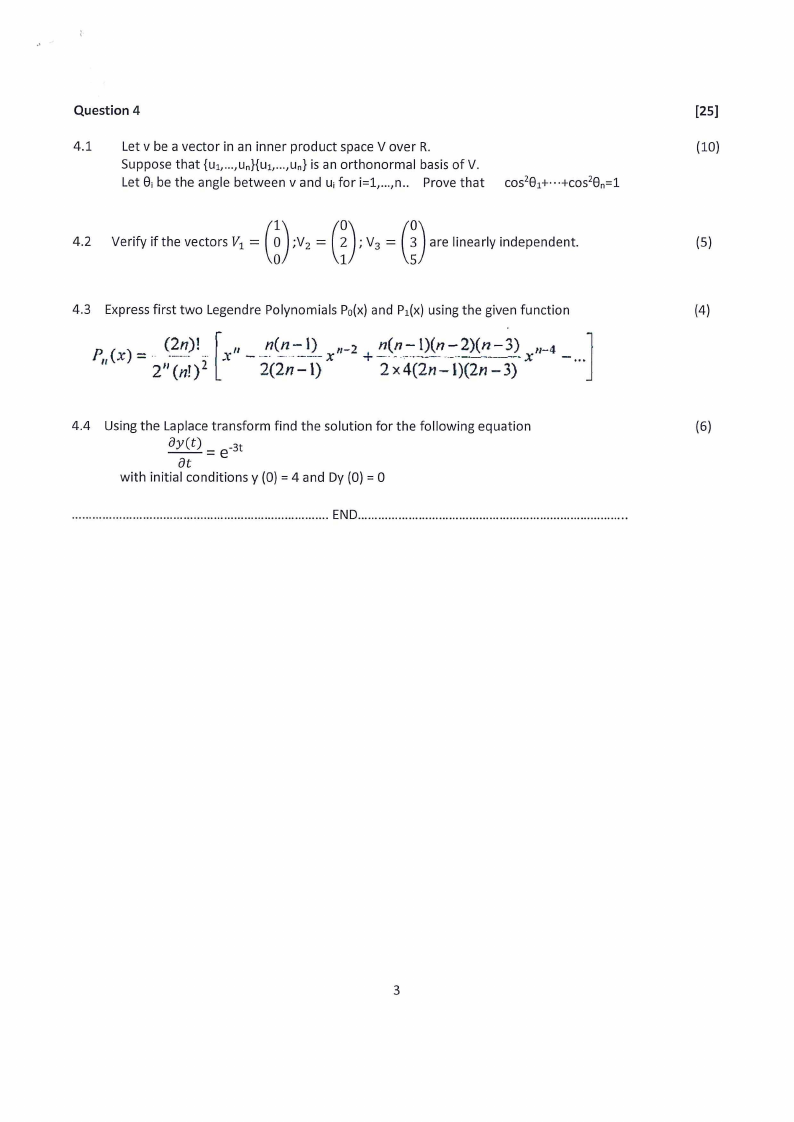
Question 4
[25]
4.1
Let v be a vector in an inner product space V over R.
(10)
Suppose that {U1,...,un}{u1,...,un} is an orthonormal basis of V.
Let 8; be the angle between v and u; for i=1, ...,n.. Prove that cos281+···+cos28n=1
G),G} (i) 4.2 Verify if the vectors V1 =
V2 =
V3 =
are Iinearly independent.
(5)
4.3 Express first two Legendre Polynomials Po(x) and P1(x) using the given function
(4)
4.4 Using the Laplace transform find the solution for the following equation
(6)
ay(t) = e-3t
at
with initial conditions y (O)= 4 and Dy (0) = 0
............................................................................ END...............................................................................
3





